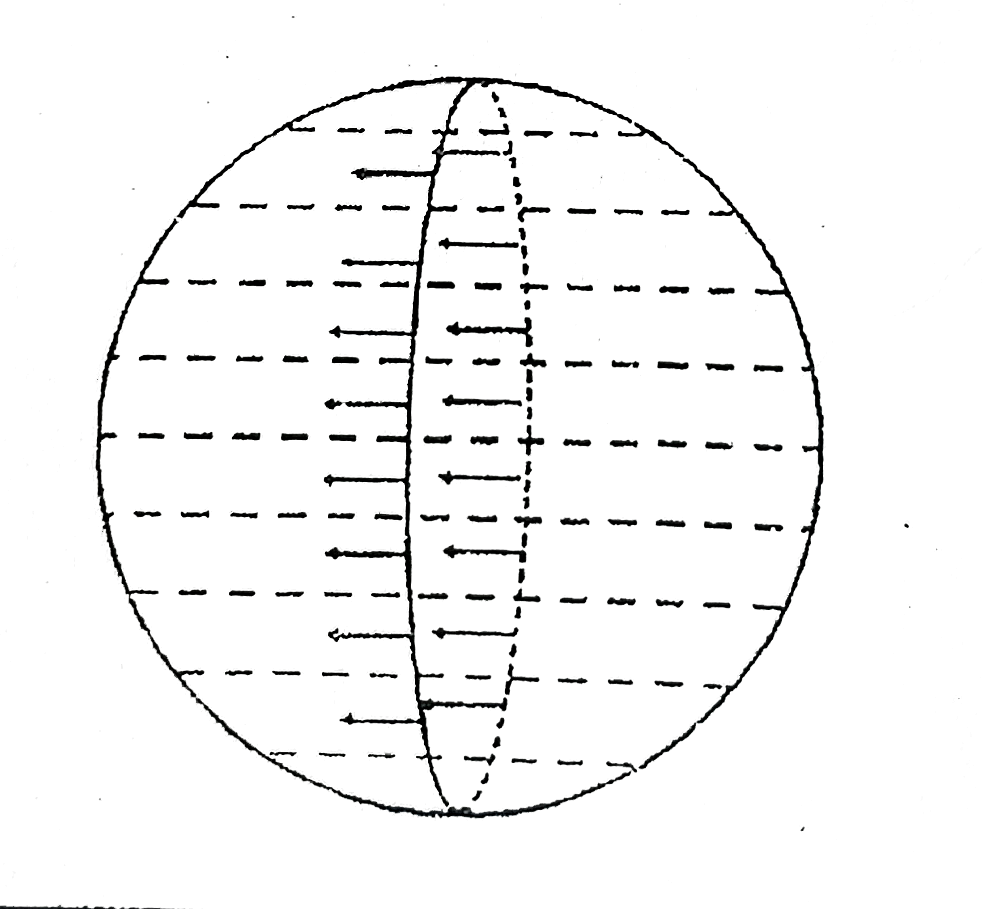
Consider the water drop of radius R. Find surface tension force between the left half surface and right half surface ?
Surface tension force
F=(T)(l)
here l= length of boundary line between left half and right half surface =2πR
So F=(T)(2πR)
Topper's Solved these Questions
- SURFACE TENSIONBOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 1 PART - I
10 Videos
- SURFACE TENSIONBOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 1 PART - II
17 Videos
- STRING WAVESBOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
- UNITS, DIMENSION & MEASUREMENTBOOK - RESONANCECHAPTER - UNITS, DIMENSION & MEASUREMENTEXERCISE - Exercise
27 Videos
Similar Questions
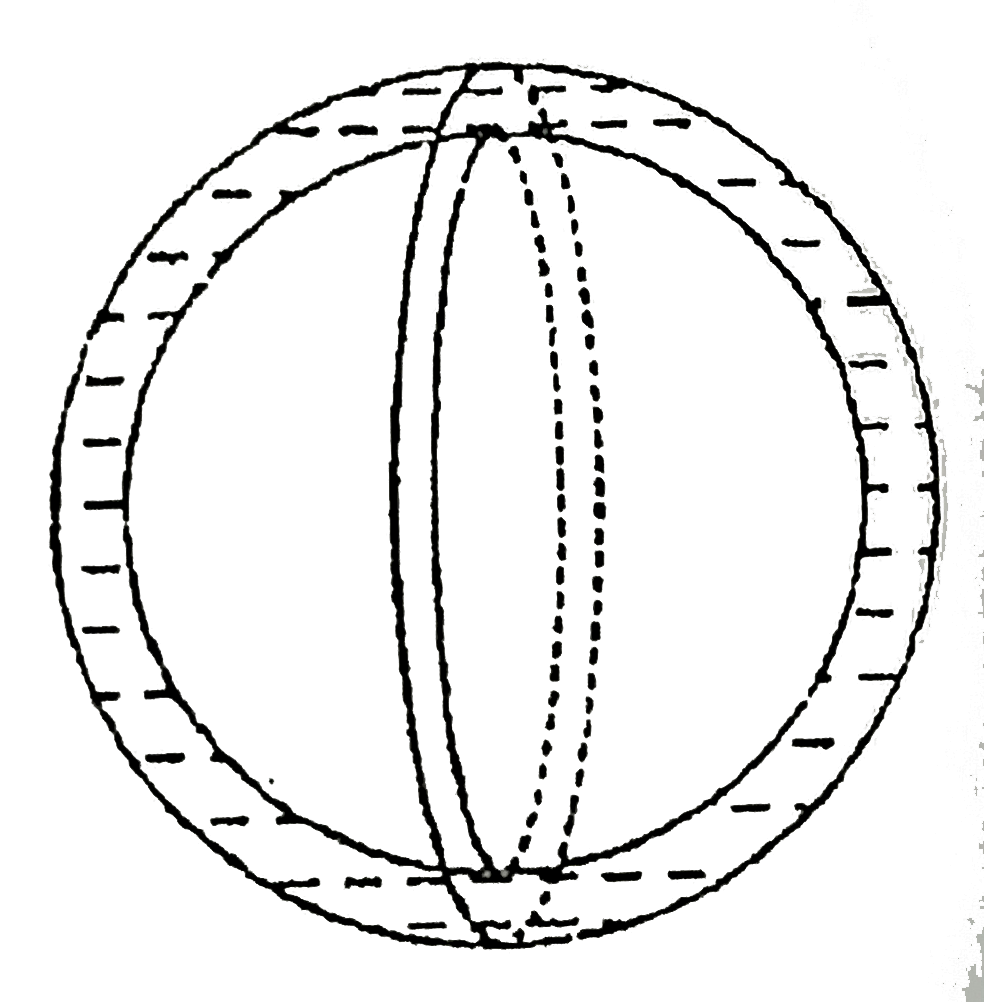
Consider a bubble of soap solution. Find the surface tension force between the left half surface and right half surface
View SolutionFigure shows the container of radius R filled with water. Consider an imaginary diametric line drividing the surface in two parts: Left half and right half. Find surface tansion force between the left half surface and the right half surface.

View Solution
Knowledge Check
The force due to surface tension is
Anormal to free surfaceBnormal to free surface downwardsCalong the free surfaceDat an angle of 60∘ with free surfaceInternal pressure inside a liquid drop of radius r and surface tension T is
A2Tr−P0B4Tr+P0CP0+2TrDT4r−P0The force exerted by surface tension on the free surface
Ais along the surfaceBis perpendicular to the surface directed upwards.Cis perpendicular to the surface directed downwards.Dnone of the above
Surface Tension ||Surface tension Force and Surface Energy
View SolutionSome drops each of radius rcoalesce to form a large drop of radius R. The surface tension T. Find the change in surface energy per unit volume?
View SolutionAn annular disc of radius r1=10cm and r2=5cm is placed on a water surface. Find the surface tension force on the disc if we want to pull it from water surface. Take coefficient of surface tension as σ=7×10−3N/m,g=10ms−12.

View SolutionSurface tension||Soap bubble and drop||Surface energy
View SolutionThe relation between surface tension and surface energy is
View Solution
