Consider the Venturi tube of Figure. Let area A equal 5a. Suppose the pressure at A is 2.0atm. Compute the values of velocity v at 'A' and velocity v at 'a' that would make the pressure p' at 'a' equal to zero. Computer the corresponding volume flow rate it the diameter at A is 5.0cm. (The phenomenon at a when p' falls to nearly zero is known as cavitation. The water vaporizes into small bubbles.)
(Patm=105N/m2,ρ=1000kg/m3).
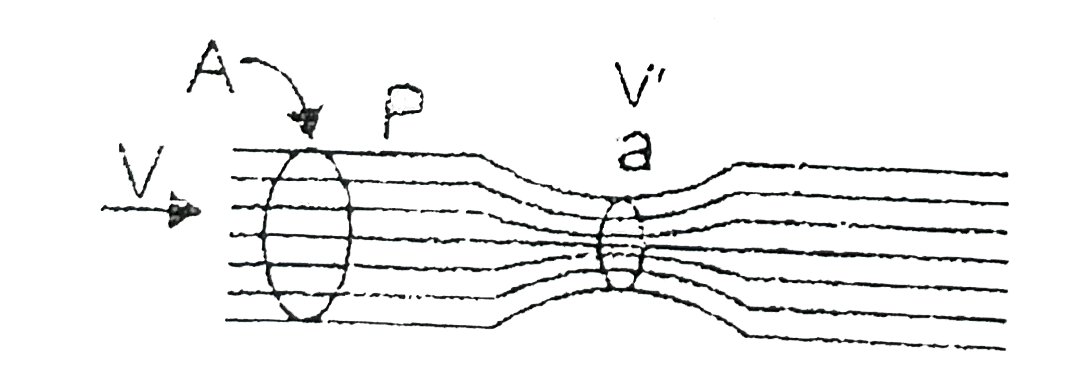
The correct Answer is:v=10√6m/s=4.1m/s;v'=50√6m/s=21m/s;
Av=8.1×10−3m3/sec
Step by step video, text & image solution for Consider the Venturi tube of Figure. Let area A equal 5a. Suppose the pressure at A is 2.0 atm. Compute the values of velocity v at 'A' and velocity v at 'a' that would make the pressure p' at 'a' equal to zero. Computer the corresponding volume flow rate it the diameter at A is 5.0 cm. (The phenomenon at a when p' falls to nearly zero is known as cavitation. The water vaporizes into small bubbles.) (P_(atm) = 10^(5)N//m^(2), rho = 1000 kg//m^(3)). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FLUID MECHANICSBOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - II Section (A)
6 Videos
- FLUID MECHANICSBOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - II Section (B)
8 Videos
- FLUID MECHANICSBOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (B)
3 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise
52 Videos
- FULL TEST 1BOOK - RESONANCECHAPTER - FULL TEST 1EXERCISE - Exercise
30 Videos
Similar Questions
Consider the venturi tube of Sample Problem 14.21 and Fig.without the manometer. Let A equal 5a. Suppose the pressure p1 at A is 3.0 atm. Compute the values of (a) the speed V at A and (b) the speed vat a that make the pressure p2 , at a equal to zero. (c) Compute the corresponding volume flow rate if the diameter at A is 5.0 cm. The phenomenon that occurs at a when p, falls to nearly zero is known as cavitation. The water vaporizes into small bubbles.
View SolutionWater is flowing through a long horizontal tube. Let PAandPB be the pressures at two points A and B of the tube
View Solution
Knowledge Check
The rms velocity of a particle is v at pressure p. If the pressure increases by two times , then the rms velocity will be
A4 vB2 vCvD0.5vThe equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, will be
APV=52RTBPV=516RTCPV=532RTDPV=5RTThe equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, wll be
APV =(5/32) TRBPV=5RTCPV=(5/2) RTDPV= (5/16) RT

In the figure, the pulley P moves to the right with a constant speed v0. If the velocity of B is v in downward direction, find the velocity of A.
View SolutionUnder a constant pressure head, the rate of streamlined volume flow of a liquid through a capillary tube is V. if the length of the capillary tube is double and diameter of the bore is halved, find the rate of flow of the liquid through the capillary tube.
View SolutionWater is flowing streamline motion through a horizontal tube. The pressure at a point in a tube is P where the velocity of flow is v. At another point where the pressure isp2, the velocity of flow is (density of water = ρ)
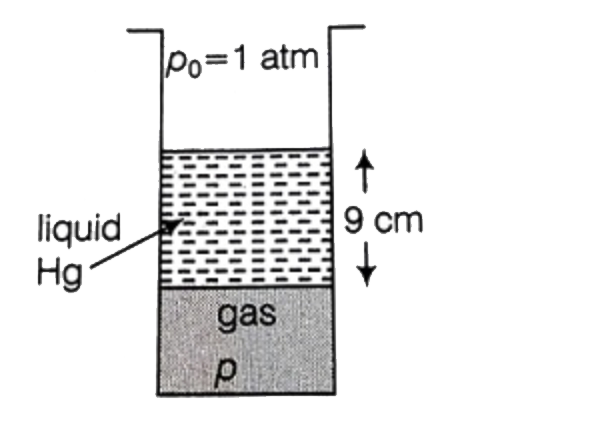
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
View SolutionIfλ=2.5 and volume is equal to 18 times to the initial volume then perssure p' is equal to (initial pressure = p)
View Solution
