If f:R→R is a continuous function satisfying f(0)=1 and f(2x)−f(x)=x∀xεR and limn→∞(f(x)−f(x2n))=P(x). Then P(x) is
A
a constant function
B
a linear function
C
a quadratic polynomial in x
D
a cubic polynomial in x
Video Solution
Text Solution
The correct Answer is:B
Write the functional equation as
f(x)−f(x2)=x2
⇒f(x2)−f(x4)=x4
⇒t(x4)−f(x8)=x8
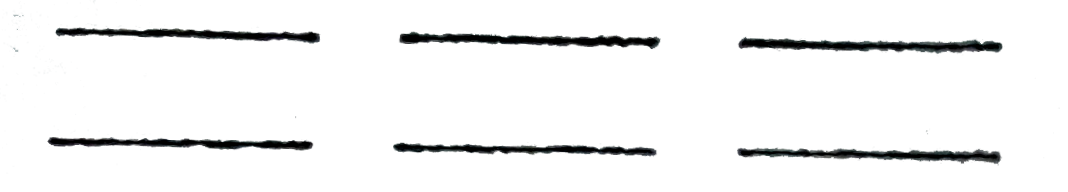
f(x2n−1)−f(x2n)=x2n
Adding up we obtain
f(x)−t(x2n)=x(12+14+−−+12n)
Hence limn→∞(f(x)−f(x2n))=x
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If f:R→R is continuous function satisfying f(0)=1 and f(2x)−f(x)=x,∀x∈R, then limn→∞(f(x)−f(x2n)) is equal to
AxB0C2xDNone of theseIf f:R→R is a continuous function satisfying f(0)=1andf(2x)−f(x)=xfor all x∈Randlimx→∞{f(x)−f(x2n)}=P(x), P(x), is
Aa constant functionBa linear polynomial in xCa quadratic polynomial in xDa cubic polynomial in xIf f(x) is a continuous function satisfying f(x)f(1/x) =f(x)+f(1/x) and f(1) > 0 then limx→1 f(x) is equal to
A2B1C3Dnone of these
Let f be a continuous function satisfying f(x) f(y)=f(x)+f(y)+f(xy)-2 for all x,y ∈ R and f(2)=5 then limx→4f(x) is
View SolutionIf f'(x)=f(x) and f(0)=1 then limx→0f(x)−1x=
View SolutionIf the function f(x) satisfies (lim)x→1f(x)−2x2−1=π,evaluate (lim)x→1f(x)
View SolutionIf f:R→R is a function satisfying the property f(x+1)+f(x+3)=2 for allx∈R than f is
View SolutionLet f be a continuous function satisfying f(x+y)=f(x)+f(y) for all x,y ∈ R and f(1)=5 then limx→4 f(x) is equal to
View Solution
