De-Broglie hypothesized that material particles have wave like properties. Figure shows a small particle in a box. The particle simply bounces back and forth at constant speed. As particles also have wave like properties it can be considered to be a wave reflecting back and forth from the ends of the box. The reflections will create a standing wave analogous to standing wave on a string tied at both ends. Since a standing wave confined to a region can have only selected wavelength, momentum of the particle is quantized. We can safely assume that such a particle only has kinetic energy. This energy must also be quantized.

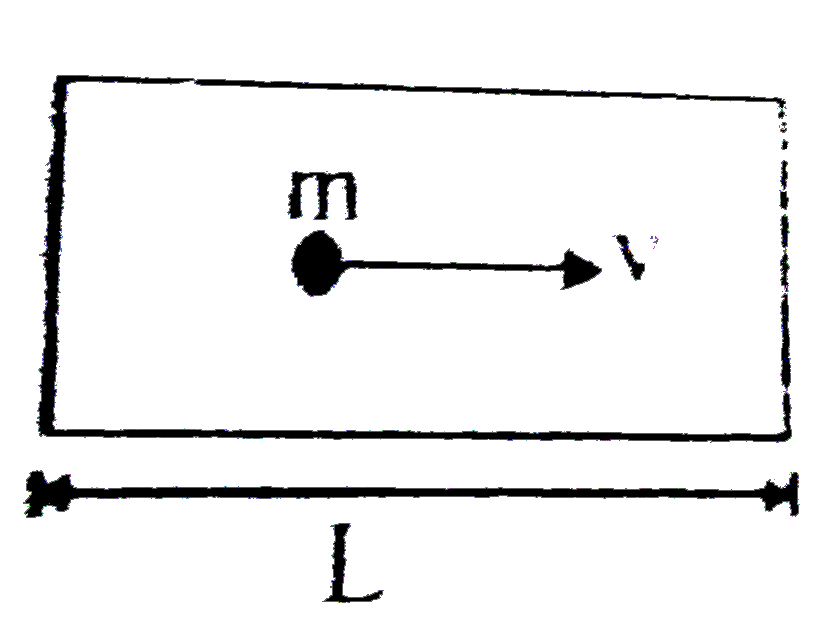
Mark the incorrect option.

confined particle has only centain discrete values of energy.

Particle in box behaves as stading wave.

The particle cannot be at rest.

Matter waves are electromagnetic in nature.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$\frac{1}{λ} = R Z^{2} (\frac{1}{n^{2}} - \frac{1}{m^{2}})$
for H visible is form $2 \to 3, 4, 5, 6$ ……….
here $z = 2$
$\Rightarrow \frac{1}{λ} = R (\frac{Z^{2}}{n^{2}} - \frac{Z^{2}}{m^{2}}) = R (\frac{4}{4^{2}} - \frac{4}{m^{2}}) \frac{4}{m^{2}} = \frac{1}{6^{2}}$
$m = 6 \to 4, 7 \to 4$ ,…….

Updated on:21/07/2023

Class 12 PHYSICS MASTER PRACTICE PROBLEM

Topper's Solved these Questions

Knowledge Check

De-Broglie hypothesized that material particles have wave like properties. Figure shows a small particle in a box. The particle simply bounces back and forth at constant speed. As particles also have wave like properties it can be considered to be a wave reflecting back and forth from the ends of the box. The reflections will create a standing wave analogous to standing wave on a string tied at both ends. Since a standing wave confined to a region can have only selected wavelength, momentum of the particle is quantized. We can safely assume that such a particle only has kinetic energy. This energy must also be quantized.

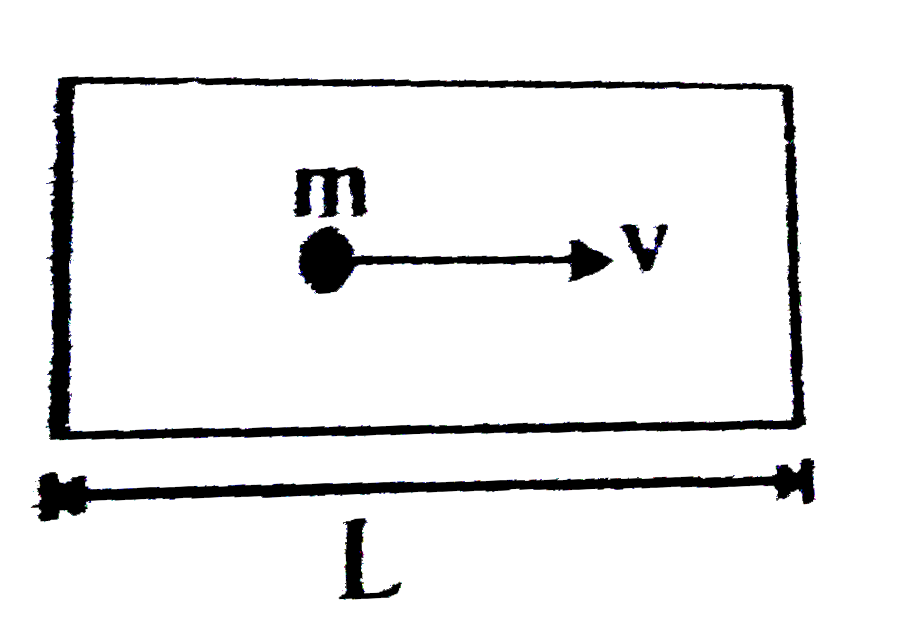
What is momentum of particle in n^(th) mode of standing wave ?
A $\frac{n h}{2 L}$
B $\frac{n h}{L}$
C $\frac{2 n h}{L}$
D $\frac{2 Ln}{h}$
De-Broglie hypothesized that material particles have wave like properties. Figure shows a small particle in a box. The particle simply bounces back and forth at constant speed. As particles also have wave like properties it can be considered to be a wave reflecting back and forth from the ends of the box. The reflections will create a standing wave analogous to standing wave on a string tied at both ends. Since a standing wave confined to a region can have only selected wavelength, momentum of the particle is quantized. We can safely assume that such a particle only has kinetic energy. This energy must also be quantized.

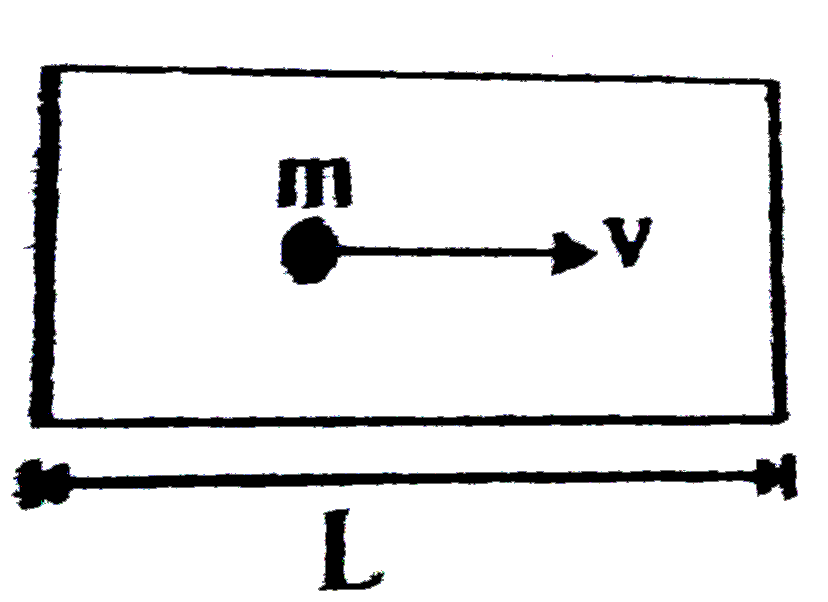
What is the particle's energy in n^(th) state?
A $\frac{h^{2} n^{2}}{8 m L^{2}}$
B $\frac{h^{2} n^{2}}{4 m L^{2}}$
C $\frac{h^{2} n^{2}}{2 m L^{2}}$
D $\frac{4 h^{2} n^{2}}{m L^{2}}$
De-Broglie hypothesized that material particles have wave like properties. Figure shows a small particle in a box. The particle simply bounces back and forth at constant speed. As particles also have wave like properties it can be considered to be a wave reflecting back and forth from the ends of the box. The reflections will create a standing wave analogous to standing wave on a string tied at both ends. Since a standing wave confined to a region can have only selected wavelength, momentum of the particle is quantized. We can safely assume that such a particle only has kinetic energy. This energy must also be quantized.

Bohr model is applied to signly ionized helium $H e^{+}$ Consider the lines that lie in the visible region of the spectrum. Which of the following transitions can given a photon of visible light?
A $n = 5$ to $n = 4$
B $n = 7$ to $n = 4$
C $n = 4$ to $n = 3$
D $n = 8$ to $n = 3$

BANSAL-MASTER PRACTICE PROBLEM-Comphrehension

Home
Ask Doubt
Profile

The correct Answer is:D

1 λ = R Z 2 ( 1 n 2 - 1 m 2 ) for H visible is form 2 → 3 , 4 , 5 , 6 ………. here z = 2 ⇒ 1 λ = R ( Z 2 n 2 - Z 2 m 2 ) = R ( 4 4 2 - 4 m 2 ) 4 m 2 = 1 6 2 m = 6 → 4 , 7 → 4 ,…….

Topper's Solved these Questions

Similar Questions

Knowledge Check

BANSAL-MASTER PRACTICE PROBLEM-Comphrehension