Can a square prism also be a cube?
To determine whether a square prism can also be a cube, we need to understand the definitions of both shapes and their properties.
1. Define a Cube:
A cube is a three-dimensional shape where all six faces are squares, and all edges are of equal length. This means that in a cube, the length, width, and height are all the same.
Hint: Remember that a cube has equal dimensions in all directions.
2. Define a Square Prism:
A square prism (also known as a rectangular prism when the bases are rectangles) is a three-dimensional shape that has two square bases and rectangular sides. The sides can be of different lengths, but the bases must be squares.
Hint: A square prism has square bases, but the height can vary.
3. Relationship Between Cube and Square Prism:
Since a cube has all its dimensions equal, it fits the definition of a square prism because it has two square bases (the top and bottom faces) and rectangular sides (which are also squares in the case of a cube).
Hint: Think about how a cube can be seen as a special case of a square prism.
4. Conclusion:
Therefore, while all cubes are square prisms (because they meet the criteria of having square bases), not all square prisms are cubes. A square prism can have different lengths for its height compared to its base dimensions, which would disqualify it from being a cube.
Hint: Consider the dimensions: if they are not all the same, it cannot be a cube.
Final Answer:
Yes, a square prism can also be a cube, but only if all dimensions are equal. Otherwise, a square prism is not a cube.
A cube is a three-dimensional shape where all six faces are squares, and all edges are of equal length. This means that in a cube, the length, width, and height are all the same.
A square prism (also known as a rectangular prism when the bases are rectangles) is a three-dimensional shape that has two square bases and rectangular sides. The sides can be of different lengths, but the bases must be squares.
Since a cube has all its dimensions equal, it fits the definition of a square prism because it has two square bases (the top and bottom faces) and rectangular sides (which are also squares in the case of a cube).
Therefore, while all cubes are square prisms (because they meet the criteria of having square bases), not all square prisms are cubes. A square prism can have different lengths for its height compared to its base dimensions, which would disqualify it from being a cube.
Yes, a square prism can also be a cube, but only if all dimensions are equal. Otherwise, a square prism is not a cube.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A regular square pyramid is placed in a cube so that the base of the pyramid and that of the cube coincide. The vertex of the pyramid lies on the face of the cube opposite to the base, as shown. An edge of the cube is 7 inches.
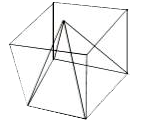
How many square inches (approximately) are in the posi- tive difference between the surface area of the cube and the surface area of the pyramid ?A134.4B133.4C138.4D135.4Which of the following is a perfect square as well as a cube?
343, 125, 81, or 64A81B125C343D64Six square faces join to make a cube.
AJoining of six square faces is done to make a cube.BMaking of cube is done by joining six square faces.CFaces which are square are joined by to make a cube.DA cube is made by joining six square faces.
Square prism is also called a _______.
View SolutionRectangular prism is also called a ________.
View SolutionShow that the quadrilateral, formed by joining the mid-points of the sides of a square, is also a square.
View SolutionGive reasons for the following:
(a) A square can be thought of as a special rectangle.
(b) A square can be thought of as a special rhombus.
( c) A rectangle can be thought of as a special parallelogram.
(d) A square is also a parallelogram.
View SolutionCalculation of square root and cube root
View Solution
