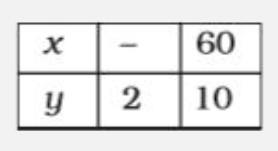
If x and y vary inversely as each other, and x=10 when y=6. Find y when x=15
To solve the problem step by step, we will use the concept of inverse variation.
1. Understand the relationship: Since x and y vary inversely, we can express this relationship as:
x⋅y=k
where k is a constant.
2. Find the constant k: We are given that x=10 when y=6. We can substitute these values into the equation to find k:
k=10⋅6=60
3. Set up the equation for the new values: Now we need to find y when x=15. Using the relationship we established, we can set up the equation:
15⋅y=60
4. Solve for y: To find y, we can rearrange the equation:
y=6015
Simplifying this gives:
y=4
5. Conclusion: Therefore, when x=15, the value of y is 4.
Final Answer:
When x=15, y=4.
x⋅y=k
where k is a constant.
k=10⋅6=60
15⋅y=60
y=6015
Simplifying this gives:
y=4
When x=15, y=4.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If y=f(x)andx=g(y) are inverse functions of each other, then
Ag''(y)=1f''(x)Bg''(y)=f''(x)(f''(x))3Cg''(y)=f'(x)(f'(x))3DNone of these
If x and y vary inversely and x=18 when y=8, find x when y=16.
View SolutionIf x and y vary inversely and x=15 when y=6, find y when x=9.
View SolutionIf x varies inversely as y and x = 20 when y = 600, find y when x = 400
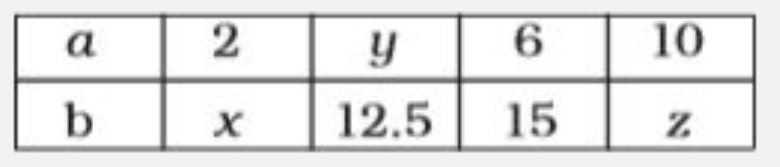
View SolutionIf a and b vary inversely to each other, then find the values of x, y, z.
View SolutionIf x varies inversely as y and y = 60 when x = 1.5. Find x. when y = 4.5.
View SolutionIf two quantities x and y vary directly with each other, then
View SolutionIf x varies as yz, then y varies inversely as
View Solution