What is the median of the frequency distribution shown in the table?
Data ValueFrequency 24 3 25 7 26 5 27 1
To find the median of the given frequency distribution, we will follow these steps:
Step 1: Create a Cumulative Frequency Table
We start by creating a cumulative frequency table from the given data.
| Data Value | Frequency | Cumulative Frequency |
|------------|-----------|----------------------|
| 24 | 3 | 3 |
| 25 | 7 | 3 + 7 = 10 |
| 26 | 5 | 10 + 5 = 15 |
| 27 | 1 | 15 + 1 = 16 |
Step 2: Calculate Total Frequency
Next, we calculate the total frequency, which is the last value in the cumulative frequency column.
Total Frequency = 16
Step 3: Determine the Median Position
The median position can be found using the formula:
Median Position=N2
where N is the total frequency.
Median Position = 162=8
Step 4: Identify the Median Class
Now, we need to find the class interval that contains the 8th position in the cumulative frequency table.
From the cumulative frequency table:
- The cumulative frequency for 24 is 3 (positions 1 to 3)
- The cumulative frequency for 25 is 10 (positions 4 to 10)
Since the 8th position falls between 4 and 10, the median class is 25.
Step 5: Conclusion
Thus, the median value for the given frequency distribution is 25.
We start by creating a cumulative frequency table from the given data.
|------------|-----------|----------------------|
| 24 | 3 | 3 |
| 25 | 7 | 3 + 7 = 10 |
| 26 | 5 | 10 + 5 = 15 |
| 27 | 1 | 15 + 1 = 16 |
Next, we calculate the total frequency, which is the last value in the cumulative frequency column.
The median position can be found using the formula:
Median Position=N2
where N is the total frequency.
Now, we need to find the class interval that contains the 8th position in the cumulative frequency table.
- The cumulative frequency for 24 is 3 (positions 1 to 3)
- The cumulative frequency for 25 is 10 (positions 4 to 10)
Thus, the median value for the given frequency distribution is 25.
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - STATISTICSEXERCISE - EXERCISES8videos
STATISTICS
- BOOK - ENGLISH SATCHAPTER - SOLVING POLYNOMIAL INEQUALITIES EXERCISE - EXERCISES3videos
SOLVING POLYNOMIAL INEQUALITIES
- BOOK - ENGLISH SATCHAPTER - STRATEGIES FOR SOLVING SAT MATH PROBLEMS EXERCISE - EXAMPLE19videos
STRATEGIES FOR SOLVING SAT MATH PROBLEMS
Similar Questions
If the median of the following frequency distribution is 32.5 . Find the value of p :
 View Solution
View SolutionThe mean of the following frequency distribution is 2117. Find the value of f
 View Solution
View SolutionFrom the frequency distribution table from the following data
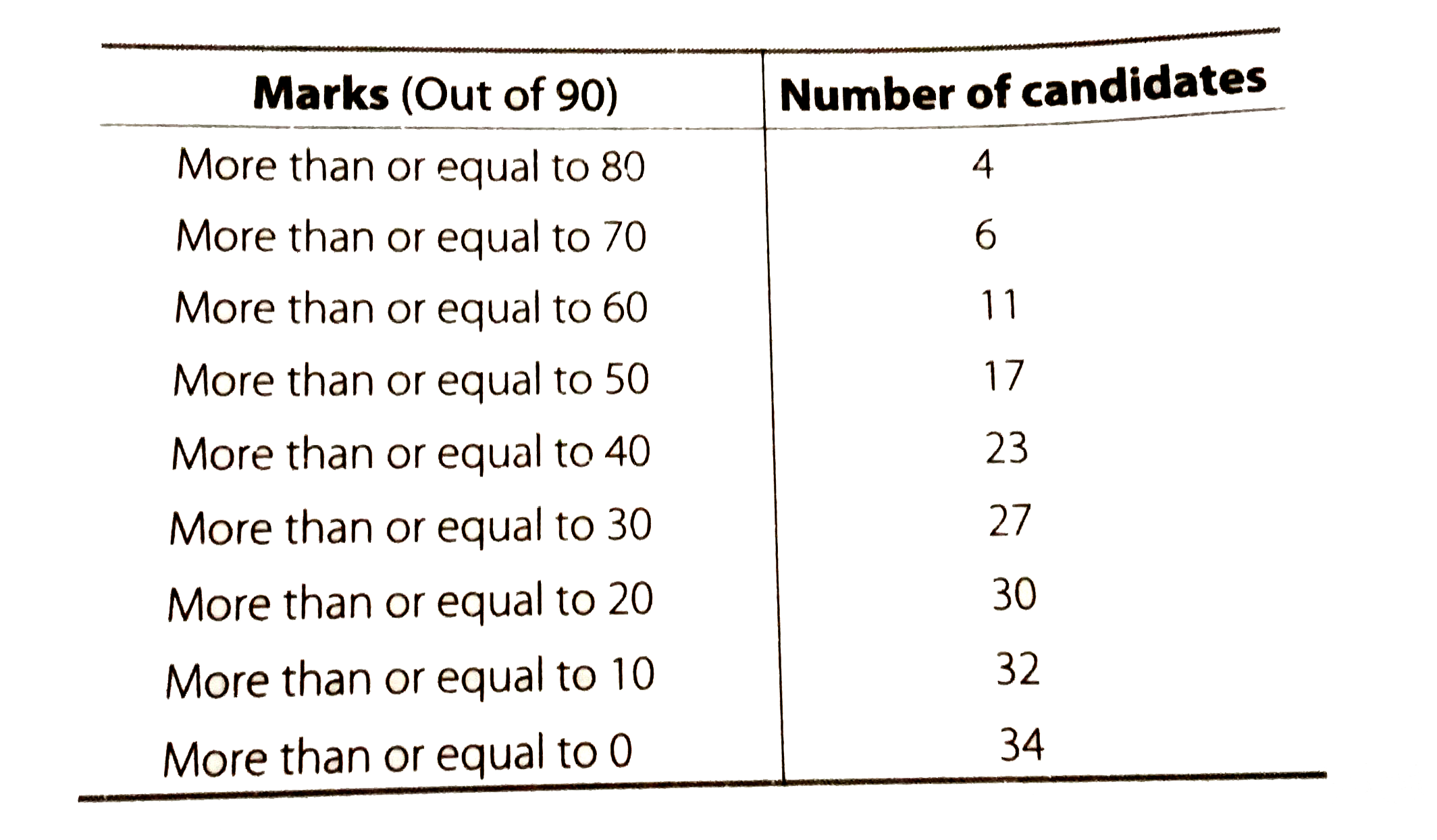 View Solution
View SolutionIf the median of the following distribution is 28, find the values of x and y.If the total frequency is 50.
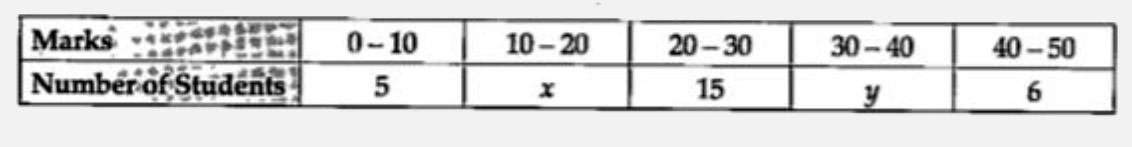 View Solution
View SolutionIf the mean of the following distribution is 7.5, find the missing frequency f
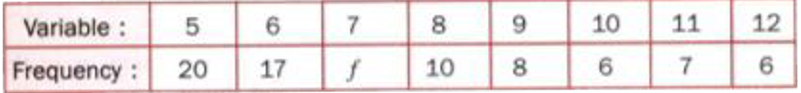 View Solution
View SolutionIf the mean of the following frequency distribution is 5, then b =
xi :3574fi :2a5bView SolutionFind the mean of the following frequency distributions: Classinterval: 0−8 8−16 16−24 24−32 32−40 Frequency: 5 6 4 3 2
View SolutionIf the mean of the distribution is 2.6, then the value of y is
Variate x12345Frequency f of x45y12View SolutionIf the mean of the following distribution is 2.6 Then the value of p is
Variable x: 1 2 3 4 5
frequency of x: 4 5 P 1 2View SolutionFind the missing frequency if the median for the given distribution is 24:
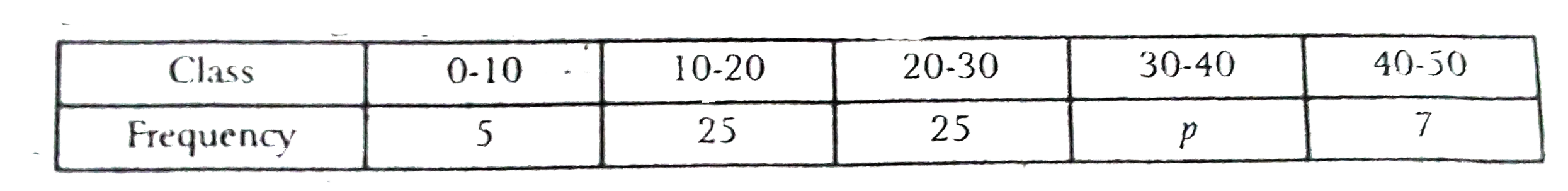 View Solution
View Solution

