Which of the following is a transformation of y=f(x) that translates this function down 3, shrinks it horizontally by a factor of 2, and reflects it about the x-axis?

A

y=−2f(x−3)

B

y=f(−2x)−3

C

y=−f(12x)−3

D

y=−f(2x)−3

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The horizontal shrinking by a factor of 2 of is the multiplication of x by 2 before the function is applied. The reflection about the x-axis is the negation of the function after it is applied. The translation down 3 is the addition of -3 after the function is applied.

Updated on:21/07/2023
|
Class 12MATHSTHREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Which of the following functions transforms y=f(x) moving it 5 units to the right?

    Ay=f(x+5)
    By=f(x-5)
    Cy=f(x)+5
    Dy=f(x)-5

  • For which of the following functions does f(x)f(2−x) ?

    Af(x)=x+2
    Bf(x)=2x−x2
    Cf(x)=2−x
    Df(x)=(2−x)2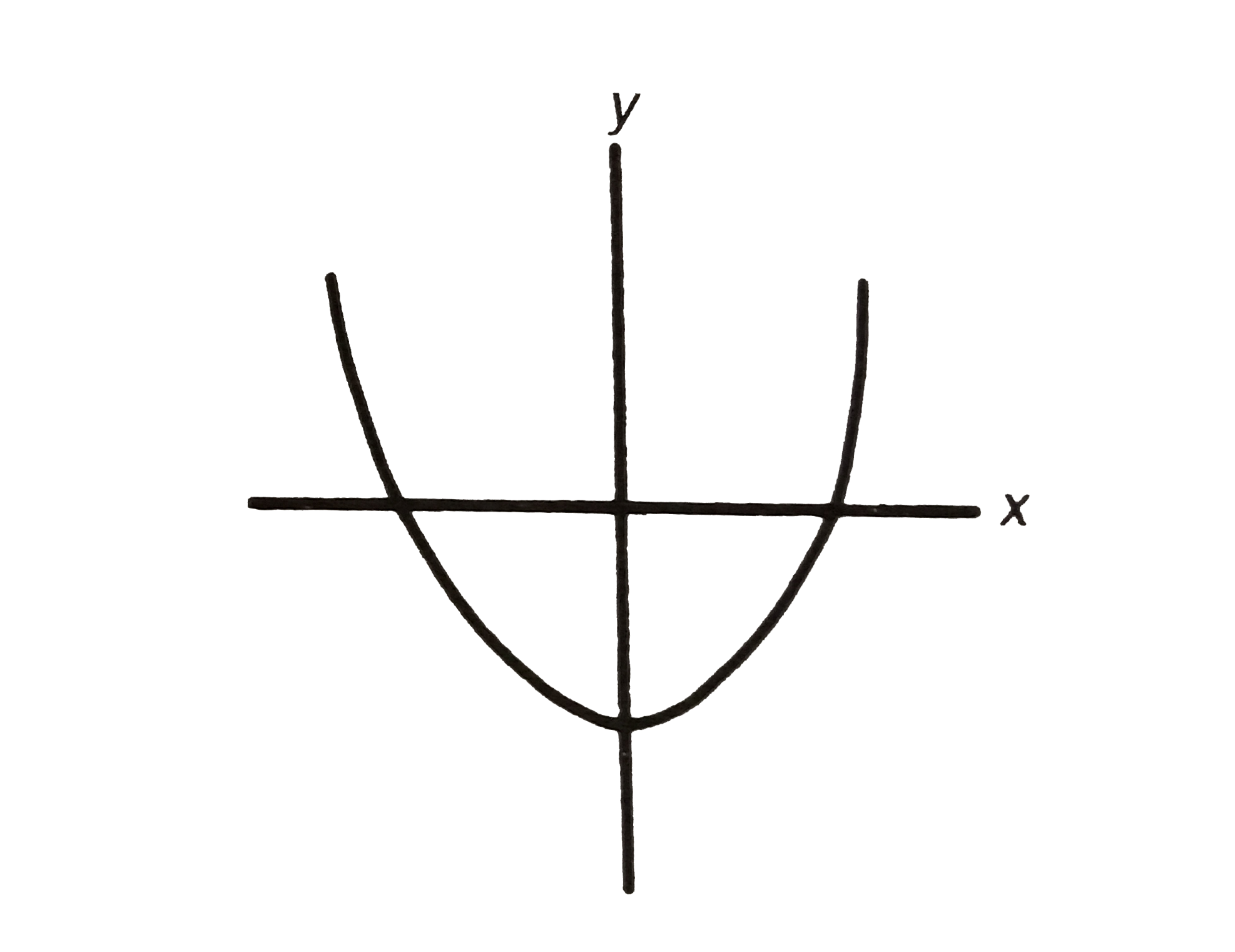

  • ocr_image
    In the figure above, the graph of y=f(x) has two transformations performed on it. First it is rotated 180∘ about the origin, and then it is reflected about the x-axis. Which of the following is the equations of the resulting curve?

    Ay=-f(x)
    By=f(x+2)
    Cx=f(y)
    Dy=f(x)