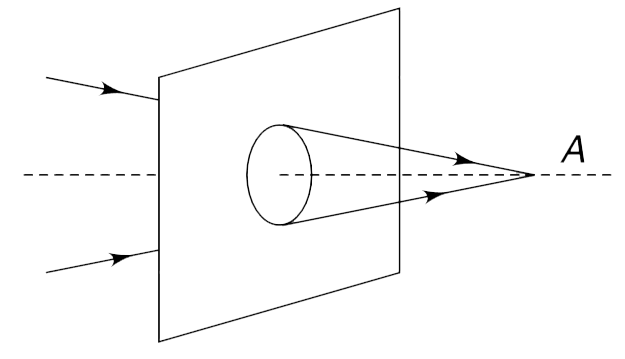
A converging beam of light rays passes through a round opening in a screen. The beam converges at a point A which is at a perpendicular distance of 15 cm from the screen and lies slightly above the central axis of the circular opening. A convex lens of focal length 30 cm is inserted in the opening. At what distance from the screen do the rays converge now ?
The correct Answer is:10cm
Step by step video, text & image solution for A converging beam of light rays passes through a round opening in a screen. The beam converges at a point A which is at a perpendicular distance of 15 cm from the screen and lies slightly above the central axis of the circular opening. A convex lens of focal length 30 cm is inserted in the opening. At what distance from the screen do the rays converge now ? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:ARIHANTChapter:GEOMETRICAL OPTICSExercise:Level 2
Explore 62 Videos
- GEOMETRICAL OPTICSBook:ARIHANTChapter:GEOMETRICAL OPTICSExercise:Level 3
Explore 9 Videos
- ELECTROSTATICSBook:ARIHANTChapter:ELECTROSTATICSExercise:Level 3
Explore 59 Videos
- MAGNETIC EFFECT OF CURRENTBook:ARIHANTChapter:MAGNETIC EFFECT OF CURRENTExercise:Magnetic effect of Current
Explore 45 Videos
Similar Questions
A beam of light converges at a point P. Now a convex lens of focal length 30 cm placed in the path of the convergent beam 12 cm from P. The point at which the beam converges now is
View SolutionA convergent beam of light passes through a diverging lens of focal length 0.2m and comes to focus at a distance of 0.3m behind the lens. Find the position of the point at which the beam would converge in the absence of the lens.
View Solution
Knowledge Check
A beam of light converges at a point P. Now a concave lens of focal length -16 cm is placed in the path of the convergent beam 12 cm from P The point at which the beam converges now is
A6.86cm right side of the lensB6.86cm left side of the lensC48cm right side of the lensD48cm left side of the lensA converging beam of light is incident on a concave lens. The beam converges behind the lens at 15 cm from its centre, in the absence of the lens. Select the correct alternative
AAfter passing through the lens, beam becomes diverging, what ever may be the focal lengthBIf focal length = 10 cm, the beam diverges awayCIf focal length = 20 cm, the beam diverges awayDFocal length = 30 cm, beam converged at focal point of the lensAn object is kept at a distance of 15 cm from a convex lens of focal length 10 cm. Calculate the image distance and state the characteristics of the image formed.
Aupright and magnifiedBinverted and magnifiedCinverted and diminishedDupright and diminished
A beam of light converges at aoint P. Now a convex lens is placed in the path of the convergent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focal length 10 cm?
View SolutionA beam of light converges at a point P. Now a convex lens is placed in the part of the converngent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focad length 10 cm ?
View SolutionA luminous object is placed at a distance of 40 cm from a converging lens of focal length 25 cm. The image obtained in the screen is
View SolutionAn object O is kept infront of a converging lens of focal length 30 cm behind which there is a plane mirror at 15 cm from the lens.
_E01_080_Q01.png)
View SolutionAn illuminated object is placed at a distance of 20 cm from a converging lens of focal length 15 cm. The image obtained on the screen is:
View Solution
