Question

Trapezium a quadrilateral having exactly one pair of parallel sides is called a trapezium.

Explanation

Area of Trapezium

Understanding the different geometrical shapes is very important. In the previous chapter, we have learned a lot of shapes with examples as well. In this lesson, students will learn about trapezium.  We will also discuss the area of a trapezium, including the area of trapezium formula, properties, and so on. Unless proper examples are seen, it becomes impossible to get the right solutions. So, for this, we will also solve some problems to get a better idea about it. 

A trapezium is a type of quadrilateral that have two parallel sides. The other two sides are non-parallel. According to Euclidean Geometry, a polygon having four sides and four vertices is called a trapezium. The parallel and non-parallel sides of a trapezium are termed as bases and legs, respectively. It is also known by the term, ‘trapezoid’. In certain times, the parallelogram is also referred to as a trapezoid with two parallel sides.

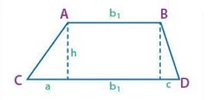
Look at the above figure. We can find that it has got sides AB and CD which are parallel to each other. Note that there are non-parallel sides which are AC and BD. The ‘h’ represents the height of the trapezium, which is the distance between the two parallel sides.

What is the Shape of Trapezium?

When there is a question regarding the shape of a trapezium, different countries have defined it in different ways. Well, there are confusions when there is a distinction made between the terms, “trapezium” and “trapezoid.”  In British, a trapezium is a quadrilateral that has got one pair of parallel sides. On the other hand, a quadrilateral with no parallel sides is termed as ‘trapezoids.’ In the U.S., a quadrilateral with no parallel sides is termed as a ‘trapezium’ while a quadrilateral having one pair of parallel sides is called ‘trapezoids.’

What are the types of trapezium?

Coming to the categorization of trapezium, it has got three different types:

• Scalene Trapezium – This is a type of trapezium that has the sides and angles of different measures


• Isosceles trapezium – This type of trapezium has the legs or the non-parallel sides of the trapezium of equal length


• Right Trapezium – This type of trapezium has at least two right angles.

What are the properties of a trapezium?

Understanding the properties of a trapezium is very important. Below are the important properties of trapezium:

• Exactly one pair of opposite sides are equal in a trapezium


• Except in isosceles trapezium, the non-parallel sides in a trapezium are unequal


• The diagonal intersect each other


• The midpoints of the line of the non-parallel sides are always found to be parallel to the bases.

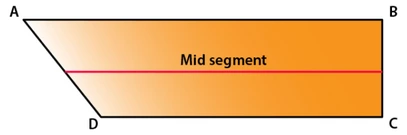
Mid-segment = (AB + CD)/2

Area of a Trapezium

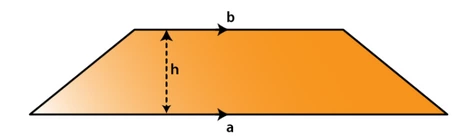
Calculating the area of a trapezium is possible when the two bases of a trapezium are taken and then multiplied by its altitude. Therefore, the area of a trapezium is as under:

Area of a Trapezium, = h(a+b)/2 square units.

Here, the bases are “a” and “b” and “h” is the height or the altitude. So, you have understood the area of a trapezium.

Perimeter of a Trapezium

Coming to the calculation of the area of a trapezium, it can be done by adding all the sides. So, the formula of area of a Trapezium is:

P = a + b + c + d units

Here, the sides of the trapezium are ‘a’, ‘b’, ‘c’ and ‘d.’

 

Related Videos:

• Area of trapezium ABCD


• Area of a rectangle


• Area of a square


• Area of an Equilateral Triangle

Moving on, we will now have a look at some examples as this would be much easier to understand how to attempt the different questions of trapezium and others.

Q.1: The sum of the bases(parallel sides) is 40 cm, and its height is 20 cm. Can you find the area of the trapezium?

Solution: Here,

 (a + b) = 40 cm

Given Height, h = 20 cm

Now applying the formula,

Area of a Trapezium, A = h(a+b)/2 square units.

Now substituting the given values,

A = (40/2)× 20

A = 20 × 20

A = 400 cm².

So, the area of trapezium = 400 cm².

Q.2: The sides of a trapezium is 4 cm, 5 cm, 6 cm and 7 cm. Find the perimeter of a trapezium

Solution: By applying the formula of the perimeter of trapezium, we know;

P = Sum of all the sides

P = 4+5+6+7

P = 22 cm

Hence, the perimeter is 22 cm.

Q.3:   Can you calculate the area of the trapezium where the sum of the bases(parallel sides) is 70 cm and the height is 40 cm?

Solution: Here,

(a + b) = 70 cm

Height, h = 40 cm

We know that,

Area of a Trapezium, A = h(a+b)/2 square units.

Substitute the given values,

A = (70/2) × 40

A = 35 × 40

A = 1400 cm².

So, the area of trapezium = 1400 cm².

Q.4: The sides of a trapezium is 6 cm, 7 cm, 8 cm and 9 cm. Can you find its perimeter?

Solution: By applying the formula of the perimeter of trapezium, we know;

P = Sum of all the sides

P = 6 + 7 + 8 + 9

P = 30 cm

Hence, the perimeter is 30 cm.

Q.5: The length of the parallel sides of a trapezium are in the ratio 3 : 2 and the distance between them is 10 cm. If the area of trapezium is 325 cm², find the length of the parallel sides.



Solution:



Let the common ratio be x,

Then the two parallel sides are 3x, 2x

Distance between them = 10 cm

Area of trapezium = 325 cm²

Area of trapezium = 1/2 (p₁ + p₂) h

So, we have 325 = 1/2 (3x + 2x) 10

Now ⇒ 325 = 5x × 5

            ⇒ 325 = 25x

            ⇒ x = 325/25

Therefore, 3x = 3 × 13 = 39 and 2x = 2 × 13 = 26



Therefore, the length of the parallel sides area are 26 cm and 39 cm. 

Important Chapters:

• NCERT Solutions for Maths


• NCERT Solution for Class 6 Maths Chapter Mensuration


• NCERT Solution for Class 6 Maths Chapter Basic Geometrical Ideas


• NCERT Solution for Class 8 Maths Chapter Mensuration