A particle is projected with a velocity of 30m/s at an angle 60∘ above the horizontal on a slope of inclination 30∘. Find its range, time of flight and angle of hit.
The coordinate system, projection velocity and its component, and acceleration due to gravity and its component are shown in the adjoining figure. Subsituting corresponding values in following equation, we get the time of flight.
T=2uyay→T=2×155√3=2√3s
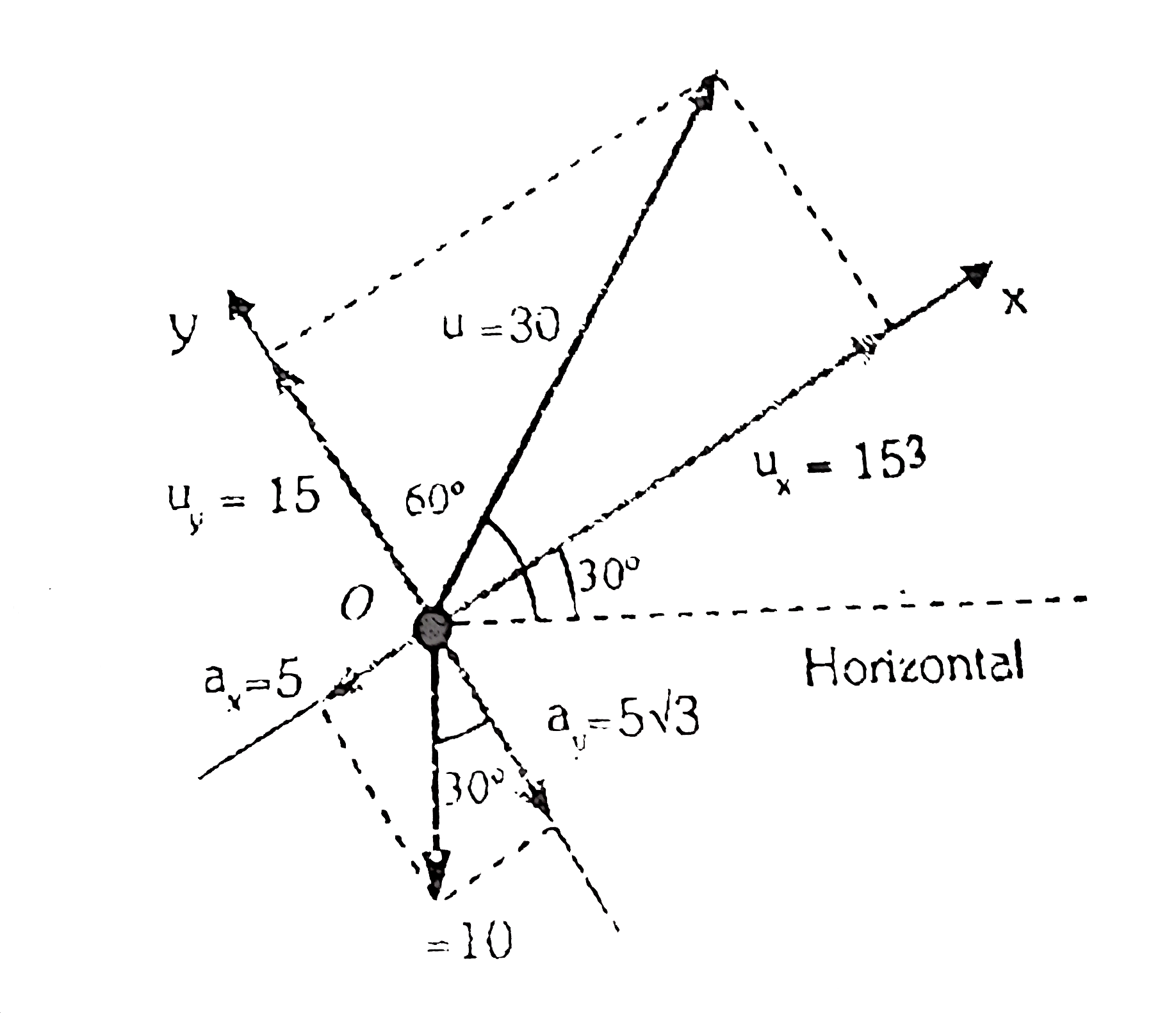
Substituting value of time of flight in following equation, we get the range R.
R=uxT−12axT2⇒R=15√3×2√3−12×5×(2√3)2=60m
In the adjoining figure, components of velocity →vP when the projectile hits the slope at point P are shown. The angle β which velocity vector makes with the x-axis is known as angle of hit. The projectile hits the slope with such a velocity →vP, whose y-component is equal in magnitude to that of velocity of projection. The x-component of velocity vx is calculated by substituting value of time of flight in following equation.
vx=ux−axt→vx=15√3−5×2√3=5√3
β=tan−1(vyvx)→β=60∘

Topper's Solved these Questions
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:EXERCISE-01
Explore 55 Videos
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:EXERCISE-02
Explore 57 Videos
- ERROR AND MEASUREMENTBook:ALLENChapter:ERROR AND MEASUREMENTExercise:Part-2(Exercise-2)(B)
Explore 22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-7
Explore 8 Videos
Similar Questions
Knowledge Check
A particle is projected wityh velocity 20 ms−1 at angle 60∘ above horizontal up on an inclined plane having inclination 30∘. Find its shortest distance from the inclined plane when it is moving 53∘ above horizontal.
(Using =10ms−2)A11.1mB8.2mC3.3mD14.4mA particle is projected with a velocity of 25m/s at an angle of 30∘ with the horizontal . Calculate
(i) The maximum height ,
(ii) time of flight and
(iii) horizontal range .A562 mB286 mC188 mD1130 mA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1m
A particle is projected with a velocity of 25m/s at an angle of 30∘ with the horizontal . Calculate
(i) The maximum height ,
(ii) time of flight and
(iii) horizontal range .
View SolutionIf a particle is projected with a velocity 49m/sec. making an angle 60∘ with the horizontal, its time of flight is given by
View SolutionA particle is projected with a velocity of 20 m/s at an angle of 30∘ to an inclined plane of inclination 30∘ to the horizontal. The particle hits the inclined plane at an angle 30∘, during its journey. The time of flight is -
View SolutionA particle is thrown at time t=0 with a velocity of 10 m/s at an angle of 60∘ with the horizontal from a point on an incline plane, making an angle of 30∘ with the horizontal. The time when the velocity of the projectile becomes parallel to the incline is :
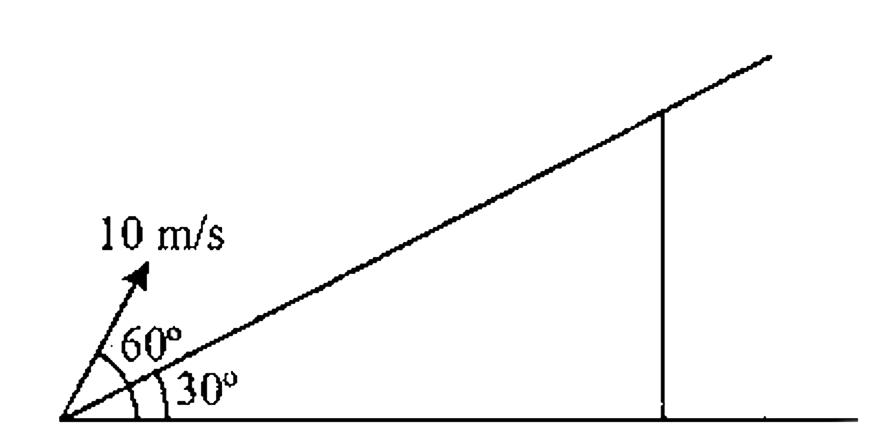
View SolutionTwo particle are projected with same initial velocities at an angle 30∘ and 60∘ with the horizontal .Then
View Solution