
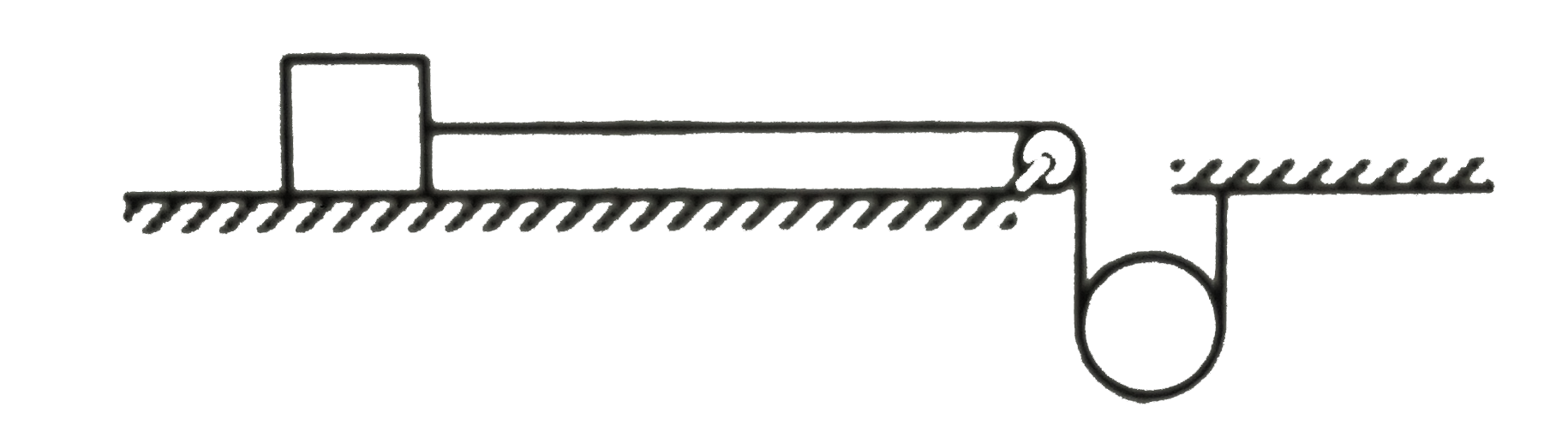
In the arrangement shown, the double pulley has a mass M and the two mass less threads have been tightly wound on the inner (radius ) and outer circumference (radius ). The block shown has a mass 4 M. The moment of inertia of the double pulley system about a horizontal axis passing through its centre and perpendicular to the plane of the figure is .
(a) Find the acceleration of the center of the pulley after the system is released.
(b) Two seconds after the start of the motion the string holding the block breaks. How long after this the pulley will stop ascending?
The correct Answer is:(a)
(b)
Step by step video, text & image solution for In the arrangement shown, the double pulley has a mass M and the two mass less threads have been tightly wound on the inner (radius = r) and outer circumference (radius R = 2r). The block shown has a mass 4 M. The moment of inertia of the double pulley system about a horizontal axis passing through its centre and perpendicular to the plane of the figure is I = (Mr^(2))/(2). (a) Find the acceleration of the center of the pulley after the system is released. (b) Two seconds after the start of the motion the string holding the block breaks. How long after this the pulley will stop ascending? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Let →u be the initial velocity of a particle and →F be the resultant force acting on it . Describe the path that the particle can take if
(a) →u×→F=0and→F=constant
(b) →u.→F=0and→F=constant
In which case can the particle retrace its path .
View SolutionTwo particles of mass m1 and m2 are connected by a spring of natural length L and force constant k. The masses are brought close enough so as to compress the spring completely and a string is used to tie the system. Assume that length of spring in this position is close to zero. The system is projected with a velocity V0 along the positive x direction. At the instant it reaches origin at time t = 0, the string snaps and the spring starts opening.
(a) Show that the mass m1(orm2) will are perform SHM in the reference frame attached to the centre of mass of the system. Find the time period of oscillation.
(b) Write the amplitude of m1 and m2 as a function of time. lt( c) Write the X co ordinates of m1 and m2 as a function of time

View SolutionThe descending pulley shown in figure has a radius 20 cm and moment of inertia 0.20 kg-m^2. The fixed pulley is light and the horizontal plane frictionless. Find the acceleration of the block if its mass is 1.0 kg.
View SolutionCalculate the moments of inertia of the figures shown, each having mass M , radius R and having uniform mass distribution about an axis perpendicular to the plane and passing through the centre?
View SolutionIn the arrangement shown in figure a weight A possesses mass m, a pulley B possesses mass M. Also known are the moment of inertia I of the pulley relative to its axis and the radii of the pulley R and 2R . The mass of the threads is negligible. Find the acceleration of the weight A after the system is set free.
View SolutionA uniform semicircular disc of mass 'm' and radius 'R' is shown in the figure. Find out its moment of inertia about. (a) axis 'AB' ( shown in the figure 0 which passes through geometrical centre and lies in the plane of the disc (b) axis 'CD' which passes through its centre of mass and it is perpendicular to the plane of the disc.
View SolutionA uniform disc of mass m and radius r and a point mass m are arranged as shown in the figure. The acceleration of point mass is :( Assume there is no slipping between pulley and thread and the disc can rotate smoothly about a fixed horizontal axis passing through its centre and perpendicular to its plane )

View SolutionA thin semi circular cylindrical shell has mass M and radius R. Find its moment of inertia about a line passing through its centre of mass parallel to the axis (shown in figure) of the cylinder.

View Solutionदो द्रव्यमान तथा M एक घिरनी पर से होकर गुजर रही एक रस्सी से जुड़ हुए है (चित्र 18.E4)। द्रव्यमान m चिकनी क्षैतिज टेबुल पर फिसलता है। घिरनी की त्रिज्या r है तथा अक्ष के परितः इसक जड़त्व आघूर्ण I है। यह अपने अक्ष के परितः मुक्त रूप से घूर्णन कर सकती है। यह मानते हुए कि घिरनी पर रस्सी फिसलती नहीं है, द्रव्यमान M का त्वरण ज्ञात करें

View SolutionFour similar point masses (m each) are symmetrically placed on the circumference of a disc of mass M and radius R. Moment of inertia of the system about an axis passing through centre O and perpendicular to the plane of the disc will be
View Solutionद्रव्यमान M तथा त्रिज्या R की एक वृत्तीय चकती का चकती के किनारे से गुजरने वाली तथा चकती के तल के लम्बवत् अक्ष के परितः जड़त्व आघूर्ण होता है :
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
View SolutionCalculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionThe moment of inertia of a circular disc of mass m and radius r about an perpendicular axis passing through its centre is
View Solution
