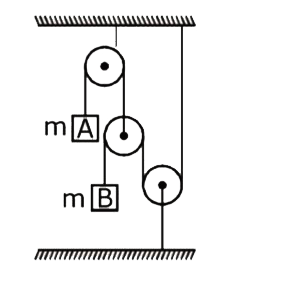
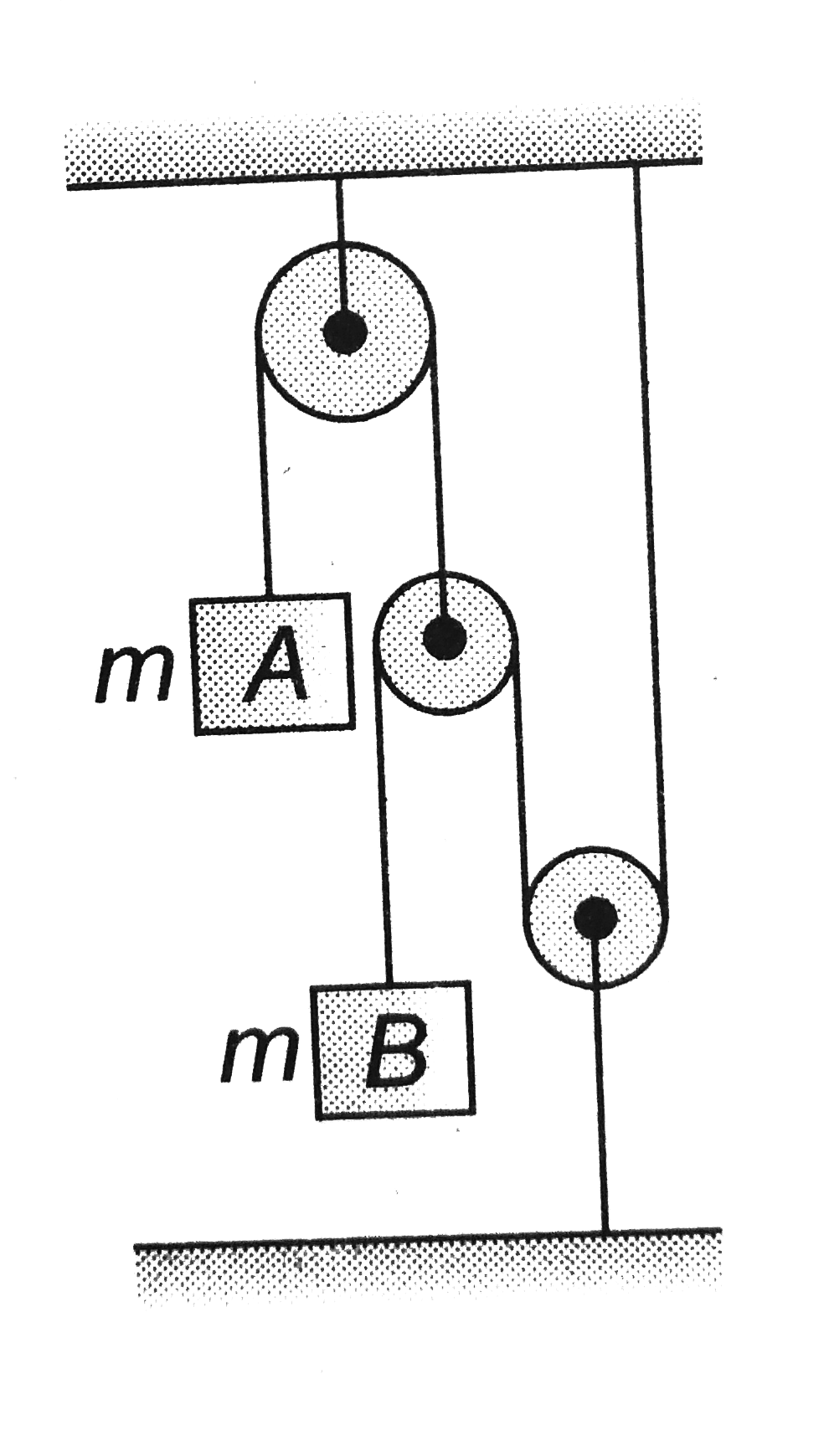
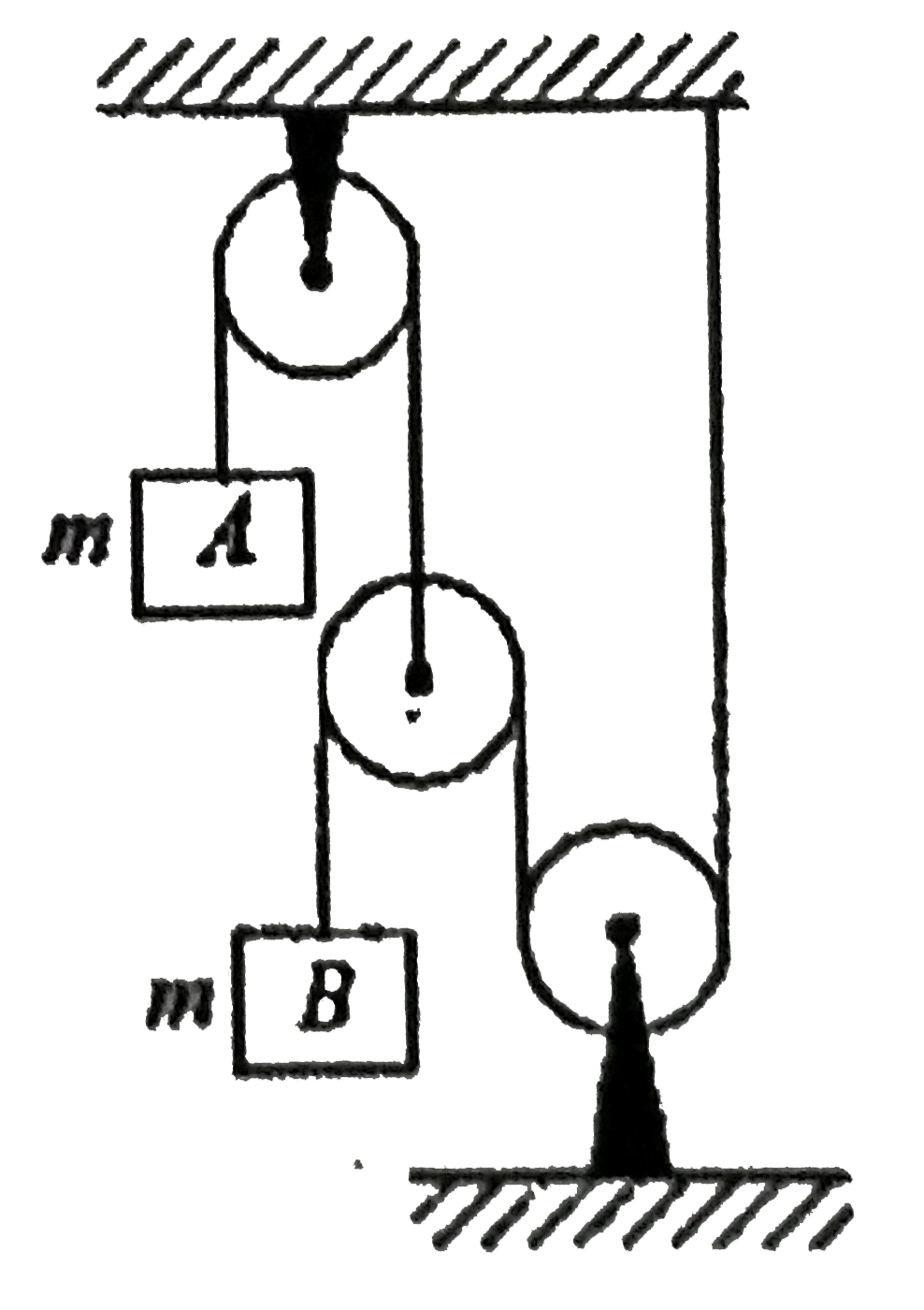
In the arrangement shown in the figure the system is in equilibrium. Mass of the block A is M and that of the insect clinging to block B is m. Pulley and string are light. The insect loses contact with the block B and begins to fall. After how much time the insect and the block B will have a separation L between them.
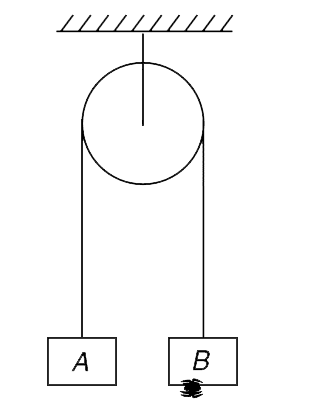
The correct Answer is:t=√2(M−m)LMg
Step by step video, text & image solution for In the arrangement shown in the figure the system is in equilibrium. Mass of the block A is M and that of the insect clinging to block B is m. Pulley and string are light. The insect loses contact with the block B and begins to fall. After how much time the insect and the block B will have a separation L between them. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
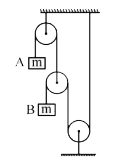
In arrangement shown the block A of mass 15 supported in equilibrium by the block B. Mass of the block B is closest to
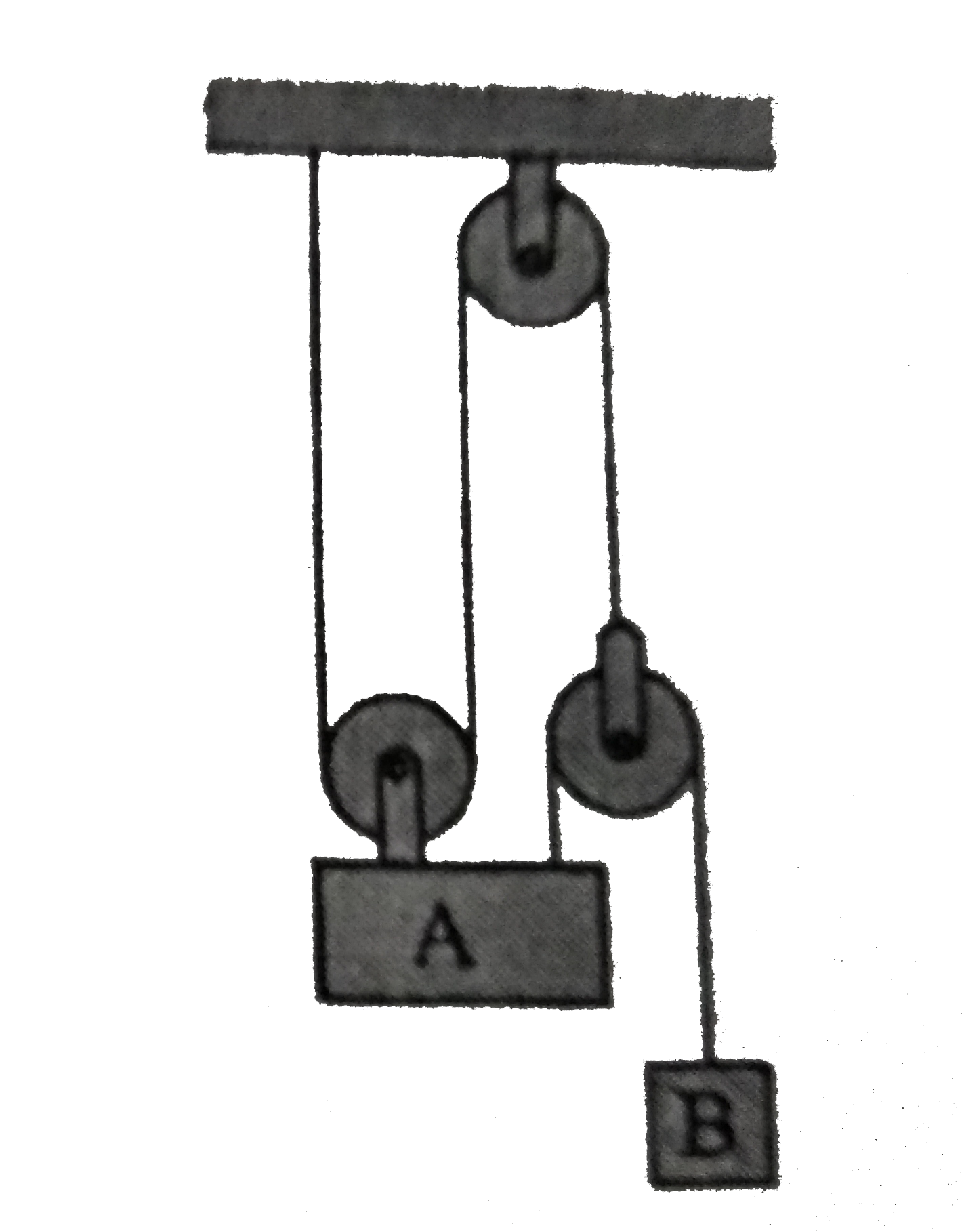 A2 kgB3 kgC4 kgD5 kg
A2 kgB3 kgC4 kgD5 kgIn the arrangement shown, the pulleys and the string are ideal. The acceleration of block B is.
 Ag/5Bg/2C2g/5D2g/3
Ag/5Bg/2C2g/5D2g/3In the arrangement shown, the pulleys and strings are massless. The acceleration of block B is :
 Ag/2B5g/3C2g/3D2g/5
Ag/2B5g/3C2g/3D2g/5
In the arrangement shown in fig neglect the masses of pulleys and string and also friction Calculate acceleration of blocks A and B

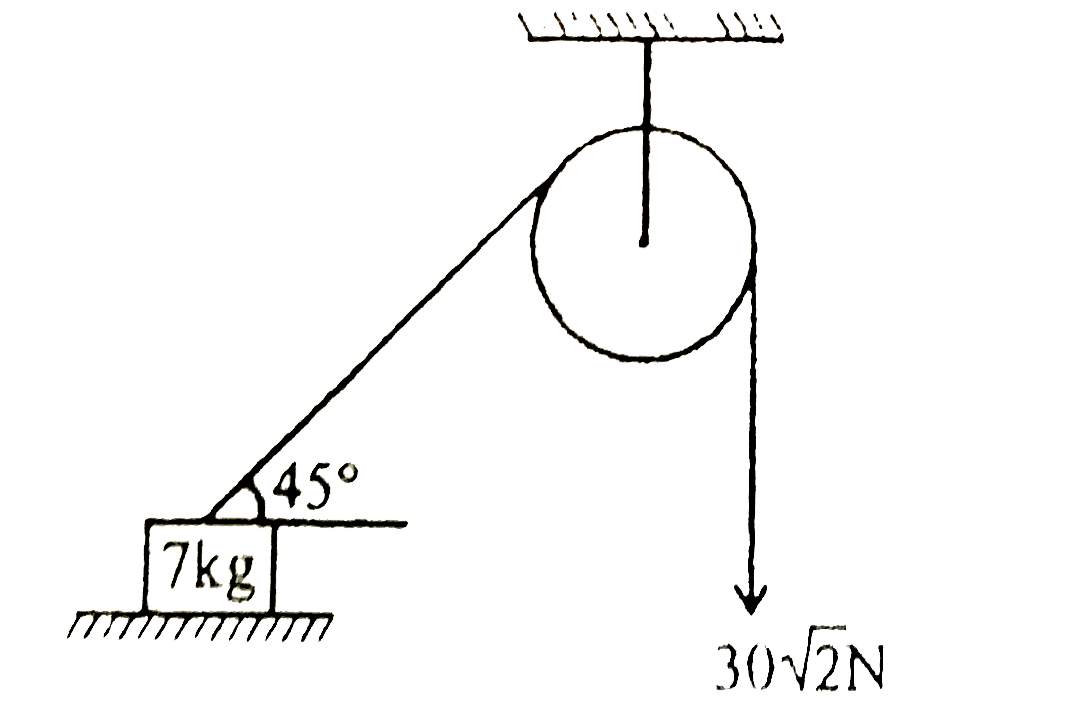
View SolutionIn the arrangements shown, the pulley is smooth and the rope is inextensible. If the system is in equilibrium, the contact force between ground and block is
View SolutionIn the arrangement shown, the pulleys are smooth and the strings are inextensible. The acceleration of block B is :
View SolutionIn the arrangement shown in the figure, mass of the block B and A is 2m and m respectively. Surface between B and floor is smooth. The block B is connected to the block C by means of a string-pulley system. If the whole system is released, then find the minimum value of mass of block C so that A remains stationary w.e.t. B. Coefficient of friction between A and B is μ.

View SolutionIn the arrangement shown in figure, there is a friction force between the blocks of masses m and 2m. The mass of the suspended block is m. The block of mass m is stationary with respect to block of mass 2m. The minimum value of coefficient of friction between m and 2m is
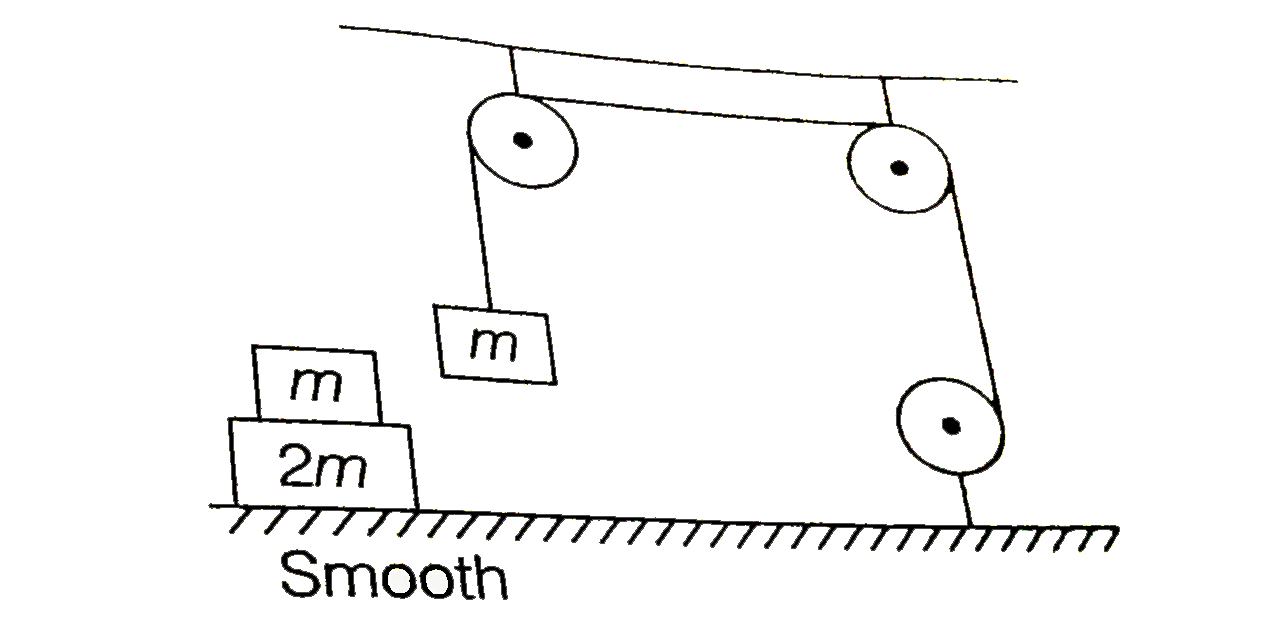
View Solution