A sugar exist in two isomeric form α−D & β−D with specific rotation of 110∘ and 30∘ respectively. When α−D form of the suar is dissolved in water exhibits mutarotation till the equilibrium value of 90∘ is attained. The equilibrium constant of mutarotation of β−D form [Assuming only α and β form in solution] is equal to:
The correct Answer is:00003.33
α−D→x%,∴90∘=(x)(110)+(1−x)30∘
β−D→(1−x)
90∘=(110x−30x)+30∘
x=6080=68
α−D1−x⇔β−Dx
Keq=x1−x
∴K′eq for β−D⇔α−D is 1Keq=1−xx
∴=K′eq=2/86/8=26=13=3.33
Topper's Solved these Questions
- STOICHIOMETRY AND BALANCING REDOX REACTION Book:FIITJEEChapter:STOICHIOMETRY AND BALANCING REDOX REACTION Exercise:SINGLE INTEGER ANSWER TYPE QUESTIONS
Explore 5 Videos
- THERMODYNAMICS AND THERMOCHEMISTRY Book:FIITJEEChapter:THERMODYNAMICS AND THERMOCHEMISTRY Exercise:SINGLE INTEGER ANSWER TYPE QUESTIONS
Explore 5 Videos
Similar Questions
The specific optical rotations of pure a- and b-D-mannopyranose are + 29.3° and -17.0, respectively. When either form is dissolved in water, specific optical rotation of the equilibrium mixture is found to be + 14.2°. Calculate the percentage of a anomer at equilibrium.
View SolutionWhen α−D glucose is dissolved in water, it undergoes a partial converion to β−D glucose to exhibit mutarotation. This conversion stops when 63.6% of glucose is in β form. Assuming that equilibrium has been attained, calculate Kc for mutarotation.
View Solution
Knowledge Check
The specific rotation of α - and β -forms of a monosaccharide are +29∘ and −17∘ respectively. When either form is dissolved in water the specific rotation of the equilibrium mixture was found to be +14∘
Aα -form = 67.4%, β -form = 32.6%Bα-form = 60%, β -form = 40%Cα -form = 70 %, β - form = 30%Dα - form = 50%, β-form = 50%α -D-glucose and β-D-glucose have a specific rotation of +112∘ and +19∘ respectively. In aqueous solution the rotation becomes +52∘. This is known as
AracemisationBmutarotationCinversionDenolisationThe equilibrium constant for mutarotation α−D Glucose ⇔β−D Glucose 1.8. What percentage of α form remains at equilibrium?
A35.7B64.3C55.6D44.4
When either form of D-Glucose is dissolved In water, the solution gradually changes its optical rotation and finally attains a constant optical rotation of +52∘.
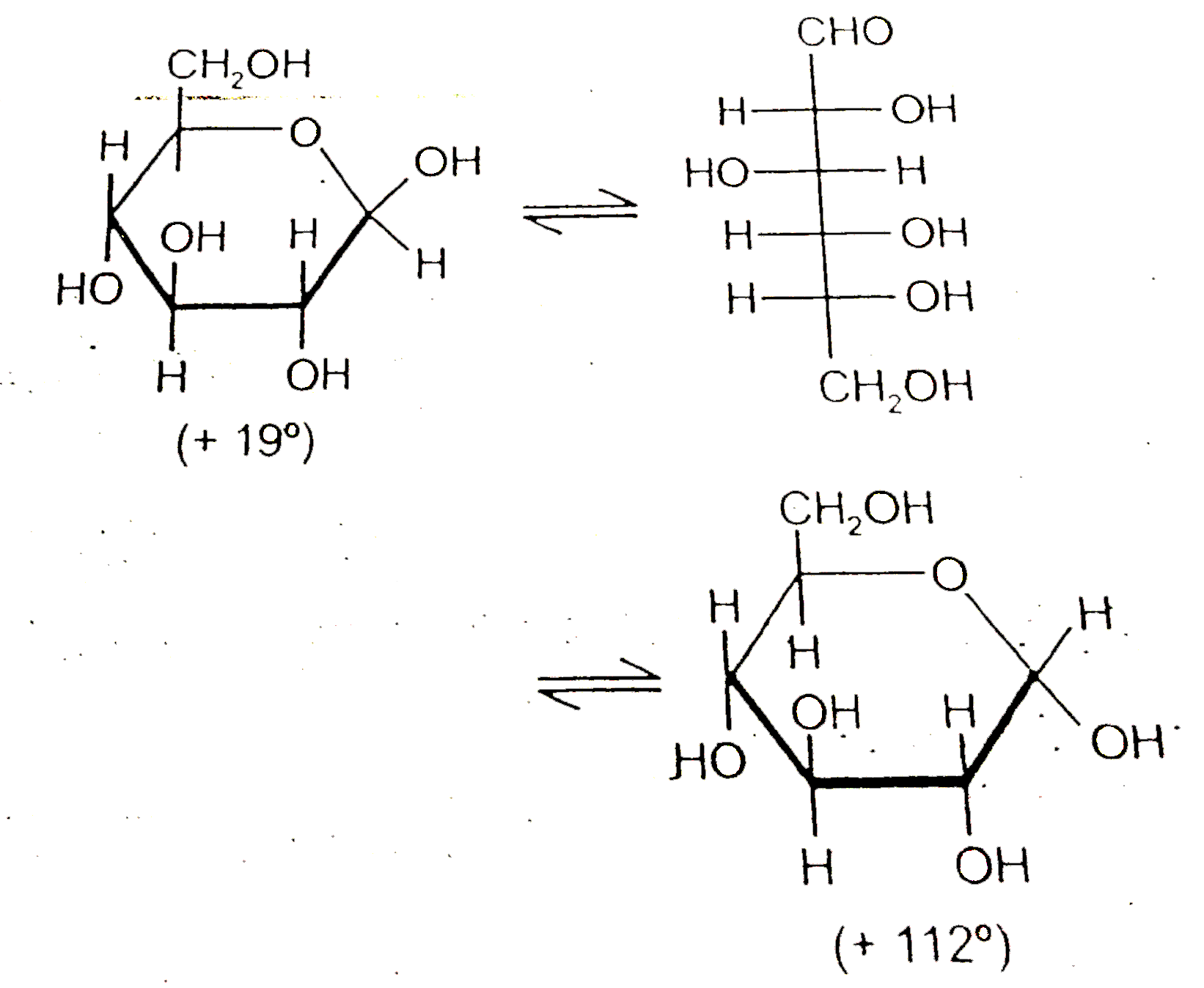
Which form of D-GIucose is more stable?
View SolutionWhen α−D glucose is dissolved in water, it undergoes a partial converion to β−D glucose to exhibit mutarotation. This conversion stops when 63.6% of glucose is in β form. Assuming that equilibrium has been attained, calculate Kc for mutarotation.
View SolutionWhen either form of D-Glucose is dissolved In water, the solution gradually changes its optical rotation and finally attains a constant optical rotation of +52∘.

What is the percentage of open chain D-Glucose in solution?
View SolutionThe beta (β) and alpha (α) glucose have different specific rotation. When either is dissolved in water, their specific rotation changed to reach a certain fixed value. This is called :-
View SolutionThe beta (β) and alpha (α) glucose have different specific rotation. When either is dissolved in water, their specific rotation changed to reach a certain fixed value. This is called :-
View Solution