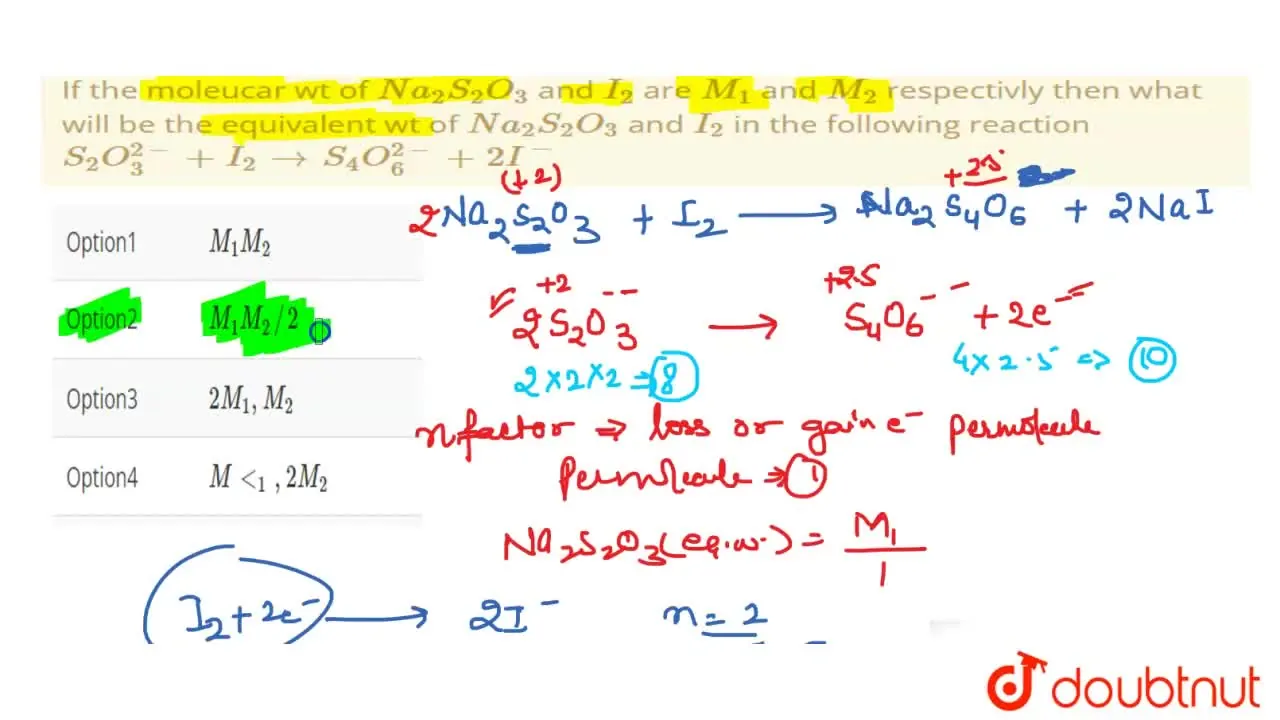
If the moleucar wt of $N a_{2} S_{2} O_{3}$ and $I_{2}$ are $M_{1}$ and $M_{2}$ respectivly then what will be the equivalent wt of $N a_{2} S_{2} O_{3}$ and $I_{2}$ in the following reaction
$S_{2} O_{3}^{2 -} + I_{2} \to S_{4} O_{6}^{2 -} + 2 I^{-}$

A

$M_{1} M_{2}$

B

$M_{1} M_{2} / 2$

C

$2 M_{1}, M_{2}$

D

$M <_{1}, 2 M_{2}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:b

To find the equivalent weight of $N a 2 S 2 O 3$ and $I 2$ in the given reaction, we will follow these steps:
Step 1: Write the balanced reaction
The reaction given is:
\( \text{S}_2\text{O}_3^{2-} + \text{I}_2 \rightarrow \text{S}_4\text{O}_6^{2-} + 2\text{I}^- \)
Step 2: Determine the oxidation states
1. For $S 2 O 3^{2 -}$ , the oxidation state of sulfur can be calculated. Let the oxidation state of sulfur be \( x \):
\(
2x + 3(-2) = -2 \implies 2x - 6 = -2 \implies 2x = 4 \implies x = +2
\)
Thus, the oxidation state of sulfur in $S 2 O 3^{2 -}$ is +2.
2. In $S 4 O 6^{2 -}$ , let the oxidation state of sulfur be \( y \):
\(
4y + 6(-2) = -2 \implies 4y - 12 = -2 \implies 4y = 10 \implies y = +2.5
\)
Thus, the oxidation state of sulfur in $S 4 O 6^{2 -}$ is +2.5.
Step 3: Calculate the change in oxidation state
- The change in oxidation state for sulfur from $S 2 O 3^{2 -}$ to $S 4 O 6^{2 -}$ is:
\(
\Delta \text{Oxidation State} = +2.5 - (+2) = +0.5
\)
- Since there are 2 sulfur atoms in $S 2 O 3^{2 -}$ , the total change is:
\(
2 \times 0.5 = 1
\)
Step 4: Determine the n-factor for $N a 2 S 2 O 3$
The n-factor for $N a 2 S 2 O 3$ is the total change in oxidation state per molecule, which is 1.
Step 5: Determine the n-factor for $I 2$
1. In the reaction, $I 2$ is reduced to $2 I^{-}$ , which means:
- Each iodine atom goes from 0 in $I 2$ to -1 in $I^{-}$ .
- The total change for $I 2$ is:
\(
2 \text{ (for 2 electrons)}
\)
Thus, the n-factor for $I 2$ is 2.
Step 6: Calculate the equivalent weights
1. The equivalent weight of $N a 2 S 2 O 3$ :
\(
\text{Equivalent weight of } Na2S2O3 = \frac{M_1}{n_1} = \frac{M_1}{1} = M_1
\)
2. The equivalent weight of $I 2$ :
\(
\text{Equivalent weight of } I2 = \frac{M_2}{n_2} = \frac{M_2}{2}
\)
Step 7: Summary of equivalent weights
- The equivalent weight of $N a 2 S 2 O 3$ is \( M_1 \).
- The equivalent weight of $I 2$ is \( \frac{M_2}{2} \).
Final Answer
Thus, the equivalent weights of $N a 2 S 2 O 3$ and $I 2$ in the reaction are:
- Equivalent weight of $N a 2 S 2 O 3$ : \( M_1 \)
- Equivalent weight of $I 2$ : \( \frac{M_2}{2} \)

Updated on:7/8/2024

|

Class 11 CHEMISTRY REDOX REACTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

If the molecular mass of $N a_{2} S_{2} O_{3}$ and $I_{2}$ are $M_{1} and M_{2}$ respectively, then what will be the equivalent mass of $N a_{2} S_{2} O_{3}$ and $I_{2}$ in the following reaction
$2 S_{2} O_{3}^{2 -} + I_{2} \to S_{2} O_{6}^{2 -} + I$
A $M_{1}, M_{2}$
B $M_{1}, M_{2} / 2$
C $2 M_{1}, M_{2}$
D $M_{1}, 2 M_{2}$
In the rection
$2 S_{2} O_{3}^{2 -} + I_{2} \to S_{4} O_{6}^{2 -} + 2 I^{-}$
A $S_{2} O_{3}^{2 -}$ gets oxidised to $S_{4} O_{6}^{2 -}$
B $S_{2} O_{3}^{2 -}$ gets oxidised to $S_{4} O_{6}^{2 -}$
C $I_{2} gets reduced to l^{-}$
D $l_{2} gets oxidised to l^{-}$
Consider the redox reaction
$2 S_{2} O_{3}^{2 -} + I_{2} \to S_{4} O_{6}^{2 -} + 2 I^{ө}$
A $S_{2} O_{3}^{2 -} + I_{2} \to S_{45} O_{6}^{2} + 2 I^{-}$
B $S_{2} O_{3}^{2 -}$ gets reduces to $S_{4} O_{6}^{2 -}$
C $S_{2} O_{3}^{2 -}$ gets oxidised to $S_{4} O_{6}^{2 -}$
D $I_{2}$ gets reduced to $I^{-}$

PRADEEP-REDOX REACTIONS -Multiple Choice Question

Home
Ask Doubt
Profile