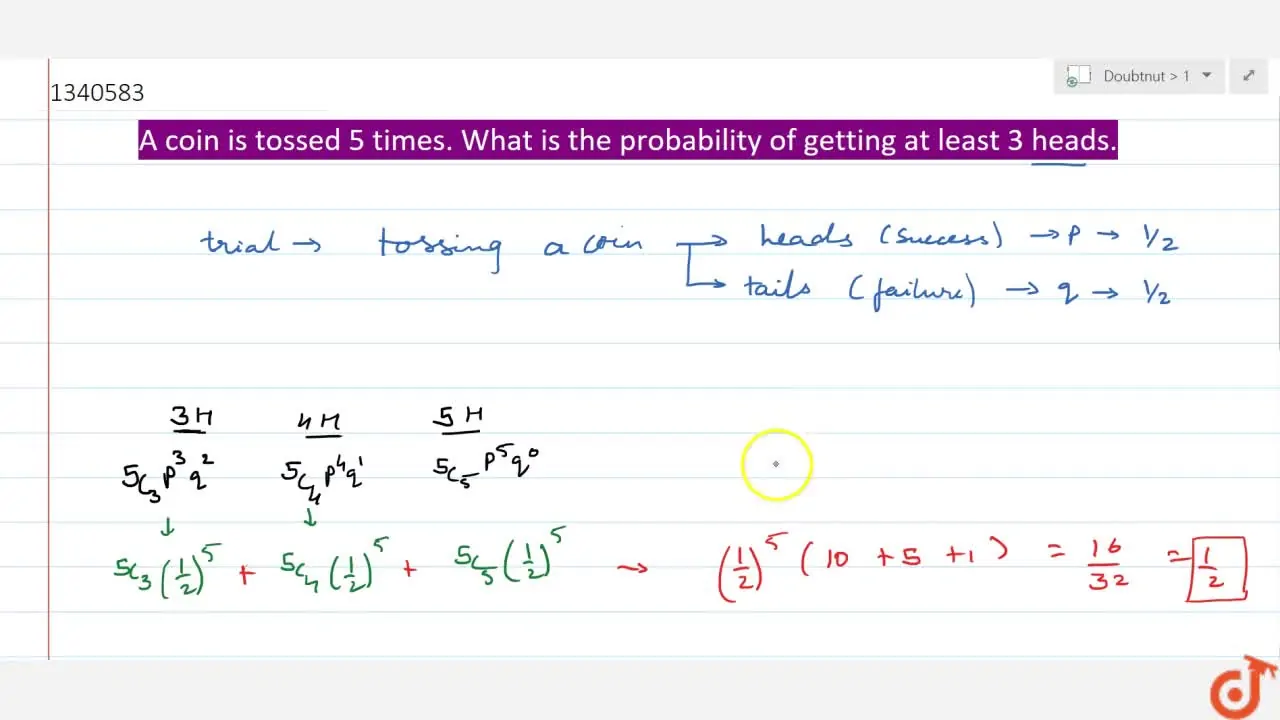
A coin is tossed 5 times. What is the probability of getting at least 3 heads.
Let X denote the number of heads in 5 tosses.
X follows a binomial distribution with {n}=5;p=probability of getting a head =12andq=1−p=12
P(X=r)=5Cr(12)r(12)5−r,r=0,1,2...5
The required probability =P(getting at least 3 heads )
=P(X≥3)
=P(X=3)+P(X=4)+P(X=5)
=5C3(12)3(12)5−3+5C4(12)1(12)5−1+5C5(12)5(12)5−0
=10(12)5+5(12)5+1(12)5
=(12)5(10+5+1)
=(12)5×16
=12
Related Playlists
Similar Questions
(i) A coin is tossed 5 times. What is the probability of getting :
(a) at least 3 heads (b) at most 2 heads
( c ) no head (d) 3 heads ?
(ii) If a fair coin is tossed 10 times, find the probability of :
(a) exactly four heads
(b) exactly six heads
( c ) at least six heads
(d) at most six heads.View SolutionA coin is tossed 6 times . Find the probability of getting at least 3 heads .
View SolutionA coin is tossed 5 times. What is the probability of getting at least 3 heads.
View SolutionA coin is tossed n times . If the probability of getting at least one head is greater than 0.9 then the minimum vaue of n is
View SolutionA die is tossed and when 6 appears then a coin is tossed two times. What is the probability of getting two heads?
View SolutionA coin is tossed n times. The probability of getting at least one head is greater than that of getting at least two tails by 5/32. Then n is :
View SolutionA coin is biased so that a head is twice as likely to occur as a tail. If the coin is tossed 3 times, what is the probability of getting 2 tails and 1 head?
View SolutionA coin is tossed two times. Find the probability of getting atmost one head.
View SolutionA coin is tossed three times. What is the probability of getting head and tail (HTH) or tail and head (THT) alternatively ?
View SolutionA coin is tossed 6 times. Find the probability of getting
(i) exactly 4 heads (ii) at least 1 head (iii) at most 4 heads .View Solution