A laboratory blood test is 99%effective in detecting a certain disease when it is fact present. However, the test also yields a false positive result for 0.5% of the healthy person tested (i.e., if a healthy person is tested, then with probability 0.005, the test will imply he has the disease). If 0.1% of the population actually has the disease, what is the probability that a person has disease given that his test result is positive?
Let E1= person having disease
E'1= person having no disease or healthy
E= result is positive, person has disease
P(E/E1)=99100=0.99
P(E/E'1)=0.005
P(E1)=0.1100=0.001
P(E'1)=1−0.001=0.999
To find: P(E1/E)
P(E1/E)=P(E/E1)⋅P(E1)P(E/E'1)⋅P(E'1)+P(E/E1)⋅P(E1)
P(E1/E)=0.001⋅0.990.005⋅0.999+0.001⋅0.99
P(E1/E)=0.000990.005985
P(E1/E)=110665
P(E1/E)=22133
Topper's Solved these Questions
Similar Questions
A laboratory blood test is 99% effective in detecting a certain disease when it is in fact, present. However, the test also yields a false positive result for 0.5% of the healthy person tested (i.e. if a healthy person is tested, then, with probability 0.005, the test will imply he has the disease). If 0.1 percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive?
View SolutionWhat is personality test ?
View Solution
Knowledge Check
The probability that a teacher will give an unannouned test during any class meeting is 1/5. If a student is absent twice, then the probability that the student will miss at least one test, is
A415B115C9125D16125One percent of the population suffers from a certain disease. There is blood test for this disease, and it is 99% accurate, in other words, the probability that it gives the correct anwer is 0.99, regardless of whether the person is sick or healthy. A person takes the blood test, and the result says that he has the disease. The probability that he actualy has the disease is
A0.99%B25%C50%D75%The person shown in the pictures is affected by a certain disease.
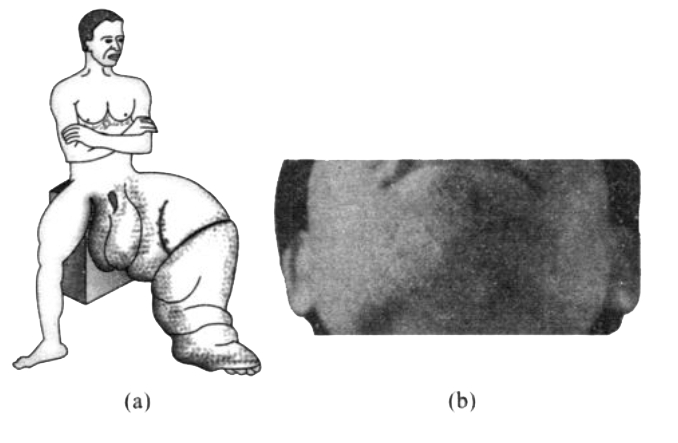 Aa - caused by Wuchereria bancrofti and W Malayi, b - caused by Epidermophyton and MicrosporumBa - Elephantiasis , b - ringwormCa - caused by a nematode , b - caused by fungiDAll of the above
Aa - caused by Wuchereria bancrofti and W Malayi, b - caused by Epidermophyton and MicrosporumBa - Elephantiasis , b - ringwormCa - caused by a nematode , b - caused by fungiDAll of the above
The probability that a teacher will give a surprise test during any class is 1/5. If a student is absent on two day what is the probability that he will miss atleast one test.
View SolutionThe percentage of marks obtained by a student in a monthly test is as follows:
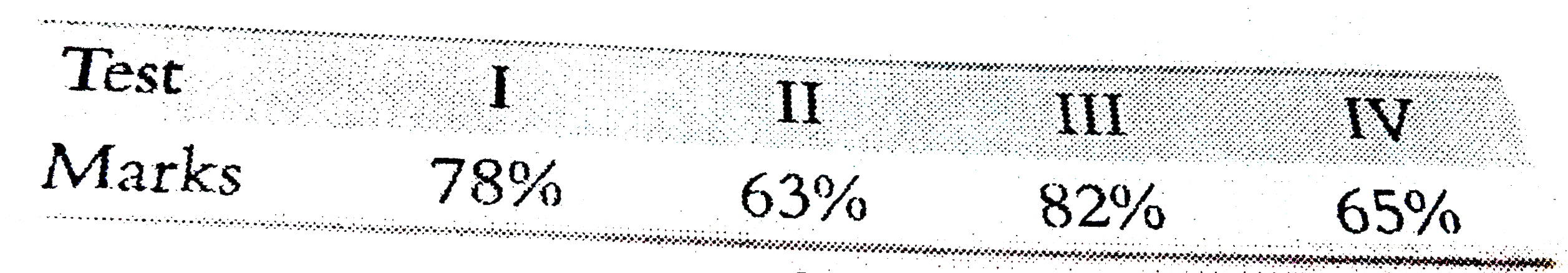
What is the probability that the student gets more than 75% marks in a test?
View SolutionIf a person is born with a hereditary disease, which corrective therapy be taken for such a disease
View SolutionThematic Apperception Test (TAT) of measuring personality is a
View SolutionThematic Apperception Test (TAT) of measuring personality is a
View Solution
