Statement-1: A brass disc is just fitted in a hole in a steel plate. The system mst be cooled to loosen the disc from the hole
Statement-2: The coefficient of linear expansion for brass is greater than the coefficient of linear expansion for steel.

A

Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1.

B

Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1.

C

Statement-1 is false, statement-2 is true.

D

Statement-1 is true, statement-2 is false.

Video Solution
Doubtnut Promotions Banner
|
Answer

Step by step video & image solution for Statement-1: A brass disc is just fitted in a hole in a steel plate. The system mst be cooled to loosen the disc from the hole Statement-2: The coefficient of linear expansion for brass is greater than the coefficient of linear expansion for steel. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12PHYSICSTHERMAL EXPANSION AND CALORIMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The brass disc fits singly in a hole in a steel plate. To loose the disk from hole

    Awe should heat the system
    Bwe should cool the system
    Cwe should apply a strong external force
    Dit can not be separated

  • In figure which strip brass or steel have higher coefficient of linear expansion.
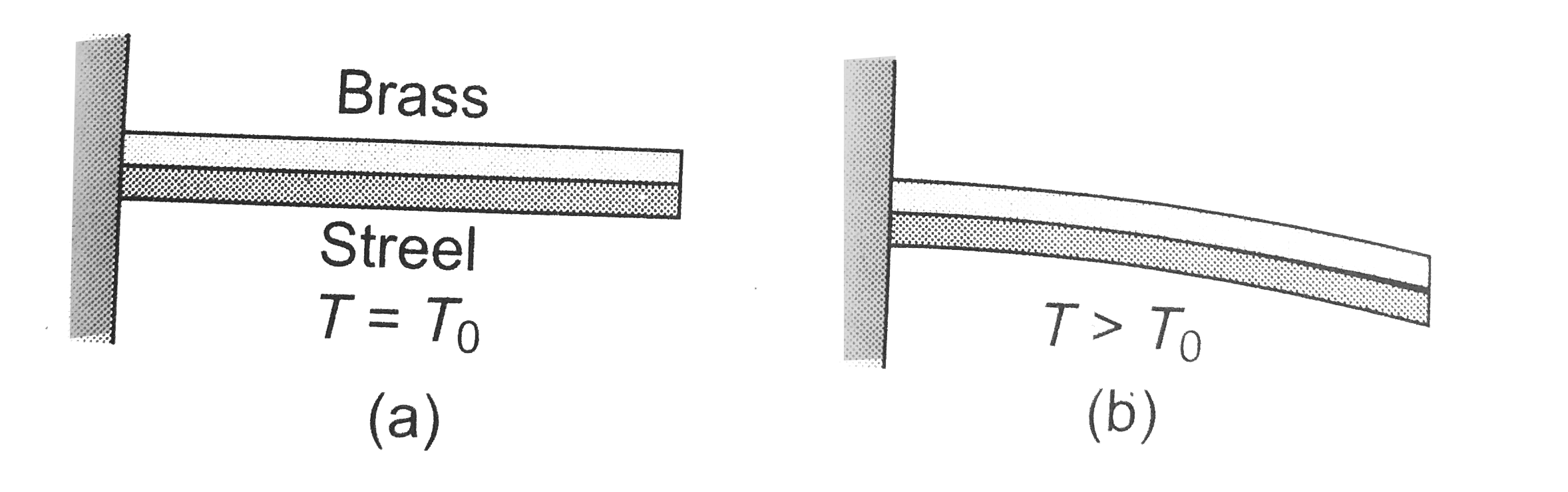    ocr_image

    Abrass strip
    Bsteel strip
    Cboth strips has same coefficient of linear expansion
    Dcannot be decided from given data

  • A brass dise fits into a hole in an iron plate. To remove the disc.

    Athe system must be cooled
    Bthe system must be heated
    Cthe plate may be heated (or) cooled
    Dthe disc must be heated