The path followed by a body projected along y axis is given by y=√3x−(1/2)x2 . If g=10ms2, then the initial velocity of projectile will be – (x and y are in m)
3√10 m/s
2√10 m/s
10√3 m/s
10√2 m/s
To find the initial velocity of the projectile given the trajectory equation y=√3x−12x2 and the acceleration due to gravity g=10m/s2, we can follow these steps:
Step 1: Identify the trajectory equation
The given trajectory equation is:
y=√3x−12x2
This can be compared to the general form of the trajectory equation for projectile motion:
y=xtan(θ)−g2u2cos2(θ)x2
where u is the initial velocity and θ is the angle of projection.
Step 2: Compare coefficients
From the given equation, we can identify:
- The coefficient of x gives us tan(θ)=√3.
- The coefficient of x2 gives us g2u2cos2(θ)=12.
Step 3: Determine the angle of projection
From tan(θ)=√3, we find:
θ=60∘
Step 4: Calculate cos(θ)
For θ=60∘:
cos(60∘)=12
Step 5: Substitute values into the second equation
Now substituting g=10m/s2 and cos(60∘)=12 into the second equation:
102u2(12)2=12
This simplifies to:
102u2⋅14=12
10⋅42u2=12
20u2=12
Step 6: Solve for u2
Cross-multiplying gives:
20=12u2
Multiplying both sides by 2:
40=u2
Step 7: Find u
Taking the square root of both sides:
u=√40=2√10m/s
Final Answer
Thus, the initial velocity of the projectile is:
u=2√10m/s
The given trajectory equation is:
y=√3x−12x2
This can be compared to the general form of the trajectory equation for projectile motion:
y=xtan(θ)−g2u2cos2(θ)x2
where u is the initial velocity and θ is the angle of projection.
From the given equation, we can identify:
- The coefficient of x gives us tan(θ)=√3.
- The coefficient of x2 gives us g2u2cos2(θ)=12.
From tan(θ)=√3, we find:
θ=60∘
For θ=60∘:
cos(60∘)=12
Now substituting g=10m/s2 and cos(60∘)=12 into the second equation:
102u2(12)2=12
This simplifies to:
102u2⋅14=12
10⋅42u2=12
20u2=12
Cross-multiplying gives:
20=12u2
Multiplying both sides by 2:
40=u2
Taking the square root of both sides:
u=√40=2√10m/s
Thus, the initial velocity of the projectile is:
u=2√10m/s
Similar Questions
Knowledge Check
The trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Time of flight of the projectile is :-A√310sB√103sC1sD2sThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]
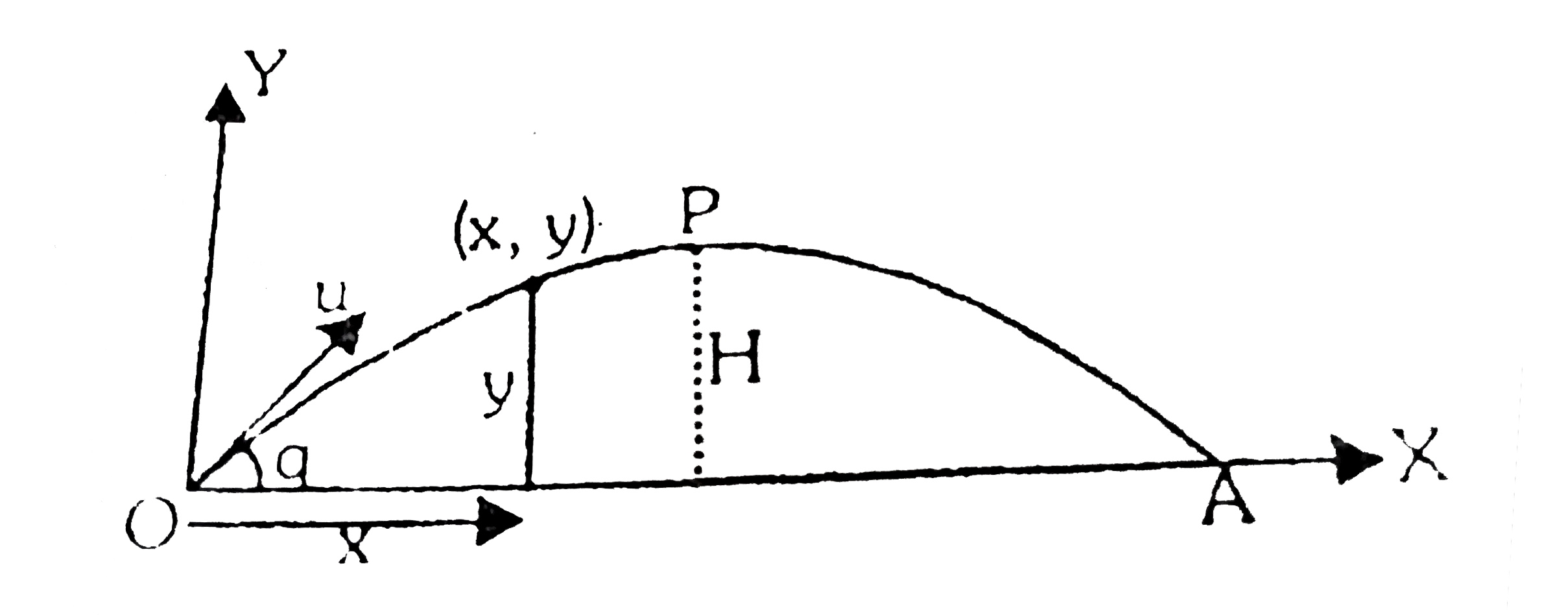
Range OA is :-A√32B√34C√3D38The trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Angle of projection θ is :-A30∘B60∘C45∘D√3 rad
The height and horizontal distances covered by a projectile in a time t are given by y=(4t−5t2)m and x=3tm the velocity of projection is
(A) 4ms−1(B) 3ms−1 (C)5ms−1 (D) 10ms−1
View SolutionThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Maximum height H is :-
View SolutionFor ground to ground projectile motion equation of path is y=12x−3/4x2. Given that g=10ms−2. What is the range of the projectile ?
View SolutionThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Radius of curvature of the path of the projectile at the topmost point P is :-
View SolutionThe equation of trajectory of an oblique projectile y=√3x−gx22. The angle of projection is
View Solution