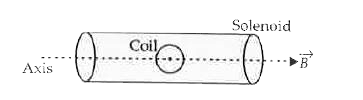
A solenoid of length 0.4 m and having 500 turns of wire carries a current of 3 amp. A thin coil having 10 turns of wire and of radius 0.01 m carries a current of 0.4 amp. The torque (in Nm) required to hold the coil in the middle of the solenoid with its axis perpendicular to the axis of the solenoid is ( V-s/A-m)
To find the torque required to hold the thin coil in the middle of the solenoid, we will follow these steps:
Step 1: Calculate the magnetic field (B) inside the solenoid.
The magnetic field inside a solenoid is given by the formula:
B=μ0⋅n⋅I
where:
- μ0=4π×10−7T m/A (permeability of free space),
- n is the number of turns per unit length of the solenoid,
- I is the current flowing through the solenoid.
First, we need to calculate n:
n=Number of turnsLength of solenoid=5000.4m=1250turns/m
Now, substituting the values into the magnetic field formula:
B=(4π×10−7)⋅(1250)⋅(3)
B=4π×10−7⋅3750
B=4.71×10−3T(approximately)
Step 2: Calculate the area (A) of the thin coil.
The area of the coil is given by the formula:
A=πr2
where r is the radius of the coil. Given r=0.01m:
A=π⋅(0.01)2=π⋅0.0001≈3.14×10−4m2
Step 3: Calculate the magnetic moment (M) of the coil.
The magnetic moment M of the coil is given by:
M=n′⋅I′⋅A
where:
- n′=10 (number of turns in the coil),
- I′=0.4A (current in the coil).
Substituting the values:
M=10⋅0.4⋅(3.14×10−4)
M=1.256×10−3A m2
Step 4: Calculate the torque (τ) on the coil.
The torque experienced by the coil in a magnetic field is given by:
τ=M⋅B
Substituting the values we calculated:
τ=(1.256×10−3)⋅(4.71×10−3)
τ≈5.91×10−6Nm
Final Result:
The torque required to hold the coil in the middle of the solenoid is approximately:
τ≈5.91×10−6Nm
The magnetic field inside a solenoid is given by the formula:
B=μ0⋅n⋅I
where:
- μ0=4π×10−7T m/A (permeability of free space),
- n is the number of turns per unit length of the solenoid,
- I is the current flowing through the solenoid.
n=Number of turnsLength of solenoid=5000.4m=1250turns/m
B=(4π×10−7)⋅(1250)⋅(3)
B=4π×10−7⋅3750
B=4.71×10−3T(approximately)
The area of the coil is given by the formula:
A=πr2
where r is the radius of the coil. Given r=0.01m:
A=π⋅(0.01)2=π⋅0.0001≈3.14×10−4m2
The magnetic moment M of the coil is given by:
M=n′⋅I′⋅A
where:
- n′=10 (number of turns in the coil),
- I′=0.4A (current in the coil).
M=10⋅0.4⋅(3.14×10−4)
M=1.256×10−3A m2
The torque experienced by the coil in a magnetic field is given by:
τ=M⋅B
Substituting the values we calculated:
τ=(1.256×10−3)⋅(4.71×10−3)
τ≈5.91×10−6Nm
The torque required to hold the coil in the middle of the solenoid is approximately:
τ≈5.91×10−6Nm
Topper's Solved these Questions
Similar Questions
A solenoid of length 0⋅4m and having 500 turns of wire carries a current of 3A. A thin coil having 10 turns of wire and of radius 0⋅01m carries a current of 0⋅4A. Calculate the torque required to hold the coil in middle of the solenoid with its axis perpendicular to the axis of the solenoid.
(μ0=4π×10−7V−s/A−m)
View SolutionA thin solenoid of length 0.4m and having 500 turns of wire carries a current 1A,then find the magnetic field on the axis inside the solenoid.
View Solution
Knowledge Check
A solenoid of 0.4 m length with 500 turns carries a current of 3 A. A coil of 10 tums and of radius 0.01 m carries a current of 0.4 A. The torque required to hold the coil with its axis at right angle to that of solenoid in the middle point of it is
A6π2×10−7NmB3π2×10−7NmC9π2×10−7NmD12π2×10−7NmA circular coil having 50 turns each of radius 0.05 m carries a current of 1 A. The magnitude of magnetic induction at a point 0.2 m from its centre on its axis is
A9×10−6 gaussB9×10−5 N/A mC9×10−4 TD9×10−6Wb/m2A solenoid of length 0.5 m has a radius of 1 cm and is made up of 500 turns. It carries a current of 5 A. The magnetic field inside the solenoid is
A3.14×10−3TB6.28×10−3TC9.14×10−3TD1.68×10−3T
A solenoid of length 0.4m and having 500 turns of wire carries a current of 3amp.Calculate the torque requred to hold a coil (having radius 0.02cm current 2A and turns 50)in the middle of the solenoid with its axis perpendicular to the axis of the solenoid.(π2=10)
View SolutionA coil made of 10 turns of a wire wound in a circular loop of radius0.02 m, carries a current of 0.5∧ and is placed inside a solenoid of 400 tums and length 0.5 m. If the solenoid carries a current of 0.2 A, what torque will be required to hold the coil in the centre of the solenoid when placed with its plane along the axis of solenoid.
View SolutionA circular loop of radius 0.0157m carries a current of 2.0 amp. The magnetic field at the centre of the loop is
(μ0=4π×10−7weber/amp−m)
View SolutionThe torque required to hold a small circular coil of 10 turns, area 1 m m2and carrying a current of(2144) A JA in the middle of a long solenoid of 103 turns/m carrying a current of 2.5 A, with its axis perpendicular to the axis of the solenoid
View SolutionThe torque required to hold a small circular coil of 10 turns, area 1mm2 and carrying a current of (2144)A in the middle of a long solenoid of 103turns/m carrying a current of 2.5A, with its axis perpendicular to the axis of the solenoid is
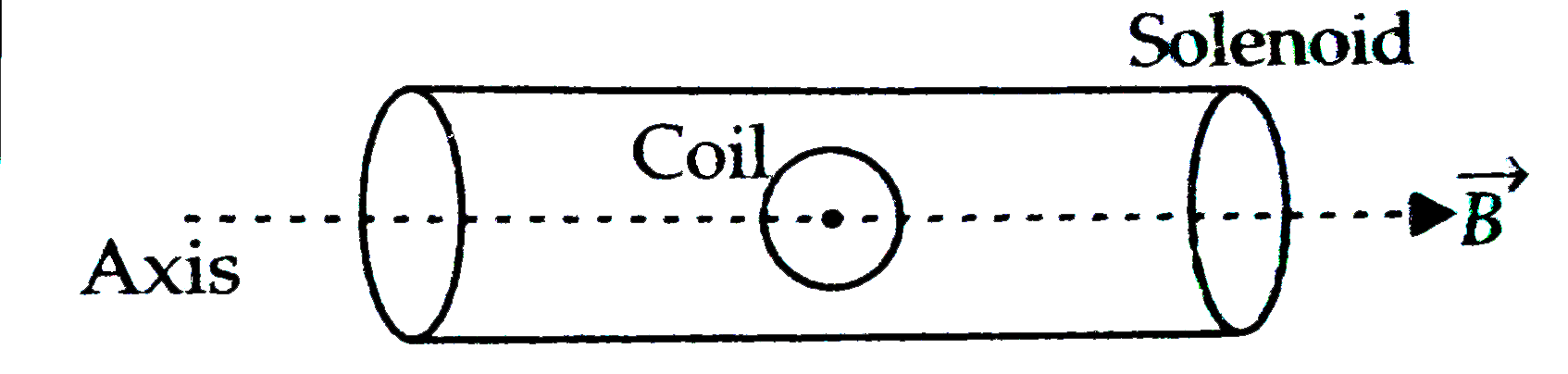
View Solution
