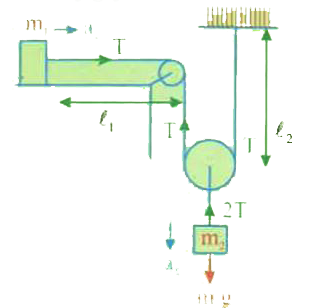
Statement– 1 : In the given fig. a=(m2−m1m1+m2)g
Statement– 2 : In the given fig., T=m1+m22m1m2
Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1.
Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1.
Statement -1 is False, Statement-2 is True
Statement -1 is True, Statement-2 is False
Step by step video & image solution for Statement– 1 : In the given fig. "a"=(("m"_(2)-"m"_(1))/("m"_(1)+"m"_(2)))"g" Statement– 2 : In the given fig., "T"=("m"_(1)+"m"_(2))/(2"m"_(1)"m"_(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
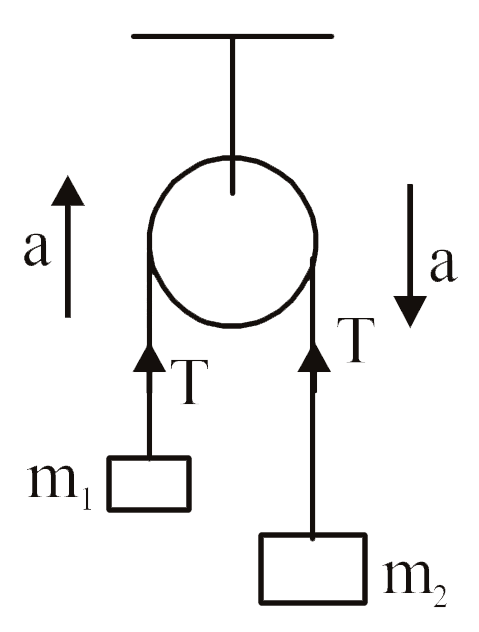
Consider the case of two bodies of masses m1andm2 which are connected by light inextensible string passing over a light smooth pulley as shown in the figure. The expression for acceleration of the system and tension of the string are expressed below under different situations:
(i) when m1>m2. In this case
a=(m1−m2m1+m2)gandT=(2m1m2m1+m2)g
(ii) when m2>m1. In this case
a=(m2−m1m1+m2)gandT=(2m1m2m1+m2)g
(iii) When m1=m2=m. In this case a=0,T=mg
What is the tension in the string?A60NB75NC100ND600NConsider the case of two bodies of masses m1andm2 which are connected by light inextensible string passing over a light smooth pulley as shown in the figure. The expression for acceleration of the system and tension of the string are expressed below under different situations:
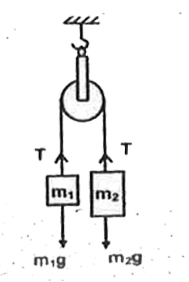
(i) when m1>m2. In this case
a=(m1−m2m1+m2)gandT=(2m1m2m1+m2)g
(ii) when m2>m1. In this case
a=(m2−m1m1+m2)gandT=(2m1m2m1+m2)g
(iii) When m1=m2=m. In this case a=0,T=mg
If the pulley is pulled upward with acceleration equal to the acceleration due to gravity, what will be the tension in the string?A75NB150NC300ND500NConsider the case of two bodies of masses m1andm2 which are connected by light inextensible string passing over a light smooth pulley as shown in the figure. The expression for acceleration of the system and tension of the string are expressed below under different situations:
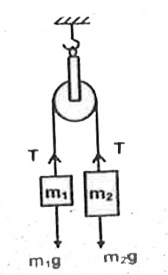
(i) when m1>m2. In this case
a=(m1−m2m1+m2)gandT=(2m1m2m1+m2)g
(ii) when m2>m1. In this case
a=(m2−m1m1+m2)gandT=(2m1m2m1+m2)g
(iii) When m1=m2=m. In this case a=0,T=mg
If m1=10kg,m2=6kgandg=10m/s2 then what is the acceleration of masses?A2.5m/s2B5m/s2C20m/s2D40m/s2
The value of ∞∑m=1tan−1(2mm4+m2+2) is
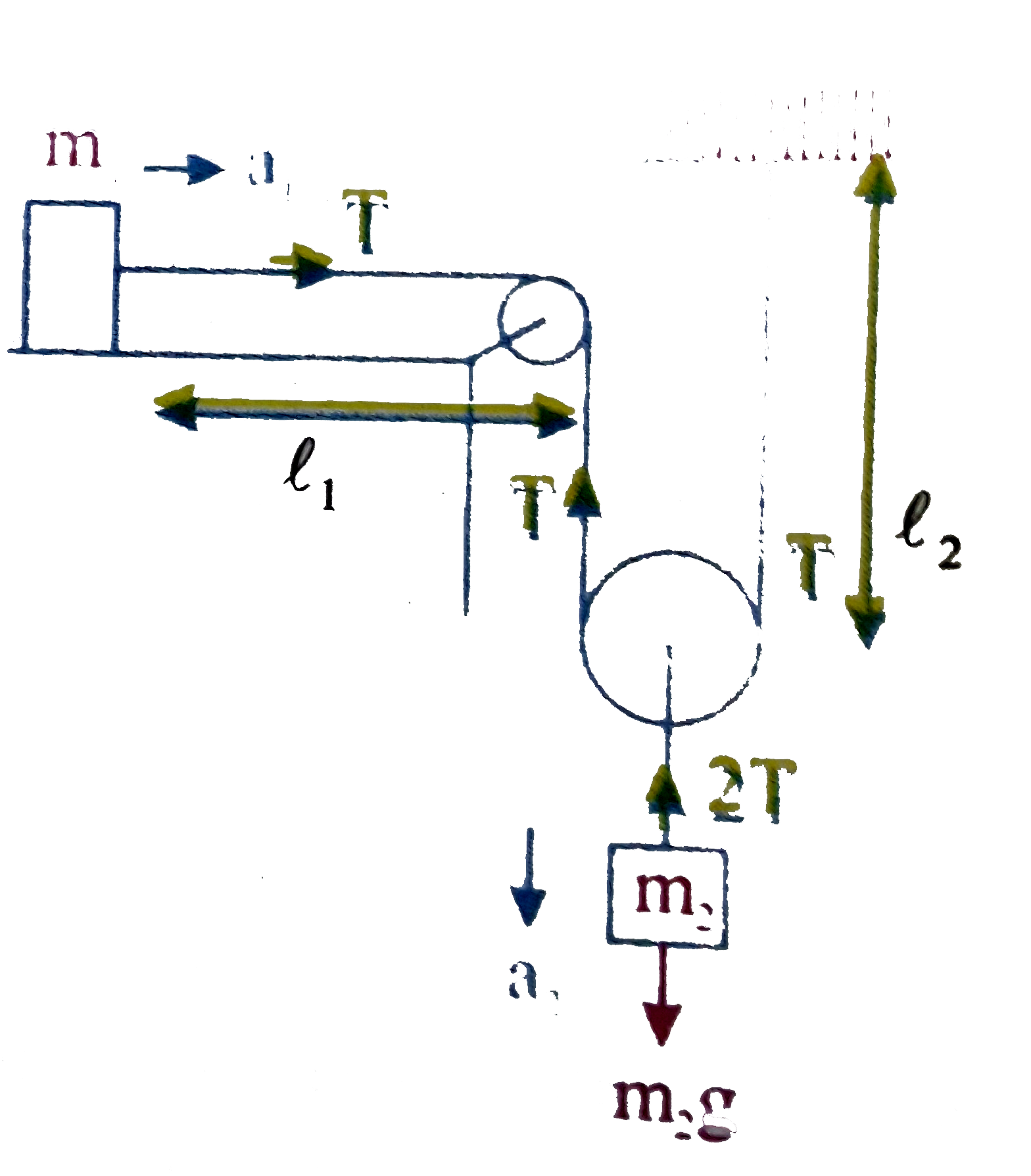
View SolutionIn the fig, find the acceleration of m1 and m2
 .
.
View SolutionIf m−1m=5 then find m2−1m2
View SolutionShow that in the case of an elastic collision between a particle of mass m1 with a particle of mass m2 initially at rest , (a) the maximum angle through which m1 can be deflected is given by 1−cos2θm=m22m21 when m1>m2 (b) θ1+θ2=π2 when m1=m2
View SolutionThe value of n∑m=1tan−1(2mm4+m2+2) is :
View Solution