What is the edge length of a cube whose volume is 4,096cm3 ?
16 cm
1,365.33 cm
64 cm
455.11 cm
The correct Answer is:A
To find the edge length of a cube whose volume is 4096cm3, we can follow these steps:
Step 1: Understand the formula for the volume of a cube
The volume V of a cube is given by the formula:
V=a3
where a is the length of an edge of the cube.
Step 2: Set the volume equal to the given value
We know that the volume of the cube is 4096cm3. Therefore, we can set up the equation:
a3=4096
Step 3: Solve for a by finding the cube root
To find the edge length a, we need to take the cube root of both sides of the equation:
a=√(3)4096
Step 4: Calculate the cube root
Now we need to calculate √(3)4096. We can do this by recognizing that 4096 is a perfect cube.
To find the cube root:
1. Factor 4096 into its prime factors.
2. We find that 4096=16×16×16 or 163.
3. Therefore, √(3)4096=16.
Step 5: State the final answer
Thus, the edge length a of the cube is:
a=16cm
Summary
The edge length of the cube is 16cm.
---
The volume V of a cube is given by the formula:
V=a3
where a is the length of an edge of the cube.
We know that the volume of the cube is 4096cm3. Therefore, we can set up the equation:
a3=4096
To find the edge length a, we need to take the cube root of both sides of the equation:
a=√(3)4096
Now we need to calculate √(3)4096. We can do this by recognizing that 4096 is a perfect cube.
1. Factor 4096 into its prime factors.
2. We find that 4096=16×16×16 or 163.
3. Therefore, √(3)4096=16.
Thus, the edge length a of the cube is:
a=16cm
The edge length of the cube is 16cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
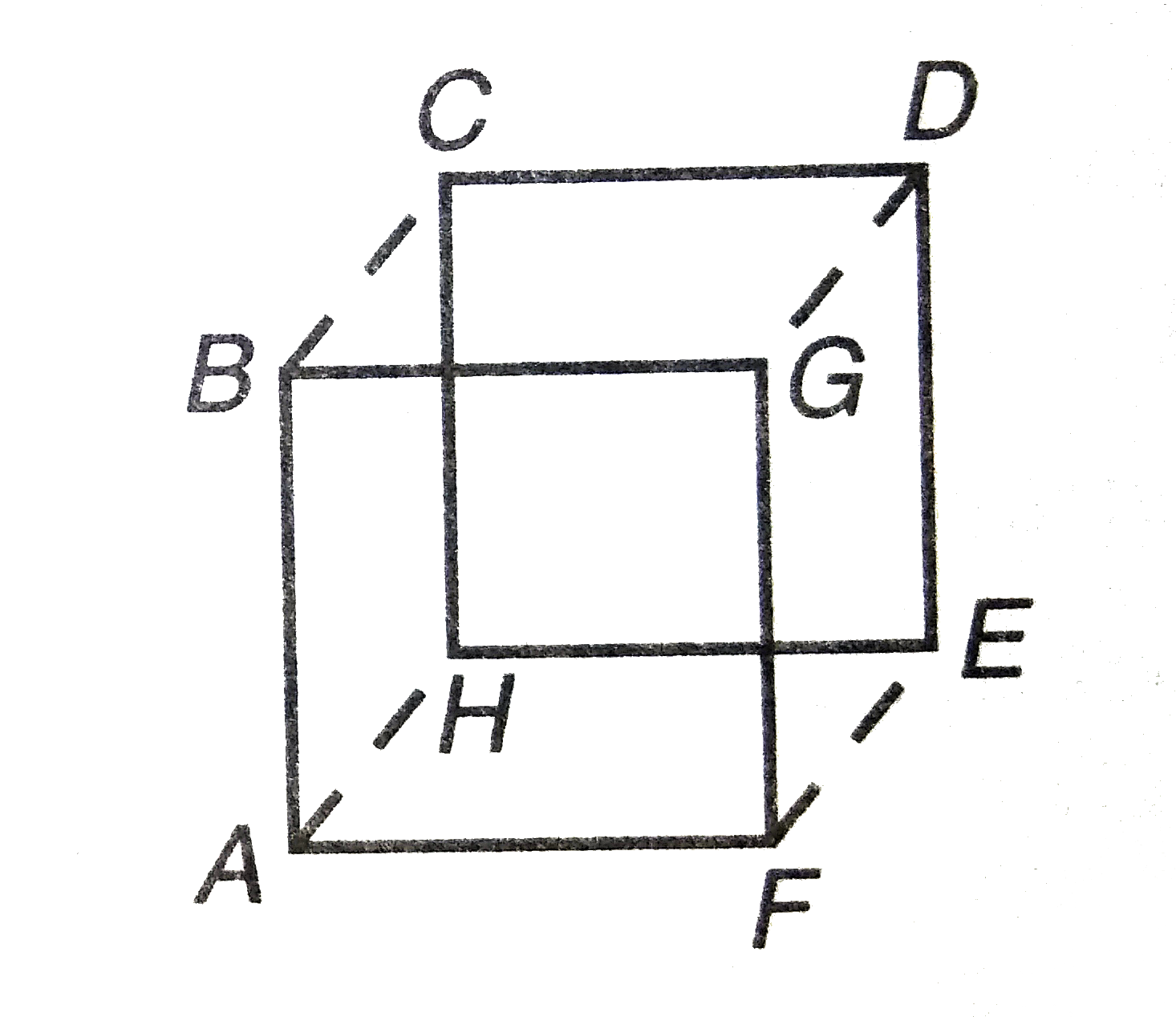
In the figure above, if the edge length of the cube is 4, what is the shortest distance from A to D?A4√2B4√3C8D4√2÷4In cubic meters , what is the volume of a large cube whose edges each measure 6 meters in length ?
A18B36C64D216The unit cell of aluminium is a cube with an edge length of 405 pm. The density of aluminium is 2.70gcm−3 . What type of unti celll of alluminium is ?
ABody centred cubic cellBFace-centred cubic cellCEnd-centred cubic cellDSimple cubic cell
A cube whose volume is 1/8 cubic centimetre is placed on top of a cube whose volume is 1 cm3. The two cubes are then placed on top of a third cube whose volume is 8 cm3. The height of the stacked cubes is (a) 3.5 cm (b) 3 cm (c) 7 cm (d) none of them
View SolutionFind the length of each edge of a cube. If its volume is
216cm3
(ii) 1.728m3
View SolutionFind the surface area of a cube whose edge is 11cm.
View SolutionIf the sum of all the edges of a cube is 36 cm, then the volume (in cm3) of that cube is (a) 9 (b) 27 (c) 219 (d) 729
View SolutionIf the length of an edge of cube A is one-third the length of an edge of cube B, what is the ratio of the volume of cube A to the volume of cube B?
View Solution
