Question

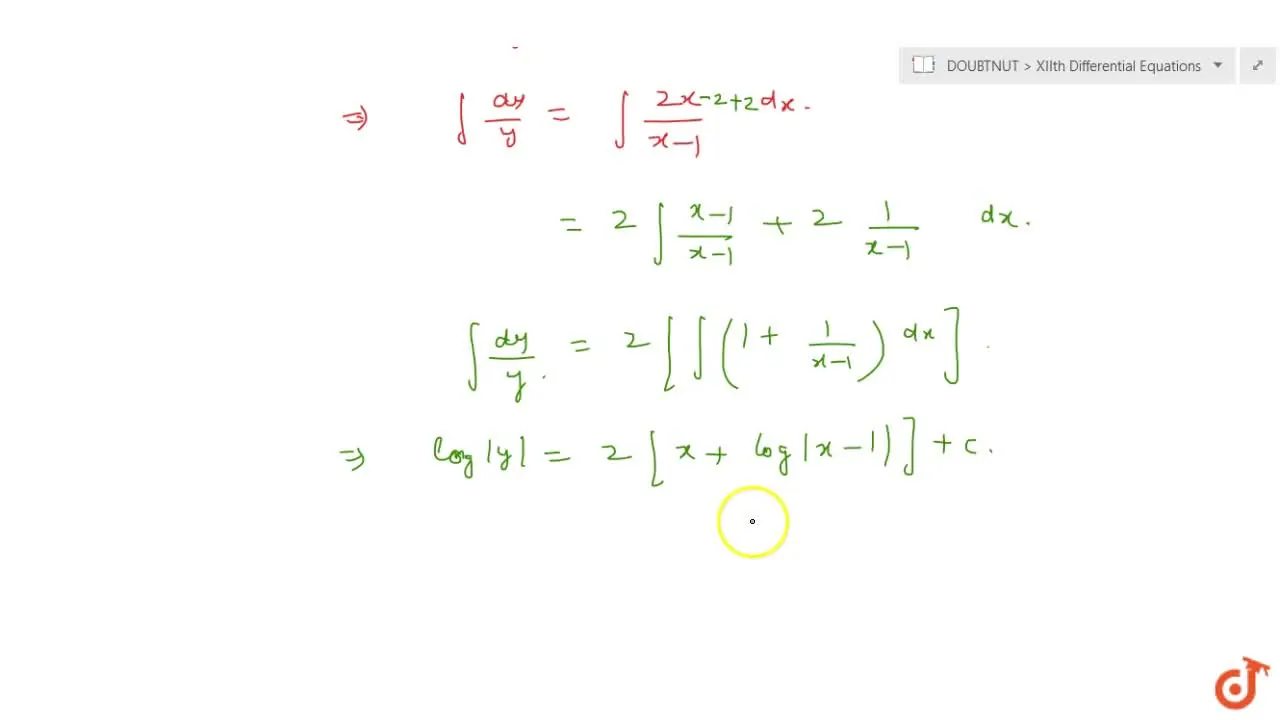
Solve the following differential equation: $(x-1)\frac{dy}{dx}=2xy$

Explanation

Provided differential equation is
$$ \begin{aligned} &(\mathrm{x}+1)(\mathrm{dy} / \mathrm{dx})=2 \mathrm{xy} \\ &\Rightarrow \frac{d y}{y}=\left(\frac{2 x}{x+1}\right) d x \\ &\Rightarrow \frac{d y}{y}=2\left(1-\frac{1}{x+1}\right) d x \end{aligned} $$
On Integrating both sides, we get

`\log |y|=2 x-2 \log |x+1|+c`

which is the required solution.