A particle of mass 4m which is at rest explodes into masses m,m & 2m. Two of the fragments of masses m and 2m are found to move with equal speeds v each in opposite directions. The total mechanical energy released in the process of explosion is :-
mv2
2mv2
1/2mv2
4mv2
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the Initial Conditions
A particle of mass 4m is initially at rest. When it explodes, it breaks into three fragments with masses m, m, and 2m.
Hint: Remember that the initial momentum of the system is zero since the particle is at rest.
Step 2: Analyze the Final Conditions
After the explosion, two fragments of masses m and 2m move with equal speeds v in opposite directions. Let’s denote the speed of the third fragment (mass m) as v1.
Hint: The direction of the velocities is important; assign a positive direction for clarity.
Step 3: Apply Conservation of Momentum
Since there are no external forces acting on the system, the total momentum before the explosion must equal the total momentum after the explosion.
- Initial momentum = 0 (since the particle was at rest)
- Final momentum = (mass of 2m) * (velocity of 2m) + (mass of m) * (velocity of m) + (mass of m) * (velocity of m)
Setting up the equation:
\( 0 = 2m(-v) + mv1 + mv \)
This simplifies to:
\( 0 = -2mv + mv1 + mv \)
Rearranging gives:
\( mv1 = 2mv - mv \)
\( mv1 = mv \)
\( v1 = v \)
Hint: Use the conservation of momentum to relate the velocities of the fragments.
Step 4: Calculate the Kinetic Energy of Each Fragment
Now, we can calculate the kinetic energy of each fragment after the explosion.
- Kinetic energy of mass 2m moving with speed v:
\( KE_{2m} = \frac{1}{2} \cdot 2m \cdot v^2 = mv^2 \)
- Kinetic energy of one mass m moving with speed −v:
\( KE_{m} = \frac{1}{2} \cdot m \cdot v^2 = \frac{1}{2} mv^2 \)
- Kinetic energy of the other mass m moving with speed v1 (which we found to be equal to v):
\( KE_{m} = \frac{1}{2} \cdot m \cdot v^2 = \frac{1}{2} mv^2 \)
Step 5: Total Kinetic Energy After Explosion
Now, we sum the kinetic energies of all fragments:
\( KE_{total} = KE_{2m} + KE_{m} + KE_{m} \)
\( KE_{total} = mv^2 + \frac{1}{2} mv^2 + \frac{1}{2} mv^2 \)
\( KE_{total} = mv^2 + mv^2 = 2mv^2 \)
Step 6: Determine the Total Mechanical Energy Released
Since the initial kinetic energy was zero (the particle was at rest), the total mechanical energy released in the explosion is equal to the total kinetic energy after the explosion:
\( \text{Total Mechanical Energy Released} = KE_{total} = 2mv^2 \)
Conclusion
Thus, the total mechanical energy released in the process of explosion is:
\( \boxed{2mv^2} \)
---
A particle of mass 4m is initially at rest. When it explodes, it breaks into three fragments with masses m, m, and 2m.
After the explosion, two fragments of masses m and 2m move with equal speeds v in opposite directions. Let’s denote the speed of the third fragment (mass m) as v1.
Since there are no external forces acting on the system, the total momentum before the explosion must equal the total momentum after the explosion.
- Final momentum = (mass of 2m) * (velocity of 2m) + (mass of m) * (velocity of m) + (mass of m) * (velocity of m)
\( 0 = 2m(-v) + mv1 + mv \)
\( 0 = -2mv + mv1 + mv \)
\( mv1 = 2mv - mv \)
\( mv1 = mv \)
\( v1 = v \)
Now, we can calculate the kinetic energy of each fragment after the explosion.
\( KE_{2m} = \frac{1}{2} \cdot 2m \cdot v^2 = mv^2 \)
\( KE_{m} = \frac{1}{2} \cdot m \cdot v^2 = \frac{1}{2} mv^2 \)
\( KE_{m} = \frac{1}{2} \cdot m \cdot v^2 = \frac{1}{2} mv^2 \)
Now, we sum the kinetic energies of all fragments:
\( KE_{total} = KE_{2m} + KE_{m} + KE_{m} \)
\( KE_{total} = mv^2 + \frac{1}{2} mv^2 + \frac{1}{2} mv^2 \)
\( KE_{total} = mv^2 + mv^2 = 2mv^2 \)
Since the initial kinetic energy was zero (the particle was at rest), the total mechanical energy released in the explosion is equal to the total kinetic energy after the explosion:
\( \text{Total Mechanical Energy Released} = KE_{total} = 2mv^2 \)
Thus, the total mechanical energy released in the process of explosion is:
\( \boxed{2mv^2} \)
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-III
42 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A particle of mass 4m, which is at rest, explodes into masses m,m and 2m, two of the fragments of masses m and 2m are found to move with equal speed v each in opposite directions. The total mechanical energy released in the process of explosion is kmv2. Find k.
View SolutionA particle of mass 4 m which is at rest explodes into three fragments. Two of the fragments each of mass m are found to move with a speed v each in mutually perpendicular directions. The total energy released in the process of explosion is ............
View Solution
Knowledge Check
A particle of mass 4m which is at rest explodes into four equal fragments. All four fragments scattered in the same horizontal plane. Three fragments are found to move with velocity v as shown in the figure. The total energy released in the process is
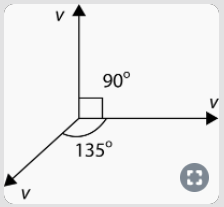 A(a) mv2(3−√2)B(b) 12mv2(3−√2)C(c) 2mv2D(d) 12mv2(1+√2)
A(a) mv2(3−√2)B(b) 12mv2(3−√2)C(c) 2mv2D(d) 12mv2(1+√2)A particle of mass 4m which is at rest explodes into four equal fragments. All 4 fragments are scattered in the same horizontal plane. Three fragments are found to move with velocity V each as shown in the figure. The total energy released in the process of explosion is
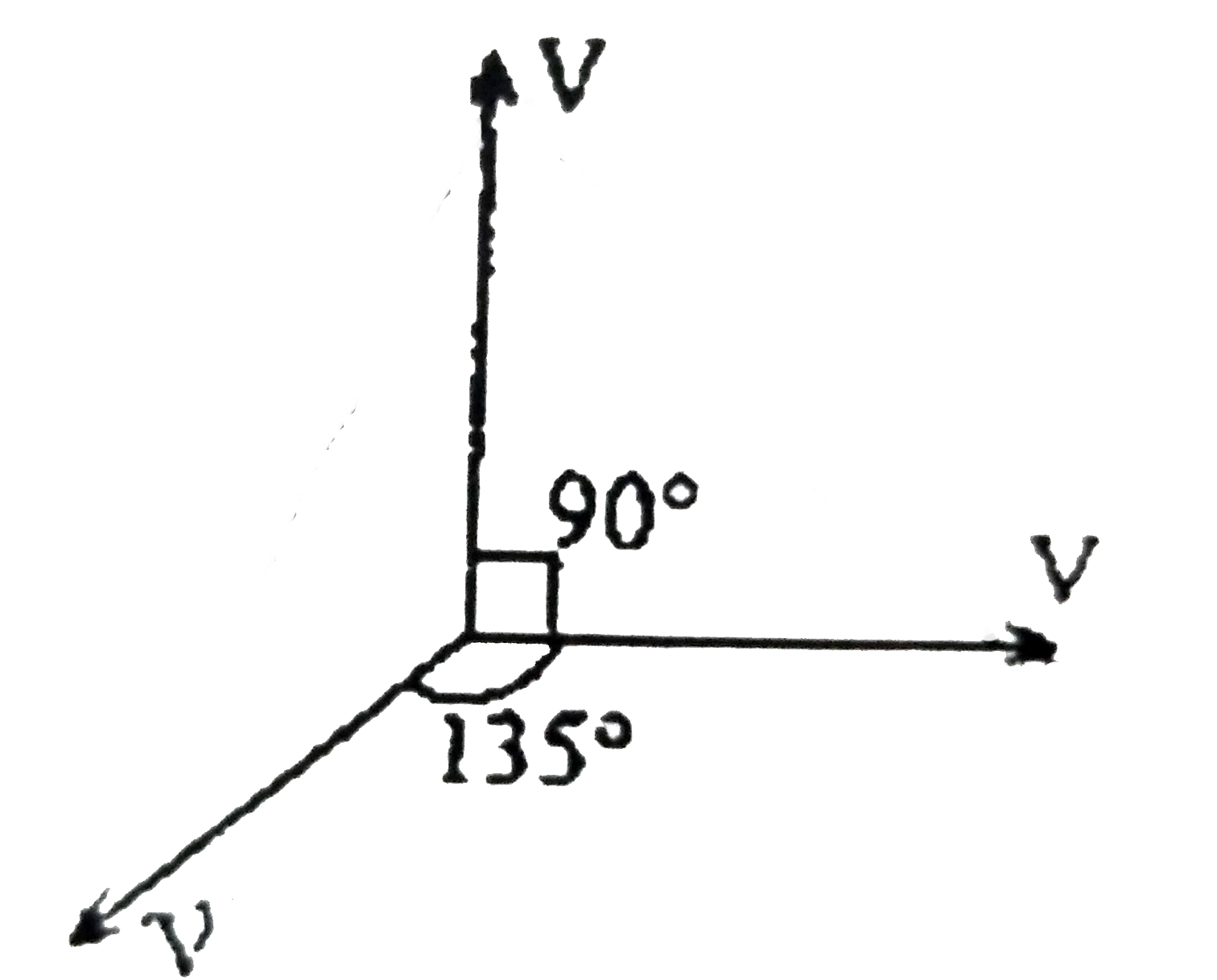 AmV2(3−√2)Bmv2(3−√2)/2C2mV2DmV2(1+√2)/2
AmV2(3−√2)Bmv2(3−√2)/2C2mV2DmV2(1+√2)/2A body of mass 4m at rest explodes into three fragments. Two of the fragments each of mass m move with speed v in mutually perpendicular directions. Total energy released in the process is
Amv2B32mv2C2mv2D3mv2
A body of mass 4m at rest explodes into three pieces. Two of the pieces each of mass m move with a speed v each in mutually perpendicular directions. The total kinetic energy released is
View SolutionThere is an object of mass 5m kept at rest in space. Suddenly it explodes in three parts of masses m , 2m and 2m . Two parts of equal masses are found to move with equal speeds v along perpendicular directions . If energy released in explosion is nmv2 , then find value of n .
View SolutionA bomb of mass '5m' at rest explodes into three parts of masses 2m, 2m and m. After explosion, the equal parts move at right angles with speed v each. Find speed of the third part and total energy released during explosion.
View SolutionA body of mass 5m initially at rest explodes into 3 fragments with mass ratio 3:1:1. Two of fragments each of mass 'm' are found to move with a speed 60m/s in mutually perpendicular direction. The velocity of third fragment is
View SolutionA particle of mass 5 m at rest suddenly breaks on its own into three fragments . Two fragments of mass m of each move along mutully perpendicular direction with speed v each . The energy released suring the process is
View Solution
