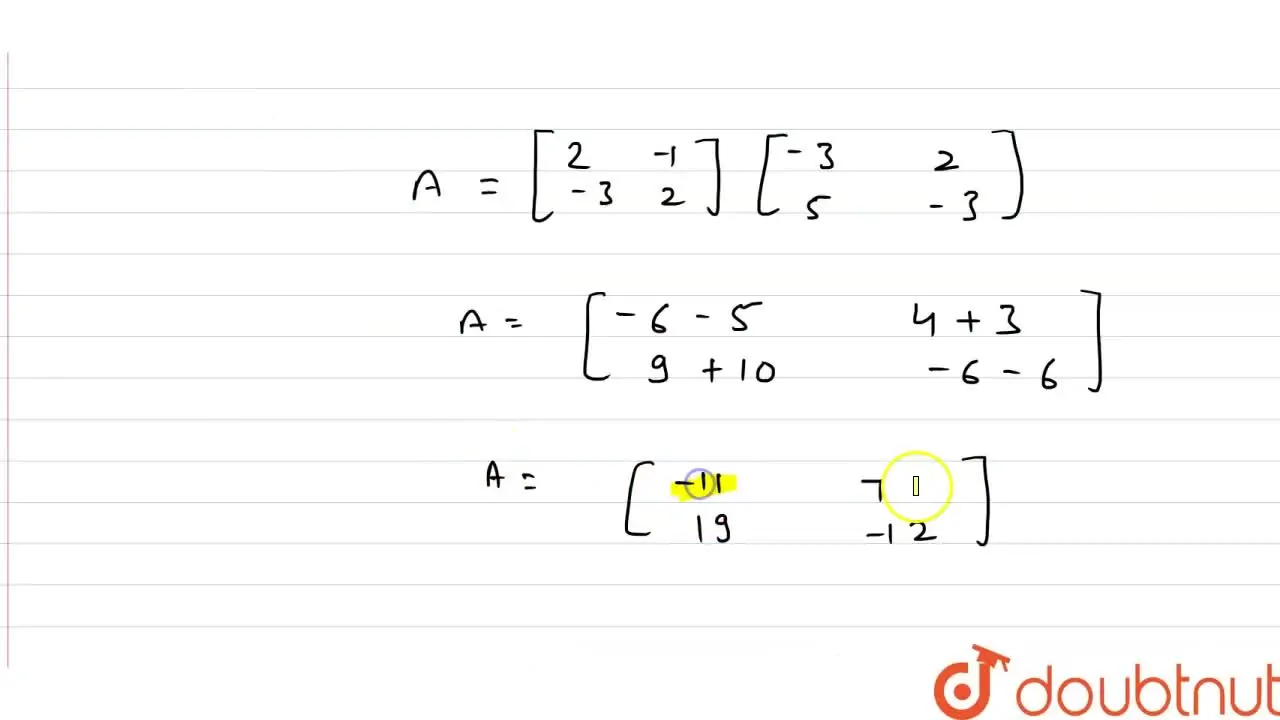
आव्यूह समीकरण को सन्तुष्ट करने वाले आव्यूह A ज्ञात कीजिए ।
Step by step video, text & image solution for आव्यूह समीकरण [(2,1),(3,2)] A[(3,2),(5,3)]=[(1,0),(0,1)] को सन्तुष्ट करने वाले आव्यूह A ज्ञात कीजिए । by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - RAJEEV BANSALCHAPTER - आव्यूह EXERCISE - एन. सी. ई. आर. टी. एक्सेम्पलर (दीर्घ उत्तरीय प्रश्न )5videos
आव्यूह
- BOOK - RAJEEV BANSALCHAPTER - आव्यूह EXERCISE - एन. सी. ई. आर. टी. एक्सेम्पलर (वस्तुनिष्ठ प्रश्न )15videos
आव्यूह
- BOOK - RAJEEV BANSALCHAPTER - आव्यूह EXERCISE - एन. सी. ई. आर. टी. कॉर्नर15videos
आव्यूह
- BOOK - RAJEEV BANSALCHAPTER - अवकलनीयता EXERCISE - कम्पटीशन कॉर्नर3videos
अवकलनीयता
- BOOK - RAJEEV BANSALCHAPTER - आव्यूह के सहखण्डज और प्रतिलोम EXERCISE - कम्पटीशन कॉर्नर2videos
आव्यूह के सहखण्डज और प्रतिलोम
Similar Questions
यदि किन्ही दो वर्ग आव्यूहों के लिए AB=BA हो तो गणितीय आगम से सिद्ध कीजिए कि :
(AB)n=AnBn09:39View Solutionआव्यूह P=⎡⎢⎣004040400⎤⎥⎦ है :
07:46View Solutionयदि A=[0−tan.α2tan.α20] और I,2 कोटि का तत्समक आव्यूह हो तो दिखाइए कि :
(I+A)=(I−A)[cosα−sinαsinαcosα]07:39View Solutionमाना कि f:R→R इस प्रकार का अवकलनीय है कि f(0)=0,f(π2)=3 एवं f'(0)=1 है। यदि x∈(0,π2] के लिए g(x)=∫π/2x[f' (t) cosec t - cot t. cot t. cosec t f(t)]dt है तब limx→0g(x) = .......... .
06:02View Solutionयदि A=[2−3−41] है, तो adj(3A2+12A) बराबर है:
06:08View SolutionIf [2132]A[−325−3]=[1001] then a is equal to (A) [0111] (B) [1011] (C) [1110] (D) [1101]
04:46View Solutionif[{:(2,1),(3,2):}]A[{:(-3,2),(5,-3):}]=[{:(1,0),(0,1):}],"then" A=?
06:22View Solutionआव्यूह समीकरण
[2132].A.[−325−3]=[1001] को संतुष्ट करता हुआ आव्यूह A ज्ञात करे ।03:56View SolutionIf [(2,1),(3,2)]A[(-3,2),(5,-3)]=[(1,0),(0,1)] then the matrix A=
02:22View Solutionसमीकरण tan2θ+sec2θ=1 को सन्तुष्ट करने वाले θ के व्यापक मान …………………. है।
06:17View SolutionIf A is a 2×2 matrix such that [2132]A[−325−3]=[1001]then the sum of the elements in A is
06:57View SolutionIf [2174]A[−325−3]=[1001] then matrix A equals
05:42View Solutionif[{:(2,1),(3,2):}]A[{:(-3,2),(5,-3):}]=[{:(1,0),(0,1):}],"then" A=?
06:18View SolutionFind the matrix A satisfying the matrix equation :
[2132]⋅A⋅[−325−3]=[1001]05:42View Solutionif[{:(2,1),(3,2):}]A[{:(-3,2),(5,-3):}]=[{:(1,0),(0,1):}],"then" A=?
08:28View Solution