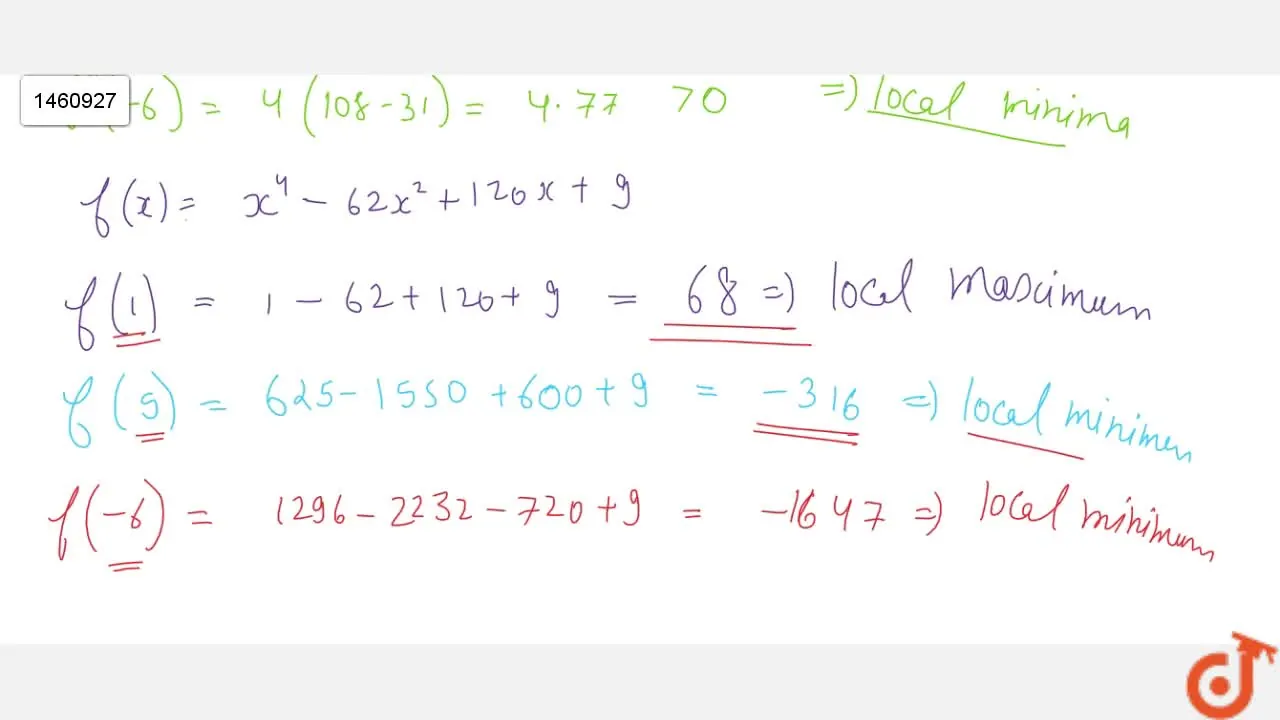Find the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x4−62x2+120x+9 . Also, find the points of inflection, if any:
Given: f(x)=x4−62x2+120x+9
⇒f′(x)=4x3−124x+120
For the local maxima or minima, we must have
f′(x)=0
⇒4x3−124x+120=0
⇒x3−31x+30=0
⇒(x−1)(x2+x−30)=0
⇒(x−1)(x+6)(x−5)=0
⇒x=1,5 and −6
Thus, x=1,x=5 and x=−6 are the possible points of local maxima or local minima.
Now,
f''(x)=12x2−124
At x=1:−
f''(1)=12(1)2−124=−112<0
So, x=1 is the point of local maximum.
The local maximum value is given by f(1)=14−62(1)2+120×1+9=68
At x=5:
f''(5)=12(5)2−124=176>0
So, x=5 is the point of local minimum .
The local minimum value is given by
f(5)=54−62(5)2+120×5+9=−316
At x=−6 :
f''(−6)=12(−6)2−124=308>0
So, x=−6 is the point of local maximum .
The local minimum value is given by
f(−6)=(−6)4−62(−6)2+120×(−6)+9=−1647
Related Playlists
Similar Questions
Find the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x4−62x2+120x+9 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x+√1−x, x≤1
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x3−2ax2+a2 x , a>0, x∈R . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x3−6x2+9x+15 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=2/x−2/x2, x>0 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=(x+1)(x+2)1/3, x≥−2 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x√32−x2, −5≤x≤5 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x+a2x, a>0, x≠0 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=(x−1)(x+2)2 . Also, find the points of inflection, if any:
View SolutionFind the points of local maxima or minima and corresponding local maximum and minimum values of f(x)=x ex . Also, find the points of inflection, if any:
View Solution