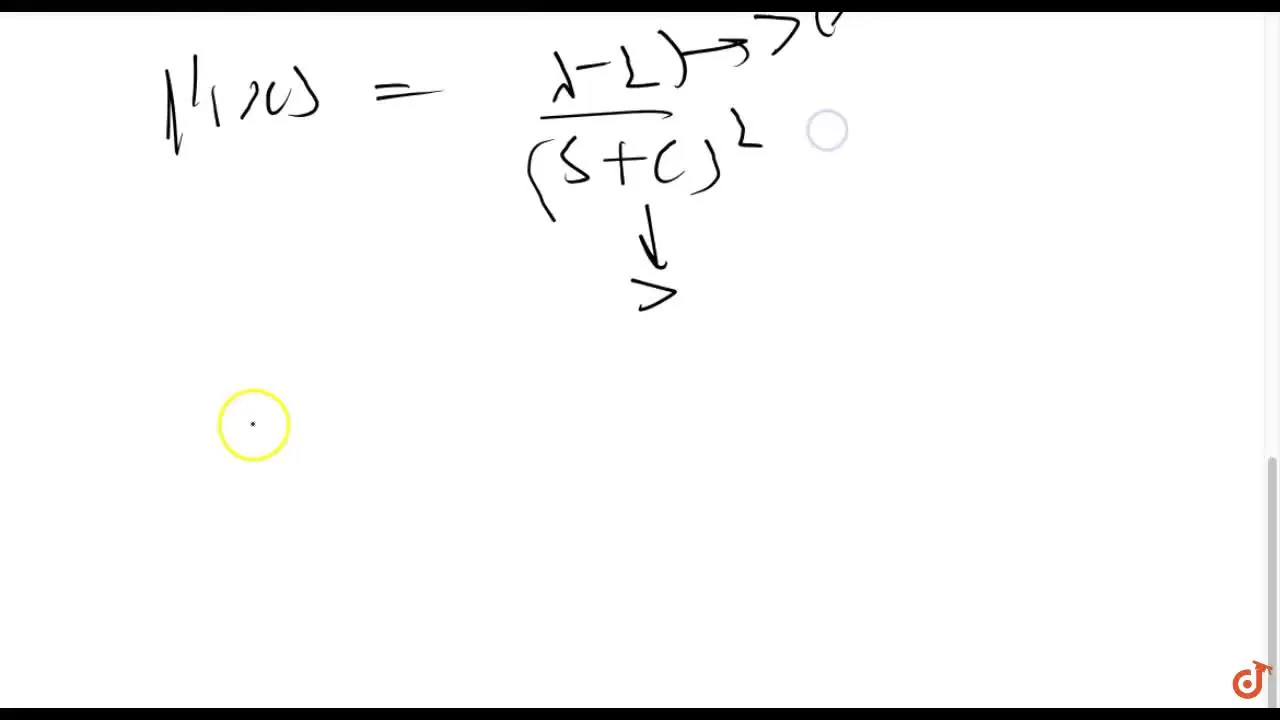The function f(x)=λsinx+2cosxsinx+cosx is increasing, if (a) λ<1 (b) λ>1 (c) λ<2 (d) λ>2
Video Solution
Text Solution
f(x)=λsinx+2cosxsinx+cosx
for increasing
f′(x)>0
⇒(sinx+cosx)(λcosx−2sinx)−(λsinx+2cosx)(cosx−sinx)(sinx+cosx)2>0
⇒λ−2(sinx+cosx)2>0
but(sinx+cosx)2>0
∴λ−2>0
λ>2
|
Updated on:21/07/2023
Related Playlists
Similar Questions
The function f(x)=λsinx+2cosxsinx+cosx is increasing, if (a) λ<1 (b) λ>1 (c) λ<2 (d) λ>2
View SolutionThe function f(x)=tan−1(sinx+cosx) is an increasing function in
View Solutionf(x)=tan−1(sinx+cosx),then f(x) is increasing in
View SolutionThe peroid of the function f(x)=|sinx|−|cosx||sinx+cosx| is
View SolutionThe range of the function
f(x)=1|sinx|+1|cosx| isView Solution∫dx(sinx+cosx)(2cosx+sinx)=
View SolutionThe period of the function
f(x)=|sinx|−|cosx| isView SolutionThe function f defined by f(x)=4sinx−2x−xcosx2+cosx,0≤x≤2π is :
View Solutionf(x)=esinx+cosx is an increasing function in
View SolutionThe maximum value of function f(x)=sinx(1+cosx),ξniR is
View Solution