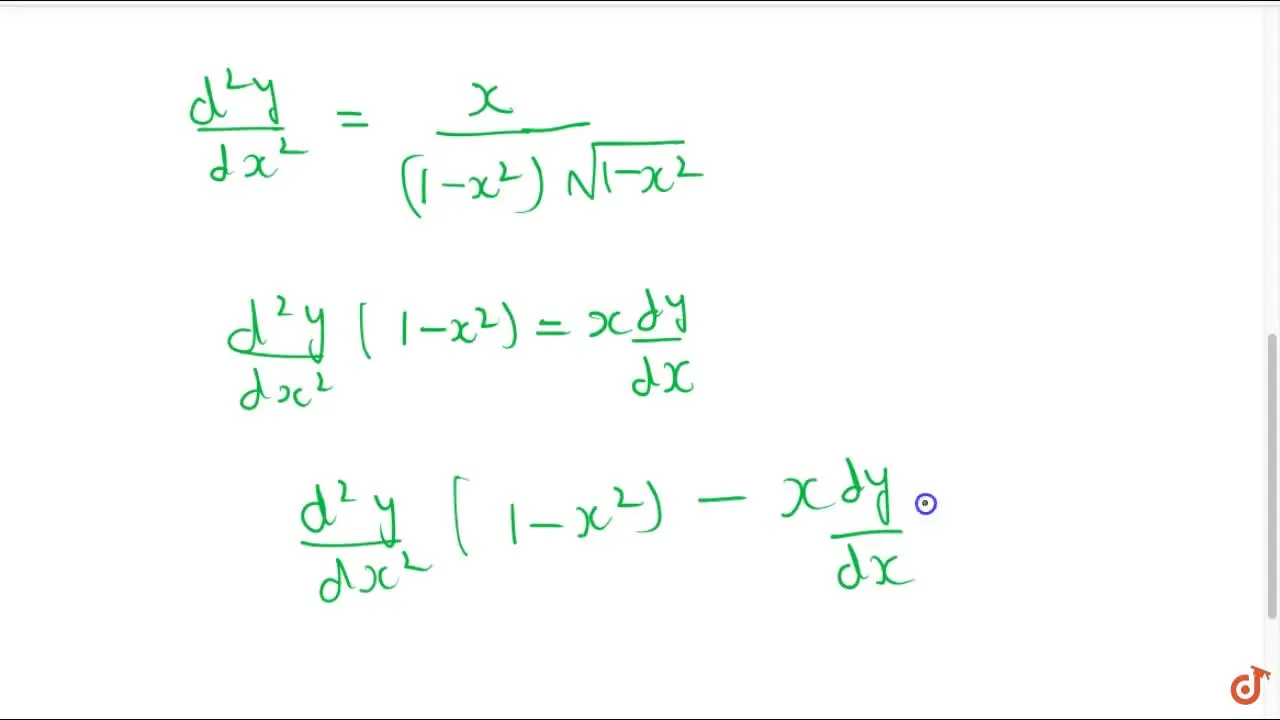If y=sin−1x , then show that (1−x2) d2ydx2−xdydx=0 .
Video Solution
Text Solution
We have given
y=sin−1x
Then proof that (1−x2) d2ydx2−xdydx=0
As, y=sin−1x
Differentiating w.r.t.x
dydx=d(sin−1x)dx
dydx=1√1−x2
(√1−x2)(dydx)=1
((√1−x2)(dydx))2=12
(1−x2)(dydx)2=1
Again Differentiating w.r.t.x
ddx((1−x2)(dydx)2)=d(1)dx
ddx((1−x2)(dydx)2)+(1−x2)ddx(dydx)2))=0
−2x(dydx)2)+(1−x2)2(dydx)×(d2ydx2)=0
⇒(1−x2) d2ydx2−xdydx=0
Hence Proved.
|
Updated on:21/07/2023
Related Playlists
Similar Questions
If y=sin−1x , then show that (1−x2) d2ydx2−xdydx=0 .
View SolutionIf y=sin−1x , show that (1−x2) d2ydx2−xdydx=0 .
View SolutionIf y=asin−1x, then show that :
(1−x2)d2ydx2−xdydx=0View SolutionIf y=sin−1xthen prove that (1−x2)dydx2−xdydx=0
View SolutionIf y=sin−1x, show that (1−x2)d2ydx2−xdydx=0.
View SolutionIf y=cos−1x, then show that :
(1−x2)d2ydx2−xdydx=0View SolutionIf y=(sin−1x)2 then prove that (1−x2)d2ydx2−xdydx−2=0.
View SolutionIf y=sin−1x ,then prove that (1−x2)d2ydx2=x(dydx)
View SolutionIf y=sin−1x, show that (1−x2)d2ydx2−xdydx=0.
View SolutionIf y=sin−1x, prove that (1−x2)d2ydx2−xdydx=0
View Solution