Question

Without expanding, show
that the value of each of the following determinants is zero:
$\left|491639742623\right|$
(ii) $|0xy-x0z-y-z0|$
(iii) $\left|143673543172\right|$

Answer

a

Explanation

(i)

\begin{equation}
\begin{aligned}
&\Delta=\left|\begin{array}{ccc}
49 & 1 & 6 \\
39 & 7 & 4 \\
26 & 2 & 3
\end{array}\right| \\ \\
&=\left|\begin{array}{ccc}
1 & 1 & 6 \\
7 & 7 & 4 \\
2 & 2 & 3
\end{array}\right|\left[\text { Applying } C_{1} \rightarrow C_{1}-8 C_{3}\right] \\
&\Rightarrow \Delta=0
\end{aligned}
\end{equation}




(ii)

$$ \begin{aligned} \Delta &=\left|\begin{array}{ccc} 0 & \mathrm{x} & \mathrm{y} \\ -\mathrm{x} & 0 & \mathrm{z} \\ -\mathrm{y} & -\mathrm{z} & 0 \end{array}\right| \\ \\ &=\frac{\mathrm{xyz}}{\mathrm{xyz}}\left|\begin{array}{ccc} 0 & \mathrm{x} & \mathrm{y} \\ -\mathrm{x} & 0 & \mathrm{z} \\ -\mathrm{y} & -\mathrm{z} & 0 \end{array}\right| \\ \end{aligned} $$
$$ \begin{aligned} &R_{1} \rightarrow R_{1} \times z, R_{2} \rightarrow R_{2} \times y \text { and } R_{3} \rightarrow R_{3} \times x \\ \\ &=\frac{1}{x y z}\left|\begin{array}{ccc} 0 & x z & y z \\ -x y & 0 & z y \\ -y x & -z x & 0 \end{array}\right| \end{aligned} $$
Applying `R_{1} \rightarrow R_{1}+R_{2}+R_{3}`
$$ =\frac{1}{x y z}\left|\begin{array}{ccc} -2 x y & 0 & 2 y z \\ -x y & 0 & z y \\ -y x & -z x & 0 \end{array}\right| $$
Applying `R_{1} \rightarrow R_{1}-2 R_{2}`
$$ =\frac{1}{x y z}\left|\begin{array}{ccc} 0 & 0 & 0 \\ -x y & 0 & z y \\ -y x & -z x & 0 \end{array}\right| $$
$$=0$$





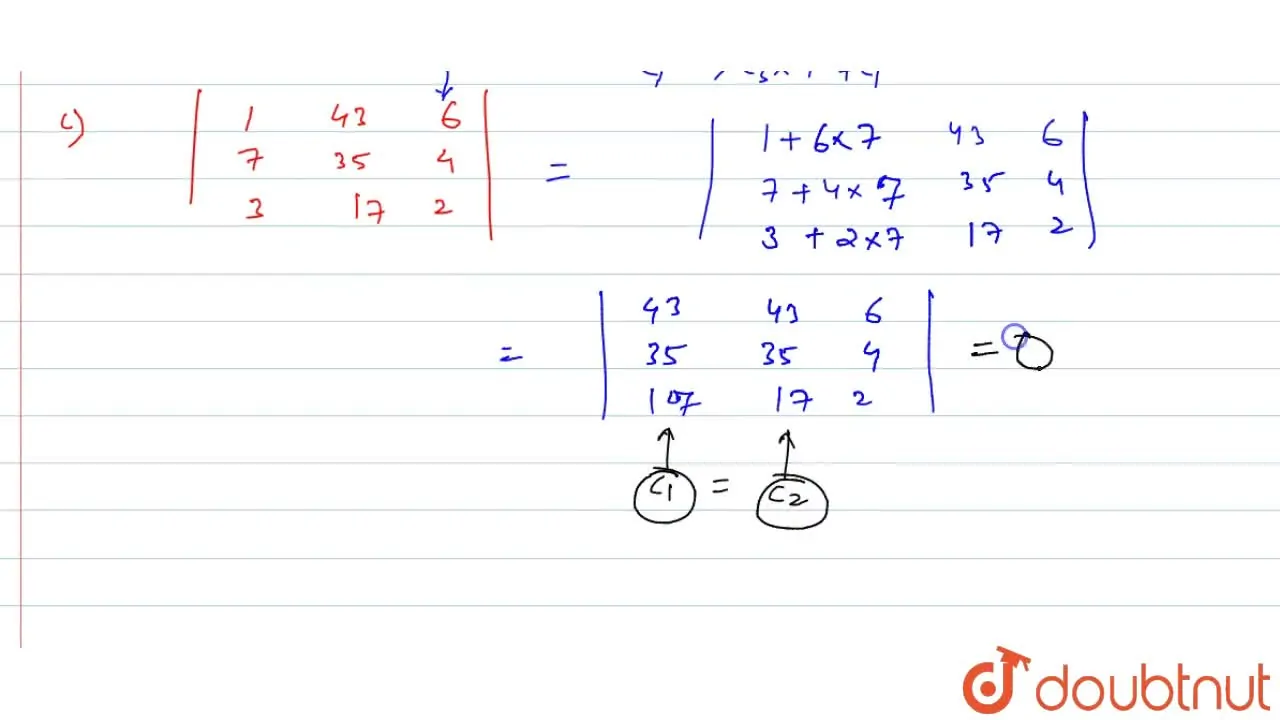
(iii)

$$ \text{Let} \Delta=\left|\begin{array}{lll}1 & 43 & 6 \\ 7 & 35 & 4 \\ 3 & 17 & 2\end{array}\right|$$
Applying `C_{2} \rightarrow C_{2}-7 C_{3}`
$$ \Rightarrow \Delta=\left|\begin{array}{lll} 1 & 1 & 6 \\ 7 & 7 & 4 \\ 3 & 3 & 2 \end{array}\right| $$
As,
$$ C_{1}=C_{2} $$
Hence determinant is zero.