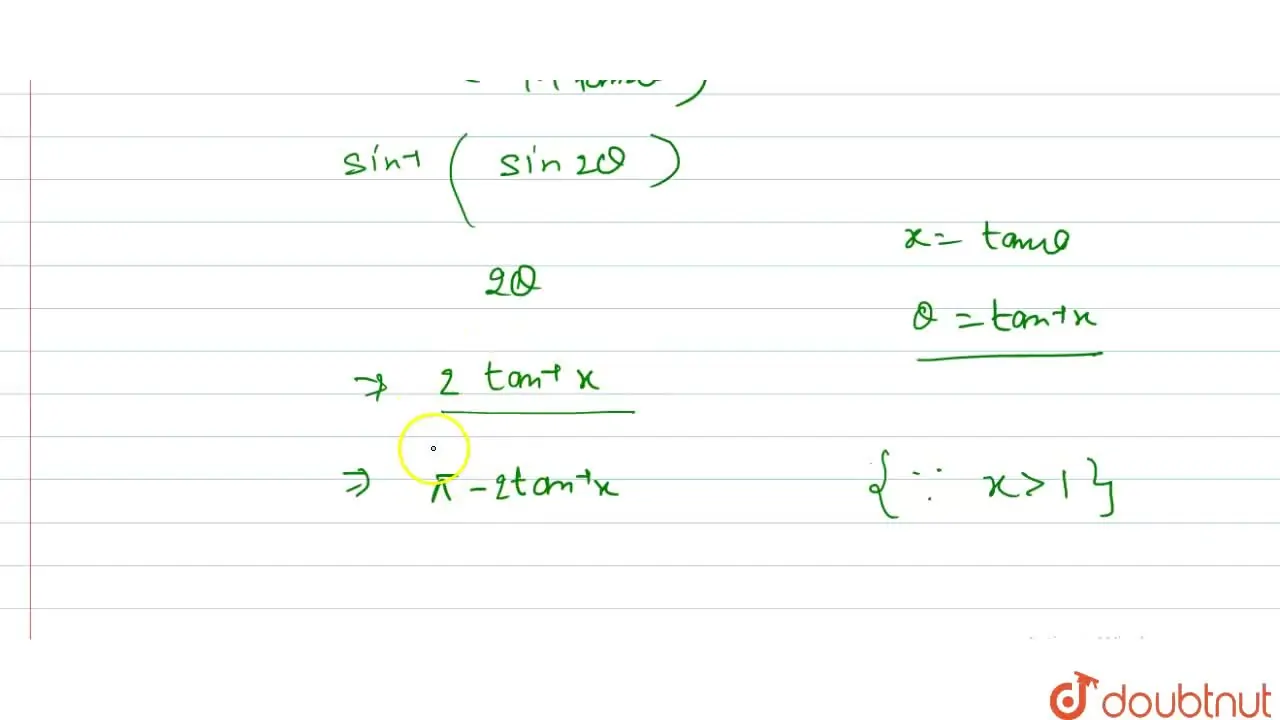If x>1 , then write the value of sin−1(2x1+x2) in terms of tan−1x
Video Solution
Text Solution
sin−1(2x1+x2) an d let x=tanθ
sin−1(2tanθ1+tanθ)
sin−1(sin2θ)
2θ
Let x=tanθ and θ=tan−1x
2tan−1x⇒π−2tan−1x
|
Updated on:21/07/2023
Related Playlists
Similar Questions
If x<0 , then write the value of cos−1(1−x21+x2) in terms of tan−1x
View SolutionIf tan−1x=θ, find the value of sin−1.2x1+x2
View SolutionWrite the value of tan−1(1x) for x<0 in terms of cot−1(x) .
View SolutionDefine: tan−1(3x−x31−3x2) in terms of tan−1x
View SolutionIf (x−1)(x2+1)>0, then find the value of sin(12tan−1.2x1−x2−tan−1x)
View SolutionIf Atan−1x,x∈R then the value of sin 2A is
View SolutionDifferentiate sin−1(2x1+2x) wrt to tan−1x,−1<x<1.
View SolutionIf |x|≤1, then 2tan−1x+sin−1(2x1+x2) is equal to
View SolutionIf: A=tan−1x, then: sin 2A=
View SolutionThe value of sin(2tan−1x),|x|≤1 is
View Solution