
A steel wire of length 60 cm and area of cross section 10−6m2 is joined with a n aluminium wire of length 45 cm and are of cross section 3×10−6m2. The composite string is stretched by a tension of 80 N. Density of steel is 7800kgm−3 and that of aluminium is 2600kgm−3 the minimum frequency of tuning fork. Which can produce standing wave in it with node at joint is
357.2 Hz
275.3 Hz
337.5 Hz
325.5 Hz
The correct Answer is:C
f1=f2(m→ no. of loops in steel wire n→ no. ofn loops in aluminimum wire)
⇒m2L1√Tρ1A1=n2L2√Tρ2A2
⇒m2×60√807800×10−6=n2×45√802600×3×10−6
⇒mn=43
∴f=m2L1√Tρ1A1=42×0.6√807800×10−6
337.5Hz.
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-02)
19 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)
31 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-3(Example)
32 Videos
- SEMICONDUCTORSBOOK - ALLENCHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
51 Videos
Similar Questions
A steel wire of length 5.0 m and cross-section 3.0×10−5m2 stretches by the same amount as a copper wire of length 3.0 m and cross -section 4.0×10−5m2 under a given load. What is the ratio of Young's modulus of steel to that of copper?
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
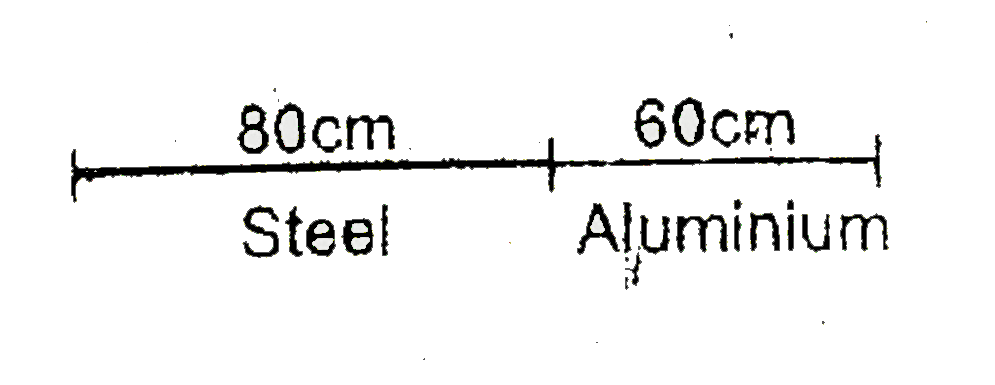
View Solution
Knowledge Check

A steel wire of length 60 cm and area of cross section 10−6m2 is joined with a n aluminimum wire of length 45 cm and are of cross section 3×10−6m2. The composite string is stretched by a tension of 80 N. Density of steel is 7800kgm−3 and that of aluminimum is 2600kgm−3 the minimum frequency of tuning fork. Which can produce standing wave in it with node at joint isA357.3HzB375.3 HzC337.5 HzD325.3 HzA steel wire of length 2.5 m and area of cross section 1.25mm2, when it is stretched by a force of 40 N and Y for steel is 2×1011N/m2. The extension produced in a steel wire is
A0.4 mmB0.4 cmC4 mmD4 cmA steel wire fo length 5m and cross-sectional area 2×10−6m2 streches by the same amount as a copper wire of length 4m and cross sectional area of 3×10−6m2 under a given load. The ratio of young's mouduls of steel to that of copper is
A8:15B15:8C5:3D3:5
Figure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is .1.0mm2 and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is 2.6gcm−3and that of steel is 7.8gcm−3
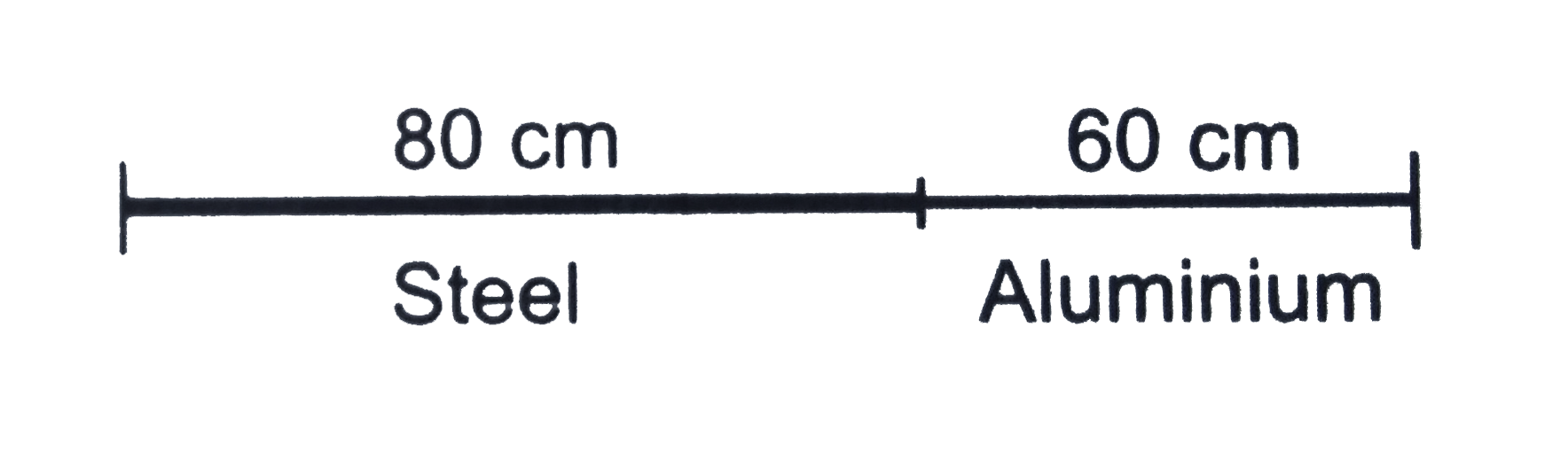
View SolutionA steel wire of length 5 m and area of cross-section 4mm2 is stretched by 2 mm by the application of a force. If young's modulus of steel is 2×1011N/m2, then the energy stored in the wire is
View SolutionA steel wire of length 4.87 mm and cross-section 3.0×10−5m2 stretches by the same amout as a copper wire of length 3.5 m and cross -section 4.0×10−5m2 under a given load . White is the ratio of the Young's modulus of steel so that of copper ?
View SolutionA steel wire of length 4.7m and cross-sectional area 3×10−6m2 stretches by the same amount as a copper wire of length 3.5m and cross-sectional area of 4×10−6m2 under a given load. The ratio of Young's modulus of steel to that of copper is
View SolutionA steel wire of length 4.5 m and cross-sectional area 3×10−5m2 stretches by the same amount as a copper wire of length 3.5 m and cross-sectional area of 1×10−5m2 under a given load. The ratio of the Young's modulus of steel to that of copper is
View Solution
