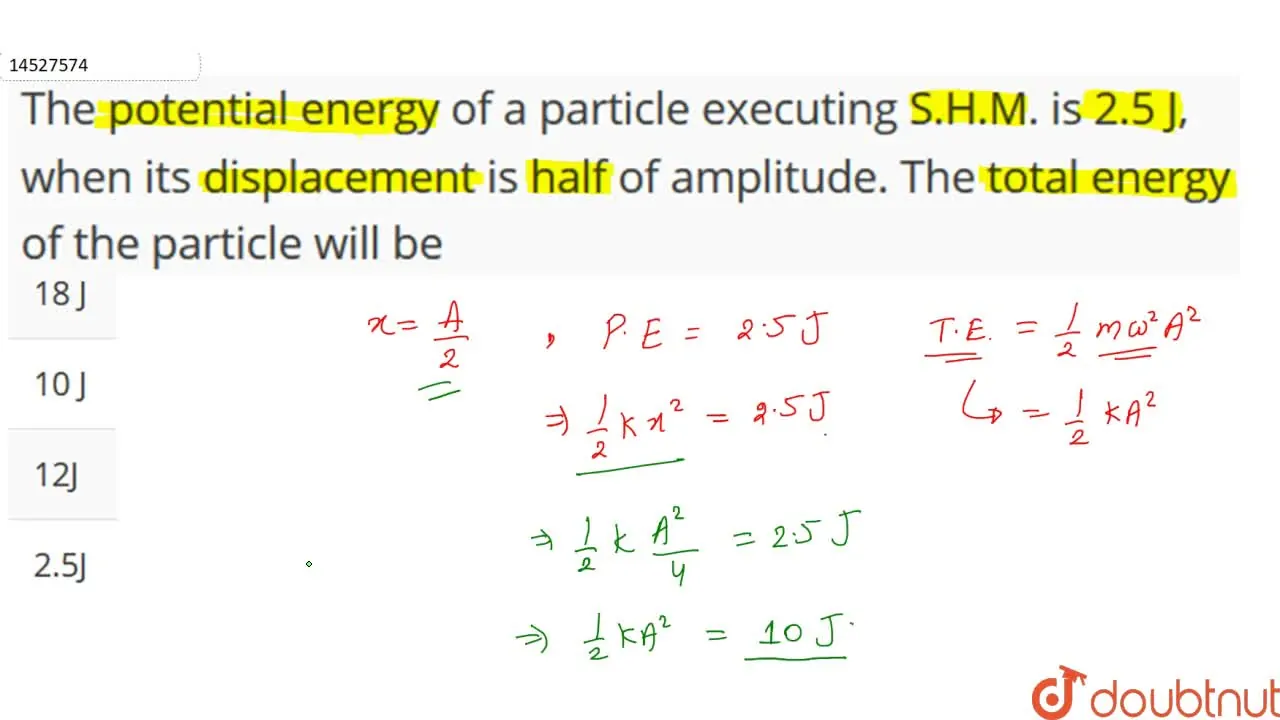
The potential energy of a particle execuring S.H.M. is 2.5 J, when its displacement is half of amplitude. The total energy of the particle be
18 J
10 J
12J
2.5J
The correct Answer is:B
Total energy =12mω2A2
Potential energy=12mω2x2
2.5=12mω2(A2)2
so total energy =10 J
Related Playlists
Similar Questions
The potential energy of a particle executing S H M is 25 J. when its displacement is half of amplitude. The total energy of the particle is
View SolutionThe potential energy of a particle perfonning S.H.M. is
View SolutionPotential energy of the particle performing S.H.M. is
View SolutionThe potential energy of a particle performing S.H.M. at mean position is
View SolutionThe potential energy of a particle performing S.H.M. at mean position is
View SolutionThe total energy of a particle executing S.H.M. is proportional to
View SolutionThe potential energy of a particle performing S.H.M. at extreme position is
View SolutionWhen the displacement is half the amplitude, the ratio of potential energy to the total energy is
View SolutionThe P.E. of a linear harmonic oscillator is 4J, when its displacment is equal to half its amplitude. The total energy of the particle is
View SolutionThe ratio of the kinetic to potential energy of a particle in SHM, when its displacement is one-half the amplitude, is
View Solution