The maximum velocity at the lowest point, so that the string just slack at the highest point in a vertical circle of radius l.
√gl
√3gl
√5gl
√7gl
The correct Answer is:C
To solve the problem of finding the maximum velocity at the lowest point of a vertical circle of radius L such that the string just slackens at the highest point, we can follow these steps:
Step 1: Understand the Energy Conservation
At the lowest point of the vertical circle, the object has maximum kinetic energy and minimum potential energy. At the highest point, the object has minimum kinetic energy and maximum potential energy. We will use the principle of conservation of mechanical energy.
Step 2: Write the Energy Equations
Let:
- m = mass of the object
- vL = velocity at the lowest point
- vH = velocity at the highest point
- g = acceleration due to gravity
At the lowest point (point A):
- Kinetic Energy (KE) = 12mv2L
- Potential Energy (PE) = 0 (taking this point as reference)
At the highest point (point B):
- Kinetic Energy (KE) = 12mv2H
- Potential Energy (PE) = mg(2L) (since the height is 2L)
Using conservation of energy:
12mv2L=12mv2H+mg(2L)
Step 3: Simplify the Energy Equation
Cancel m from the equation:
12v2L=12v2H+2gL
Multiply through by 2:
v2L=v2H+4gL
Step 4: Analyze Forces at the Highest Point
At the highest point, for the string to just slack, the tension in the string must be zero. The only forces acting on the mass at the highest point are the gravitational force and the centripetal force required to keep it moving in a circle.
The equation for the forces at the highest point (where tension T=0) is:
mg=mv2HL
Cancel m:
g=v2HL
Rearranging gives:
v2H=gL
Step 5: Substitute v2H into the Energy Equation
Now, substitute v2H back into the energy equation:
v2L=gL+4gL
v2L=5gL
Step 6: Solve for vL
Taking the square root:
vL=√5gL
Conclusion
Thus, the maximum velocity at the lowest point so that the string just slackens at the highest point is:
vL=√5gL
At the lowest point of the vertical circle, the object has maximum kinetic energy and minimum potential energy. At the highest point, the object has minimum kinetic energy and maximum potential energy. We will use the principle of conservation of mechanical energy.
Let:
- m = mass of the object
- vL = velocity at the lowest point
- vH = velocity at the highest point
- g = acceleration due to gravity
- Kinetic Energy (KE) = 12mv2L
- Potential Energy (PE) = 0 (taking this point as reference)
- Kinetic Energy (KE) = 12mv2H
- Potential Energy (PE) = mg(2L) (since the height is 2L)
12mv2L=12mv2H+mg(2L)
Cancel m from the equation:
12v2L=12v2H+2gL
v2L=v2H+4gL
At the highest point, for the string to just slack, the tension in the string must be zero. The only forces acting on the mass at the highest point are the gravitational force and the centripetal force required to keep it moving in a circle.
mg=mv2HL
g=v2HL
v2H=gL
Now, substitute v2H back into the energy equation:
v2L=gL+4gL
v2L=5gL
Taking the square root:
vL=√5gL
Thus, the maximum velocity at the lowest point so that the string just slackens at the highest point is:
vL=√5gL
Topper's Solved these Questions
Similar Questions
One end of a string of length 1.5 m is tied to a stone of mass 0.4 kg and the other end to a small pivot on a smooth vertical board. What is the minimum speed of the stone required at its lower most point so that the string does not slack at any point in its motion along the vertical circle ?
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
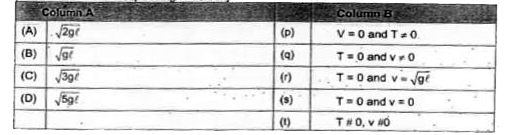
View Solution
Knowledge Check
Assertion For looping a verticla loop of radius, r the minimum velocity at lowest point should be √5gr. Reason In this event the velocityh at the highest point will be zero.
ABoth assertion and reson are true and reason is the correct explanation of assertionBBoth assetion and reason are true but reason is not the correct explanation of assertionCAssertion is true but reason is falseDBoth assetion and reason are flaseA particle is projected so as to just move along a vertical circle of radius r. The ratio of the tension in the string when the particle is at the lowest and highest point on the circle is -
A1Bfinite but largeCzeroDInfiniteA horizontal cylinder is fixed, its inner surface is smooth and its radius is R. A small block is initially at the lowest point. The minimum velocity that should by given to the block at the lowest point, so that it can just cross the point P is u then
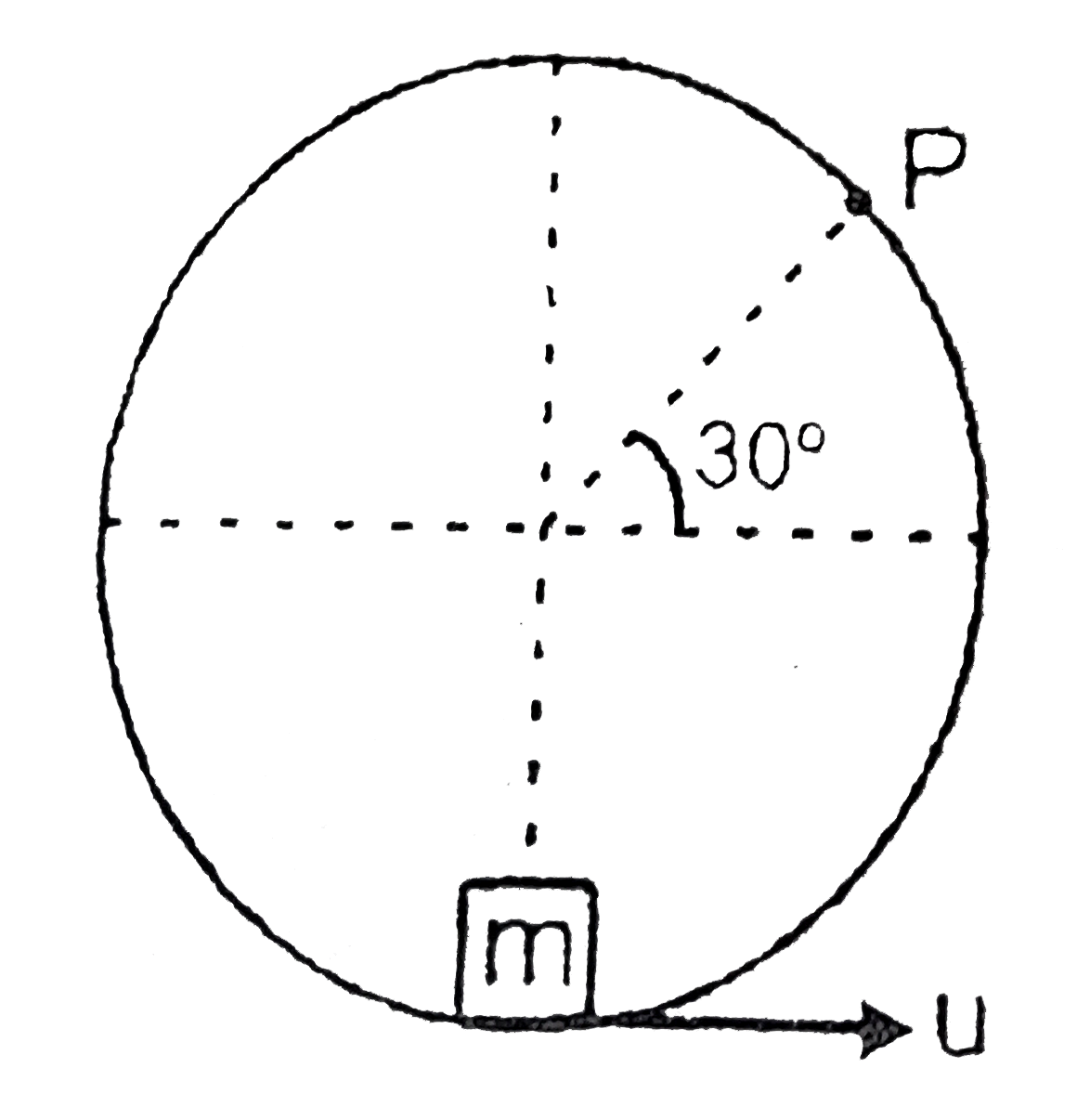 AIf the block moves anti clockwise then u=√3.5gRBIf the block moves anti clockwise then u=√3gRCIf the block moves clockwise then u=√3.5gRDIf the block moves clockwise then u=√5gR
AIf the block moves anti clockwise then u=√3.5gRBIf the block moves anti clockwise then u=√3gRCIf the block moves clockwise then u=√3.5gRDIf the block moves clockwise then u=√5gR
A stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point .
View SolutionA body of mass 0.4 kg is tied to one end of a string and the other end of the string is tied to a small pivot on a vertical wall . Calculate the minimum speed of the body required at its lower most pint to avoid slacking of string at any point in its motion along the vertical circle of radius 1 m .
View SolutionA stone is tied to one end of a string and rotated in a vertical circle . What is the difference in tensions of the string at lowest and highest points of the vertical circle ?
View SolutionA stone of mass 0.4 kg is tied to a string and rotated in a vertical circle of radius 1.2 m . Calculate the speed of the stone for which the tension in the string is zero at the highest point of the circle . What is the tension at the lowest point in this case ?
View SolutionA particle P is attached to two fixed points O1 and O2 in a horizontal line, by means of two ight inextensible strings of equal length l. It is projected with a velocity just sufficient to make it describe a circle, in a vertical plane, without the strings getting slack and with the angle<O2O1P=<O1O2P=θ. When the particle is at its lowest point, the string O2P breaks and the subsequent path of the particle was found to be a circle of radius l cosθ. Find θ.
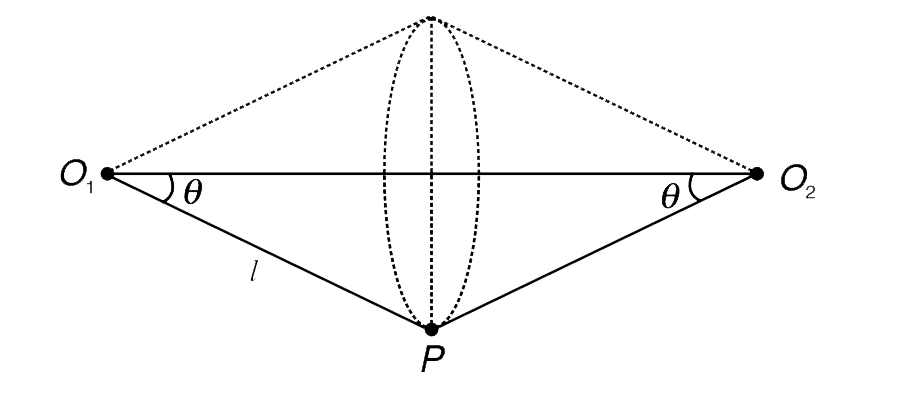
View Solution
