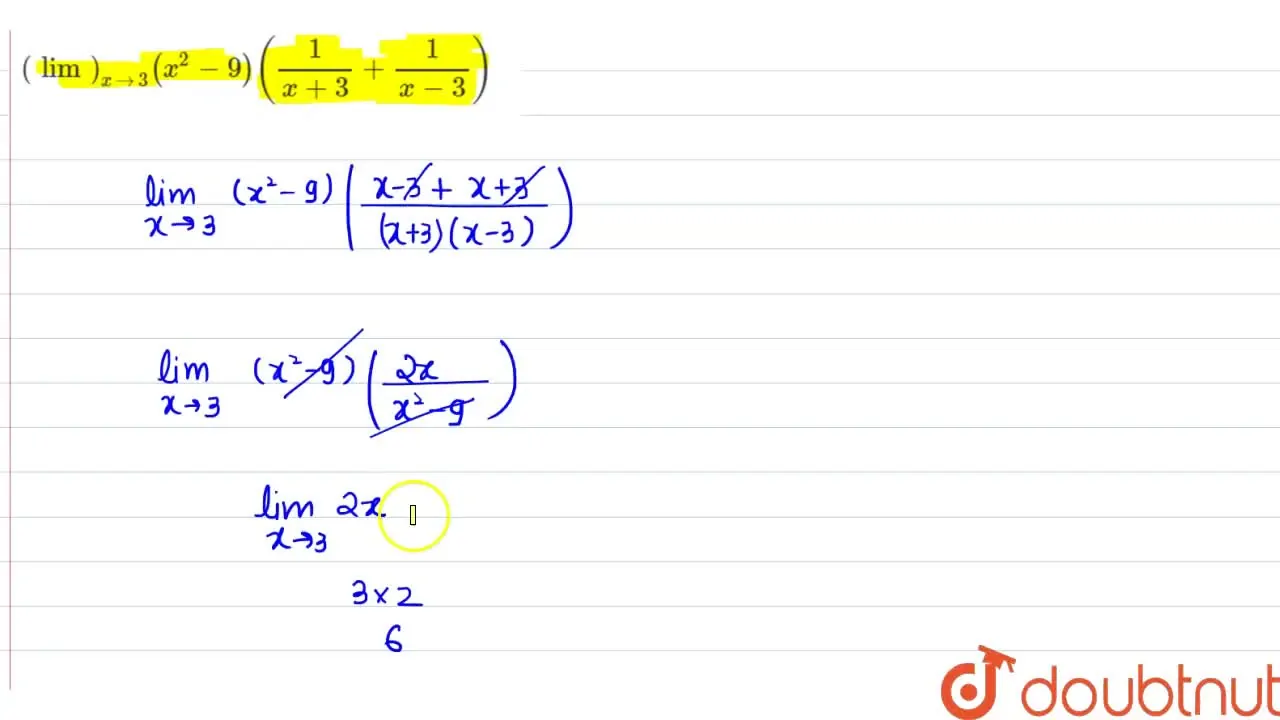
Evaluate the following limit: limx→3(x2−9)(1x+3+1x−3)
Video Solution
Text Solution
Given,
(lim)x→3(x2−9)(1x+3+1x−3)
=(lim)x→3(x2−9)x−3+x+3(x+3)(x−3)
=(lim)x→3(x2−9)2xx2−9
=(lim)x→3(2×3)
=6
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Evaluate the following limit: limx→2(3−x)
View SolutionEvaluate the following limits :
limx→1(3x+1)(x+1)View SolutionEvaluate the following limits :
limx→139x2−13x−1View SolutionEvaluate the following limit: limx→3x4−81x2−9
View SolutionEvaluate the following limits :
limx→1{2(x+3)+7}View SolutionEvaluate the following limit: limx→3(x2−9)(1x+3+1x−3)
View SolutionEvaluate the following limits :
limx→2x2−x−2x2−3x+2View SolutionEvaluate the following limit: limx→1(1x2+x−2−xx3−1)
View SolutionEvaluate the following limit: limx→03x+1x+3
View SolutionEvaluate the following limit: limx→3(1x−3−3x2−3x)
View Solution