The sequence N of natural numbers is divided into classes as follows. Show that the sum of the numbers in nth row is (2n2+1)
The number of numbers in successive row are 2,4,6 and hence there will be 2n numbers in nth row which will be in the AP of cd 1. The first term in successive row are
S=1+3+7+11+....+tn
S=13+7+11+...+tn−1=tn
Sutracting O=1+[2+4+6+(n−1)]terms−tn
∴Sn=sum of an AP in which
A=n2−n+1,D=1,N=2n
Sn=2π2[2(n−n+1)(2n−1).1]
n(2n2+1)
Topper's Solved these Questions
Similar Questions
The sequence of odd natural numbers is divided into groups 1,3,5,7,9,11,…. and so on. Show that the sum of the numbers in nth group is n3.
View SolutionThe series of natural numbers is divided into groups (1),(2,3,4):(5,6,7,8,9) and so on . Show the sum of the numbers in the nth group is (n−1)3+n3
View Solution
Knowledge Check
The sum of first 30 even natural numbers is
A930B950C910D980The natural numbers are written as follows :
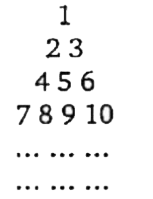
The sum of numbers in the nth row is :Aa. n(n+1)2Bb. n2+12Cc. n(n2+1)2Dd. none of theseThe natural numbers are written as follows :
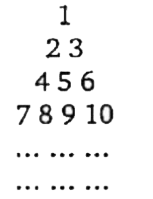
The sum of numbers in the nth row is :An(n+1)2Bn2+12Cn(n2+1)2Dnone
84. The natu The natural numbers are written as follows 2 3 7 8 9 10 th The sum of the numbers in the n" row is (A) (n2-1)
View SolutionSum of square of m natural numbers
View SolutionThe series of natural numbers is divided into groups :
1, (2, 3, 4), (5, 6, 7, 8, 9),…… and so on. Then the sum of the numbers in the nth group is
View SolutionNatural numbers are divided into groups in the following way: 1,(2,3),(4,5,6),(7,8,9,10), Show that the sum of the numbers in the nth group is n(n2+1)2
View SolutionIf the sequence of even natural numbers is 2, 4, 6, 8, …, then what is the nth term of the sequence ?
View Solution
