Find two numbers whose arithmetic mean is $34$ and the geometric mean is $16$ .

Video Solution

Text Solution

Verified by Experts

Given, arithmetic mean is $34$ and the geometric mean is $16$ .

$A M = 34$

$\frac{a + b}{2} = 34$

$a + b = 68$ $(1)$

$G M = 16$

$\sqrt{a b} = 16$

$a b = 256$

We know,

$a - b = \sqrt{{(a + b)}^{2} - 4 a b}$

$a - b = \sqrt{{(68)}^{2} - 4 \cdot 256}$

$a - b = \sqrt{3600} = 60$ $(2)$

Adding $(1)$ and $(2)$

$2 a = 128$

$a = 64$

$a + b = 68$

$b = 68 - 64 = 4$

|

Updated on:21/07/2023

Class 11 MATHS SEQUENCES AND SERIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The harmonic mean of two positive numbers a and b is 4, their arithmetic mean is A and the geometric mean is G. If $2 A + G^{2} = 27, a + b = α$ and $| a - b | = β$ , then the value of $\frac{α}{β}$ is equal to
A1
B3
C $\frac{5}{2}$
D5
Question 2 - Select One
Find the arithmetic mean of:

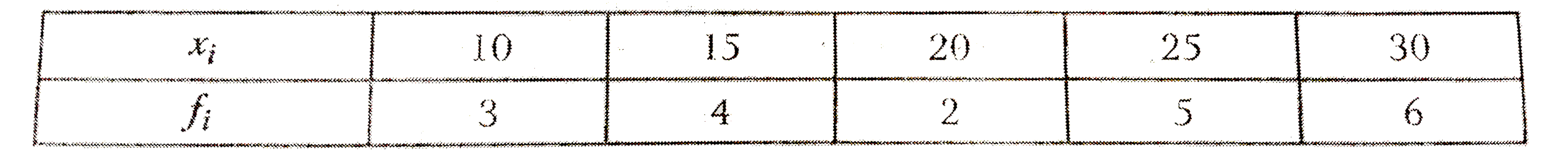A21
B21.4
C22.75
D21.75
Question 3 - Select One
What will be quadratic equation in x when the roots have arithmetic mean A and the geometric mean G?
A $x^{2} + 2 A x + G^{2} = 0$
B $x^{2} + G^{2} x + A = 0$
C $x^{2} - 2 A x + G^{2} = 0$
D $x^{2} + G x + A = 0$

RD SHARMA-GEOMETRIC PROGRESSIONS-Solved Examples And Exercises

Home
Ask Doubt
Profile