Find the sum of the following geometric series: 0. 15+0. 015+0. 0015+ to..... 8 t e r m s
Video Solution
Text Solution
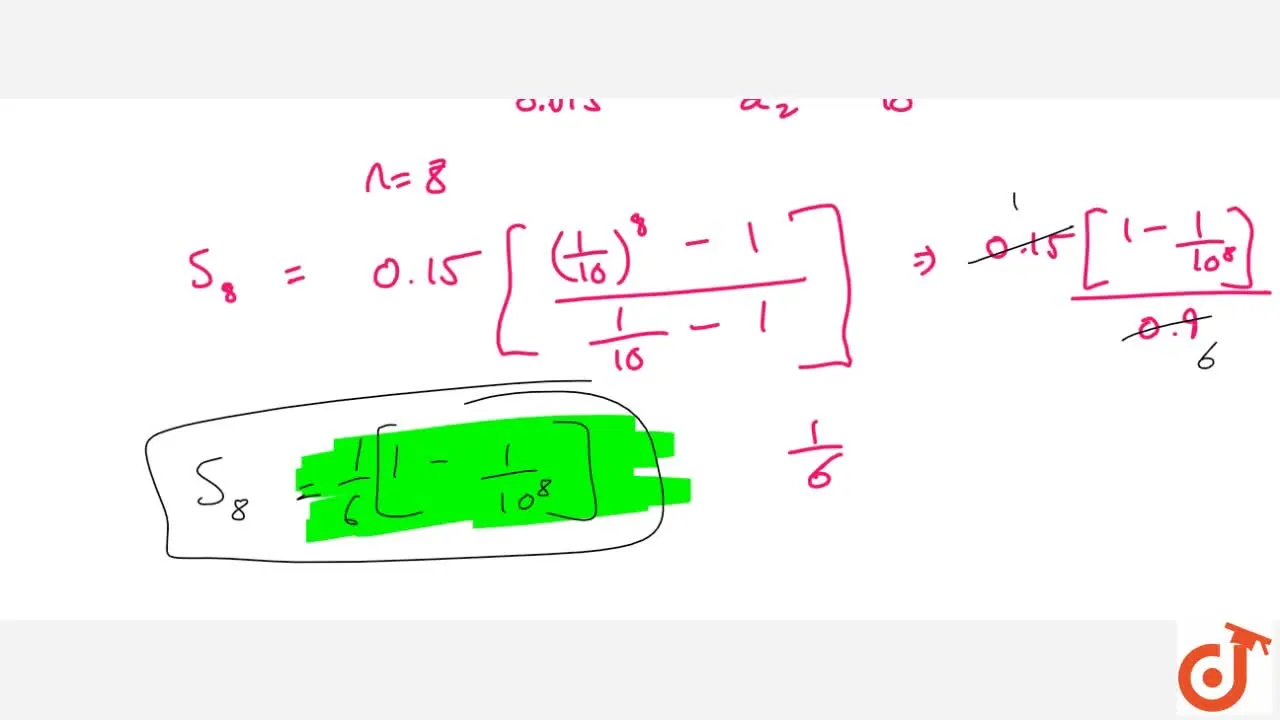
Here, a=0.15 and
r=110.
S8=a(1−r8)1−r
=0.15(1−(110)8)1−110
=16(1−(1108))
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Find the sum of the following geometric series: 0. 15+0. 015+0. 0015+ to..... 8 t e r m s
View SolutionFind the sum of the following series: 0.5+0.55+0.555+→ n terms
View SolutionFind the sum of the following series :
0.6+0.06+0.006+... to 16 termsView SolutionFind the sum of the following series: 0.5+0.55+0.555→ n terms
View SolutionFind the sum of the following series : 0.7+0.77+0.777+→n terms
View SolutionThe sum of the geometric progression 0.15, 0.015, 0.0015,…20 terms is
View SolutionFind the sum in each of the following :
15+0.632+13.8View SolutionFind the sum of the following series ,
(i)0.5+0.05+0.005+0.0005+…
(ii) 0.3+0.18+0.108+…View SolutionFind the sum of 7 terms of the series 2+0.2+0.02+….
View SolutionFind the sum to indicated number of terms in each of the geometric progressions : 0.15, 0.015, 0.0015, . . . , 20 terms.
View Solution