If the different permutations of all the letter of the word EXAMINATION are listed as in a dictionary, how many words are there in this list before the first word starting with E?

Video Solution

Text Solution

Verified by Experts

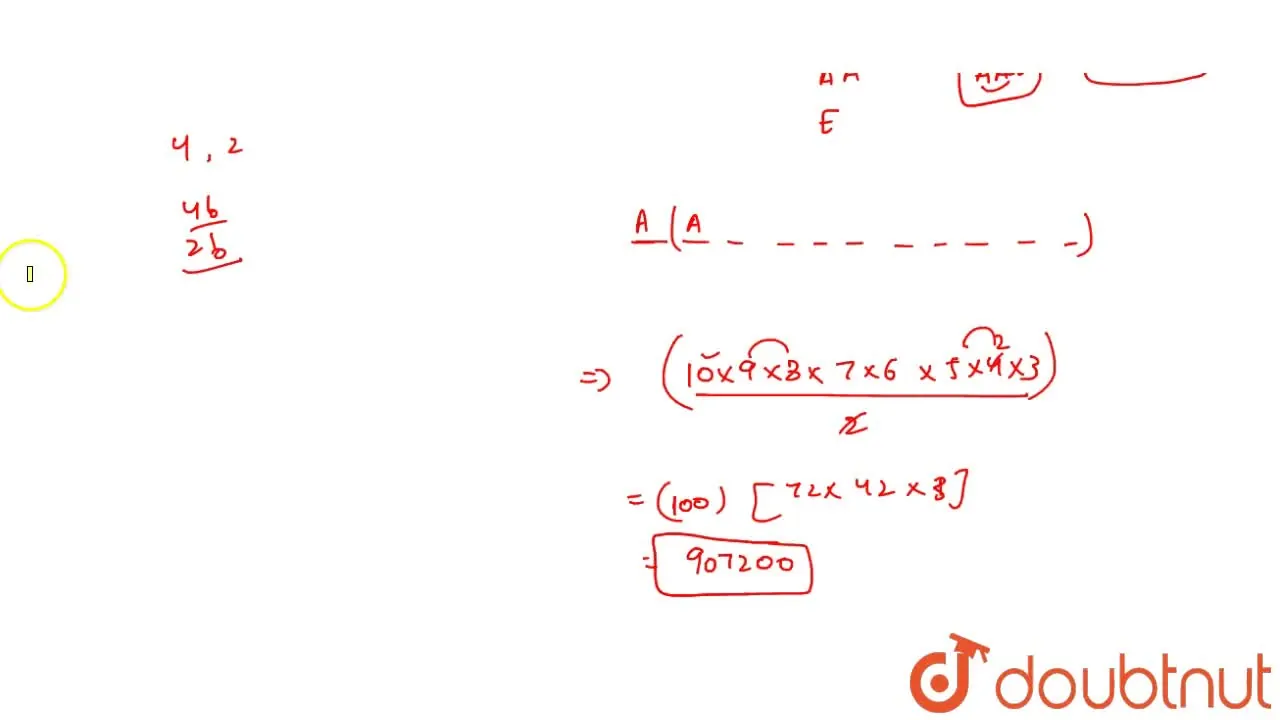
In this problem, we have to find the number of words starting with A.
In EXAMINATION, we have two I's and two N's and all other letters are different.
∴ Number of ways of arrangement = $\frac{10!}{2! 2!}$
= $\frac{10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2!}{2 \times 1 \times 2!}$
= $907200$

Updated on:21/07/2023

If the different permutations of the word EXAMINATION are listed as in a dictinary how many items are there in this list before the first word starting with E?
A $906200$
B $907200$
C $908200$
DNone of these
If all permutations of the letters of the word 'AGAIN' are arranged as in dictionary, then fiftieth word is
ANAAGI
BNAGAI
CNAAIG
DNAIAG
If all permutations of the letters of the word AGAIN are arranged as in dictionary, then fiftieth word is
ANAAGI
BNAGAI
CNAAIG
DNAIAG