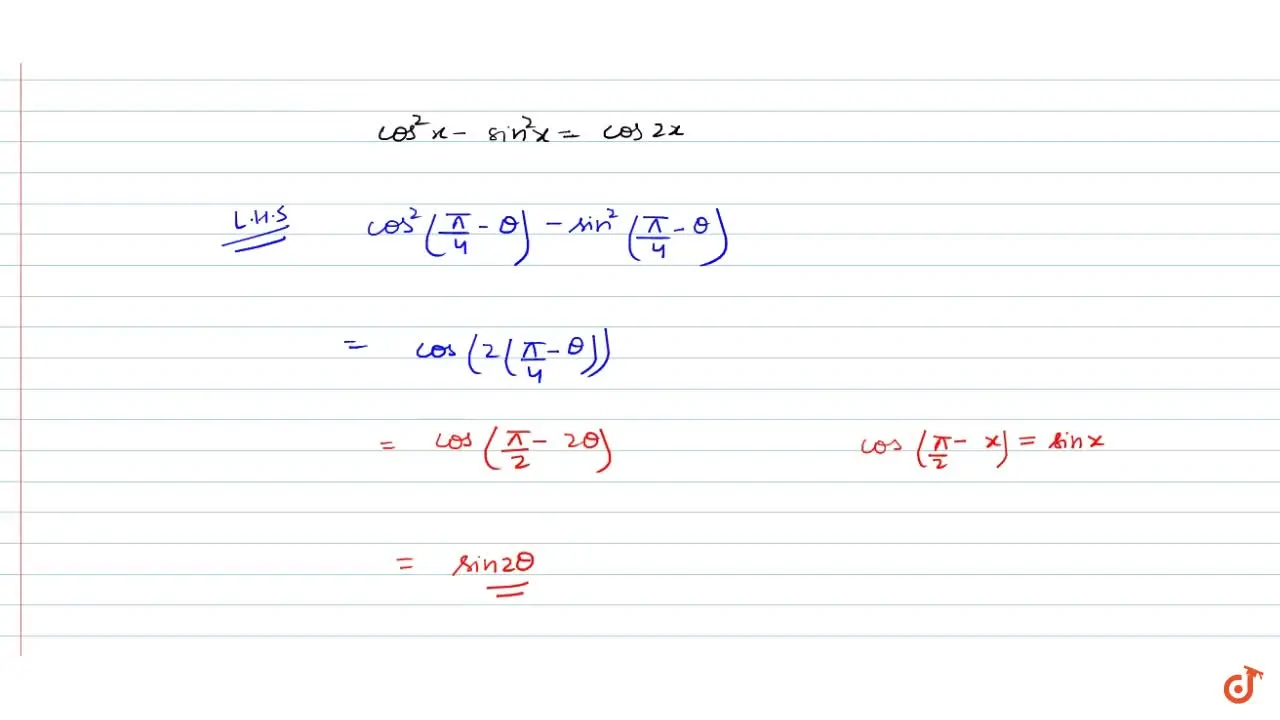Prove that:cos2(π4−θ)−sin2(π4−θ)=s∈2θ
Video Solution
Text Solution
Given,cos2(π4−θ)−sin2(π4−θ)
using,
cos2A−sin2B=cos(A+B).cos(A−B)]
⇒cos{π4+θ+π4−θ}.cos{π4+θ−π4−θ}
⇒cos(2π4).cos2θ
⇒cos(π2).cos2θ
⇒0 [since cos(π2)=0]
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Prove that:cos2(π4−θ)−sin2(π4−θ)=s∈2θ
View SolutionProve that cos4θ−sin4θcos2θ−sin2θ=1
View SolutionFind cos(2π+θ)c o s e c(2π+θ)tan(π/2+θ)sec(π/2+θ)cosθcot(π+θ)
View SolutionThe value of cos2(π4+θ)−sin2(π4−θ) is
View SolutionProve that :
cos4θ−cos2θ=sin4θ−sin2θView Solutioncos2(π4−θ)+cos2(π4+θ)=.....
View SolutionProve that sin4θ−cos4θ=sin2θ−cos2θ
View SolutionProve that sin4θ−cos4θ=sin2θ−cos2θ.
View SolutionProve that sin4θ−cos4θsin2θ−cos2θ=1
View SolutionProve that: tan(π4+θ)+tan(π4−θ)=2sec2θ .
View Solution