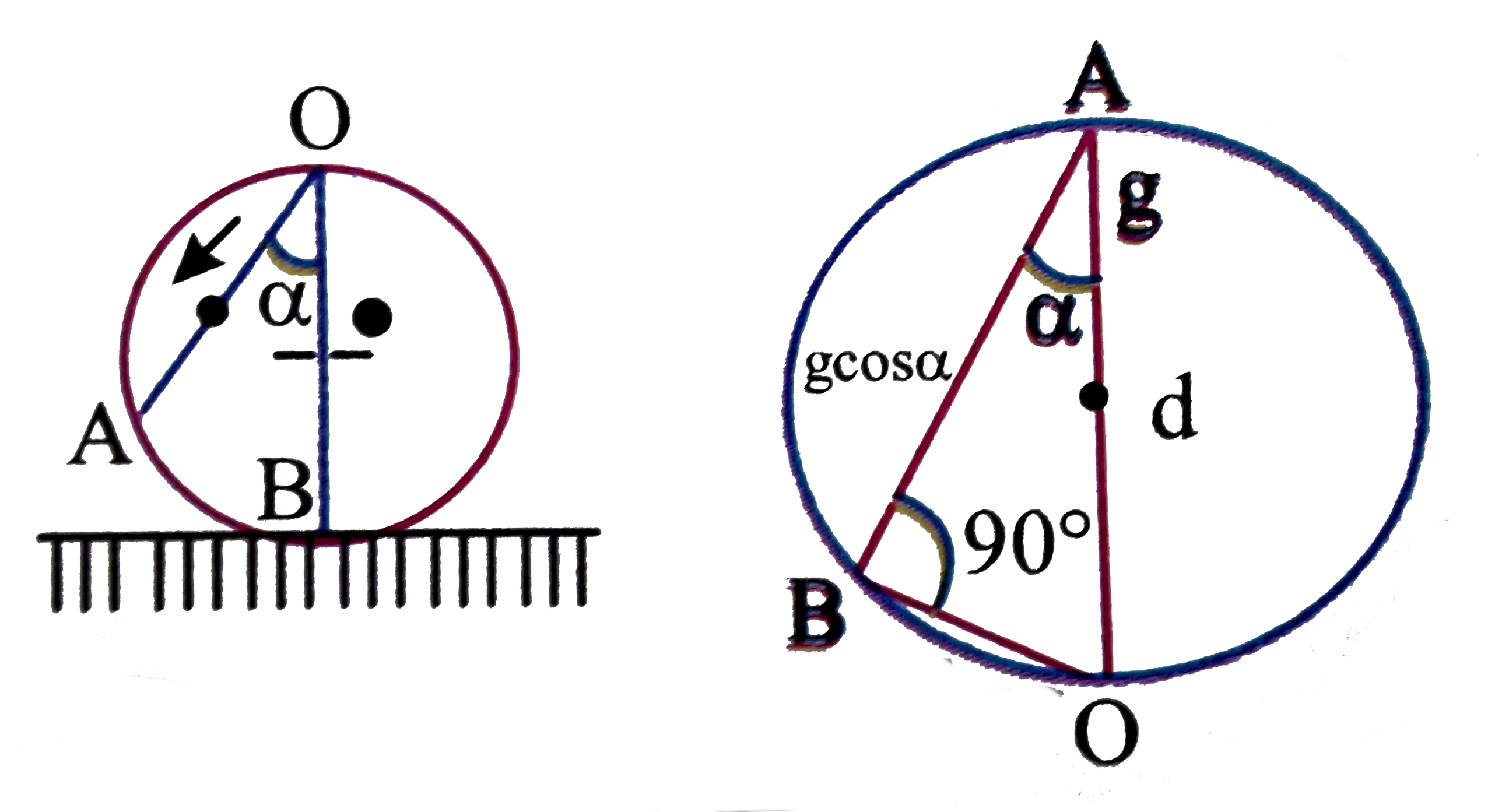
A frictionless wire is fixed between A and B inside a circle of radius R. A small bead slips along the wire. Find the time taken by the bead to slip from A to B.
2√R/g
gR/√gcosθ
2√gRgcosθ
2√gRcosθg
The correct Answer is:A
AB=2Rcosθ
acceleration along AB
a=gcosθ
u=0 from A to B

2Rcosθ=0+12(gcosθ)t2
t=2√Rg
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.16
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.17
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.14
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
21 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A bead is free to slide down on a smooth wire rightly stretched between points AandB on a vertical circle of radius 10m. Find the time (in sec) taken by the bead to reach point B, if the bead slides from rest from the highest point A on the circle.
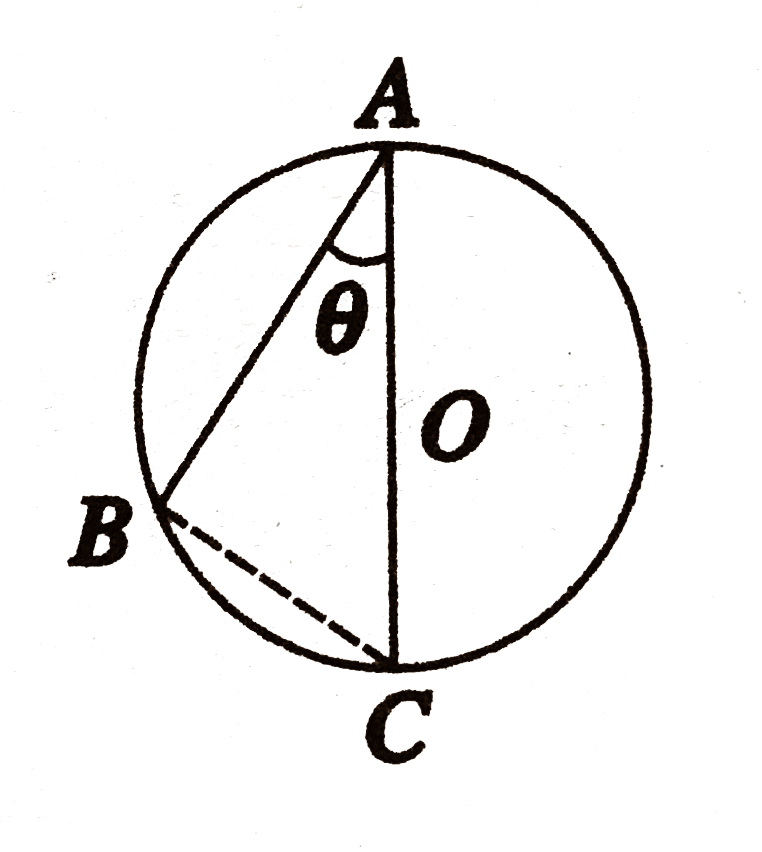 .
.
View SolutionA thin insulating wire is stretched·along the diameter of an. insulated circular hoop of radius R. A small bead of mass m and charge-q is threaded onto the wire. Two small identical charges are tied to the hoop at points opposite to each other, so that the diameter passing through them is perpendicular to the thread as shown in figure. The bead is released at a point which.is located at a distance x0 from the centre of the hoop. Assume that x0<<R.
(a) What is the resultant force acting on the charged bead?
(b) Describe the motion of the bead after it is released X
(c) Use the assumption that xR<<1 to obtain an approximate equation of motion, and find the displacement and velocity of the bead as functions of time
(d) When will the velocity of the bead will bcome zero for the first time?

View Solution
Knowledge Check
A friction wire AB is fixed on a sphere of radius R. A very small spherical ball slips on this wire. The time taken by the ball to slip from A to B.
 A√2gRgcosθB2√gR.cosθgC2√RgDgR√gcosθ
A√2gRgcosθB2√gR.cosθgC2√RgDgR√gcosθA friction wire AB is fixed on a sphere of radius R. A very small spherical ball slips on this wire. The time taken by the ball to slip from A to B.
 A2√gRgcosθB2√gRcosθgC2√RgDgR√gcosθ
A2√gRgcosθB2√gRcosθgC2√RgDgR√gcosθA frictionless wire is fixed between A and B inside of a hollow sphere of radius R as shown. A beam slips along the wire starting from the rest at point A. The time taken by the beam to slip from A to B will be
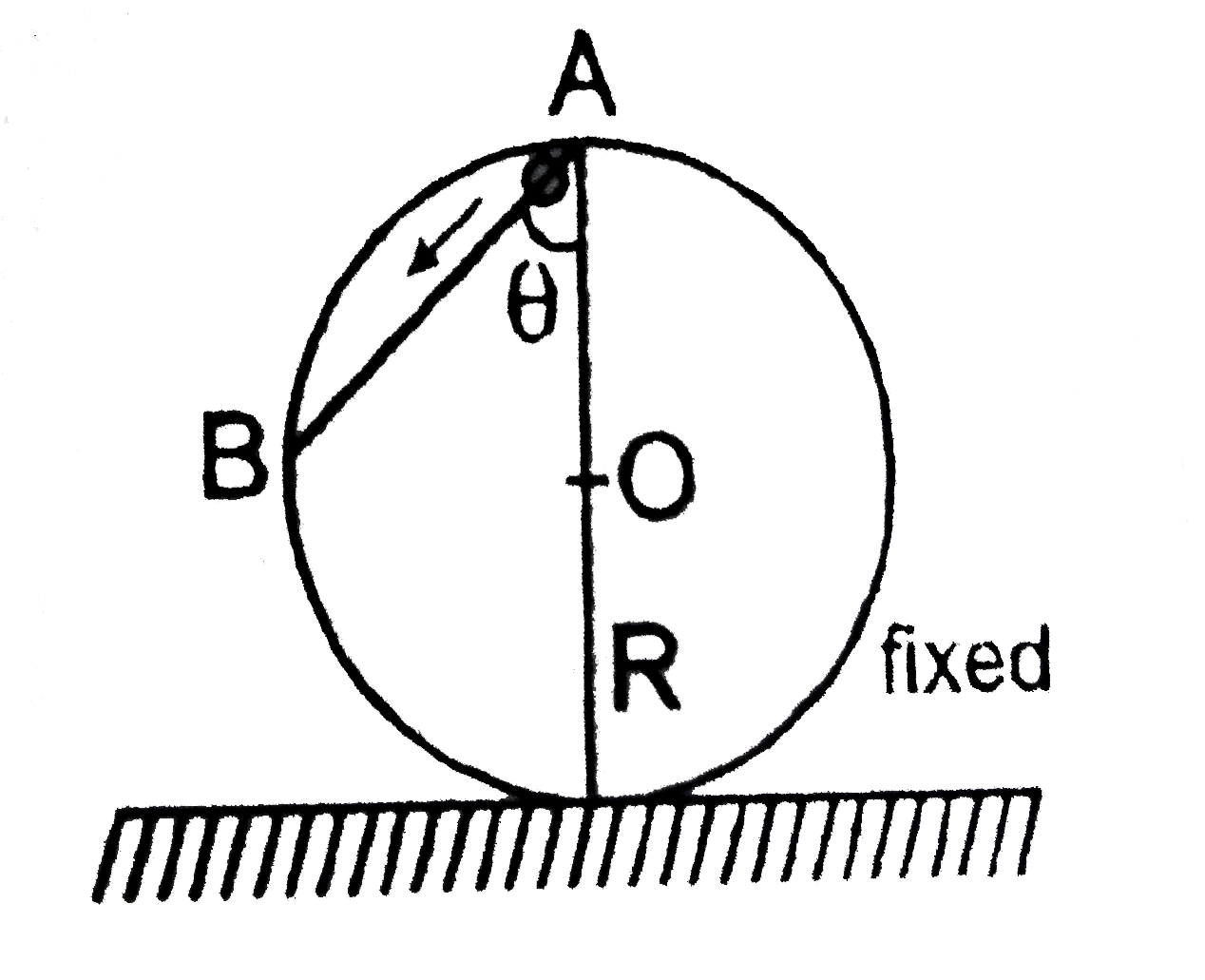 A2√R/gBgR√gcosθC2√gRgcosθD2√gRcosθg
A2√R/gBgR√gcosθC2√gRgcosθD2√gRcosθg
A thin insulating wire is stretched along the diameter of an insulated circular hoop of radius E. A small bead of mass m and charge –q is threaded onto the wire. Two small identical charge= are tied to the hoop at points opposite to each other, so that the diameter passing throughtheL-is perpendicular to the thread (see figure). The bead is released at a point which is a distance-, from the centre of the hoop. Assume that x0<<R.
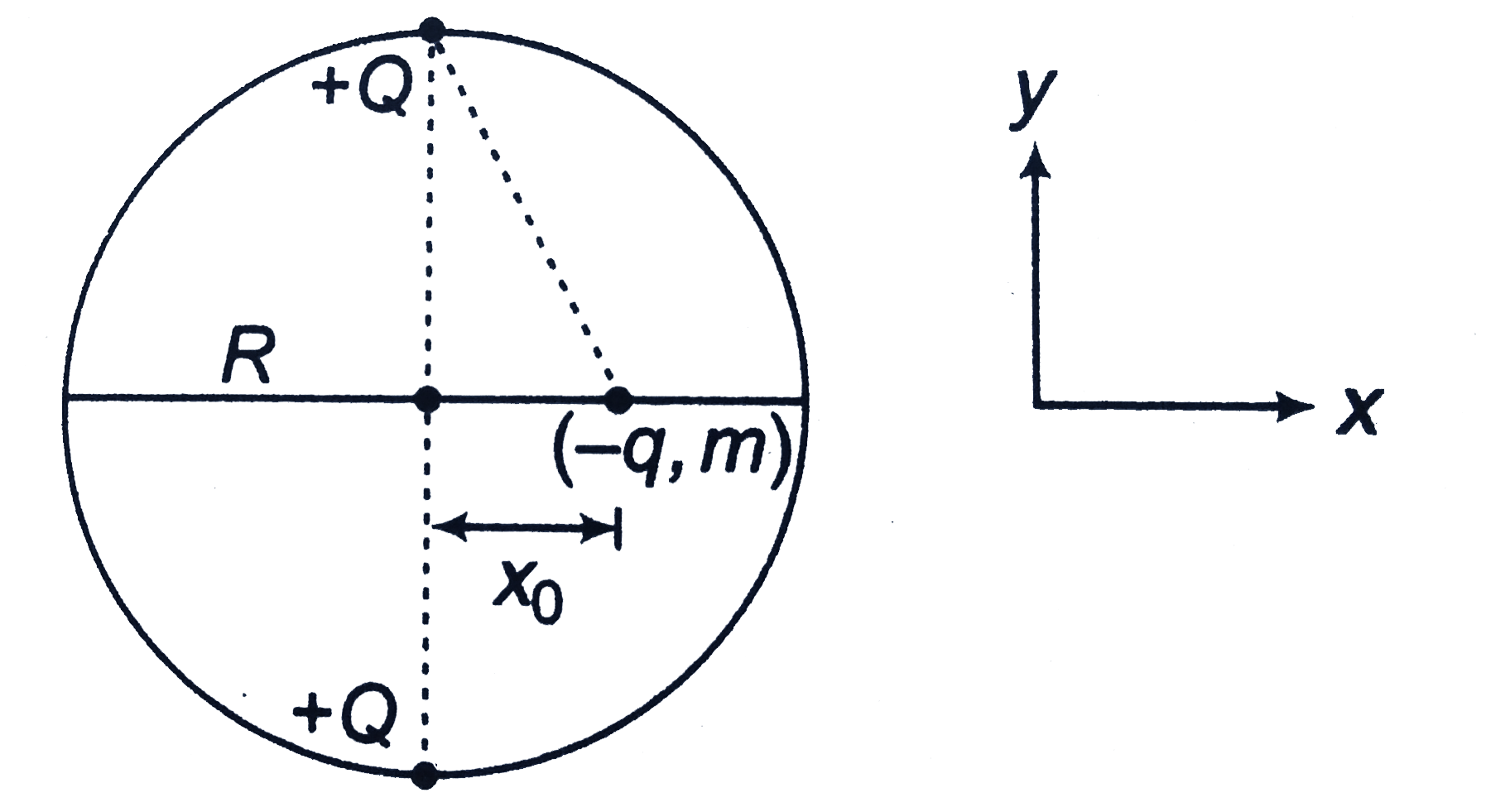
a. What is the resultant acting on the charged bead?
b. Describe (qualitatively) the motion of the bead after it is released.
c. Use the assumption that x/R<<1 to obtain an approximate equation of motinand find the dislacemet ad velocity of the bead as functuions of time.
d. When will the velocity of the bead will become zero for the first time?
View SolutionA bead slides on a frictoinless strainght rod fixed between points A and B of a vertical circular loop of radius as shown in the figure. Line BC is a vertical diameter. Denoting acceleration due to gravity by g, which of the following is correct expression for the time taken by the bead to slide from A to B
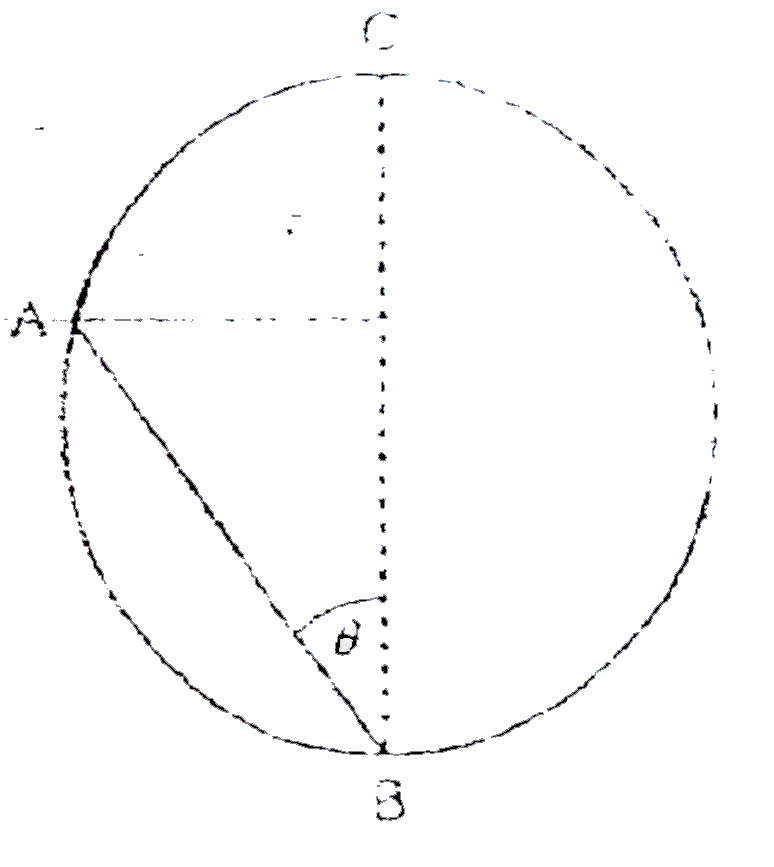
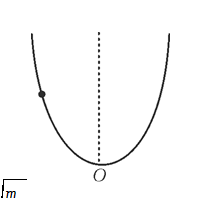
View SolutionA bead of mass m slides down a frictionless thin fixed wire held on the vertical plane and then performs small oscillations at the lowest point O of the wire. The wire takes the shape of a parabola near O and potential energy is given by U (x)=cx2, where c is a constant. The period of the small oscillations will be :
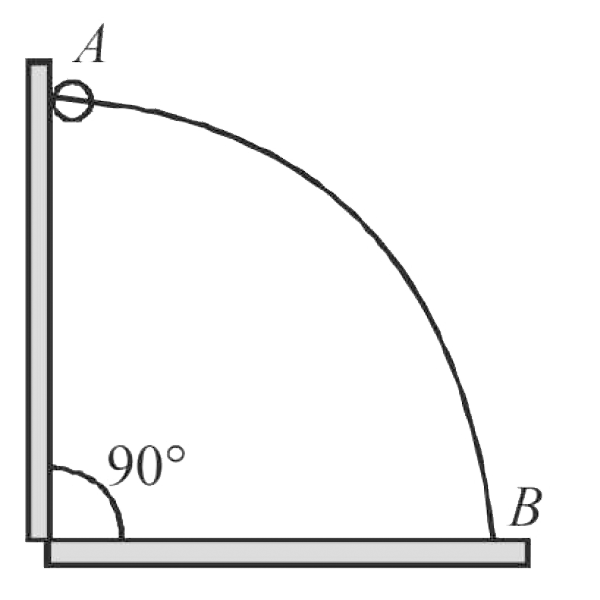
View SolutionA wire, which passes through the hole in a small bead, is bent in the form of quarter of a circle. The wire is fixed vertically on ground as shown in the figure. The bead is released from near the top of the wire and it slides along the wire without friction. As the bead moves from A to B, the force it applies on the wire is
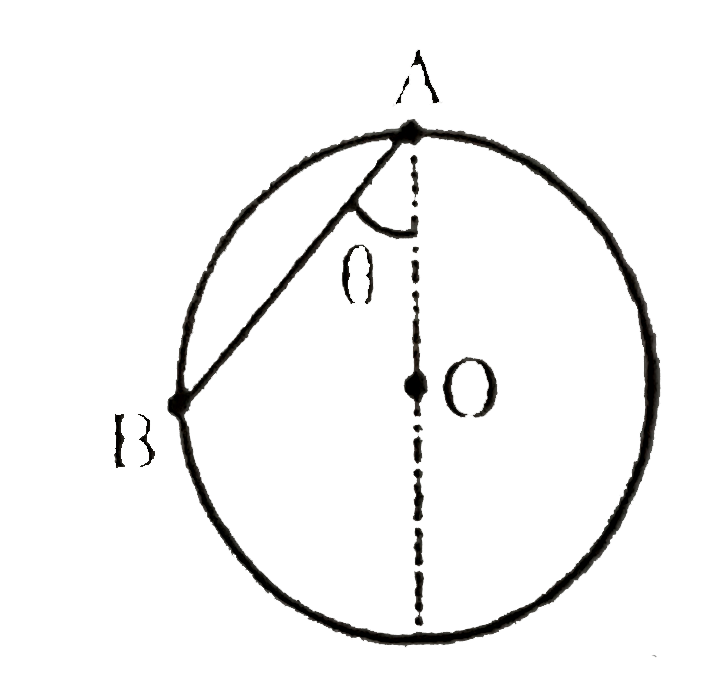
View SolutionA bead is free to slide down a smooth wire tightly stretched between points A and on a verticle circle of radius R. if the bead starts from rest at 'A', the highest point on the circle, its velocity when it arrives at B is :-
View Solution
