Question

A particle performing simple harmonic motion undergoes unitial displacement of $\frac{A}{2}$ (where A is the amplitude of simple harmonic motion) in 1 s. At $t=0$, the particle may be at he extreme position or mean position the time period of the simple harmonic motion can be

Options

A. `12s`
B. `2.4`
C. `6s`
D. `1.2s`

Answer

A::B::C::D

Explanation

It `T` be the time period`,` time to fo from `O` to `Q` is `(T)/(12)` and from `M` to `P` is `(T)/(6)`

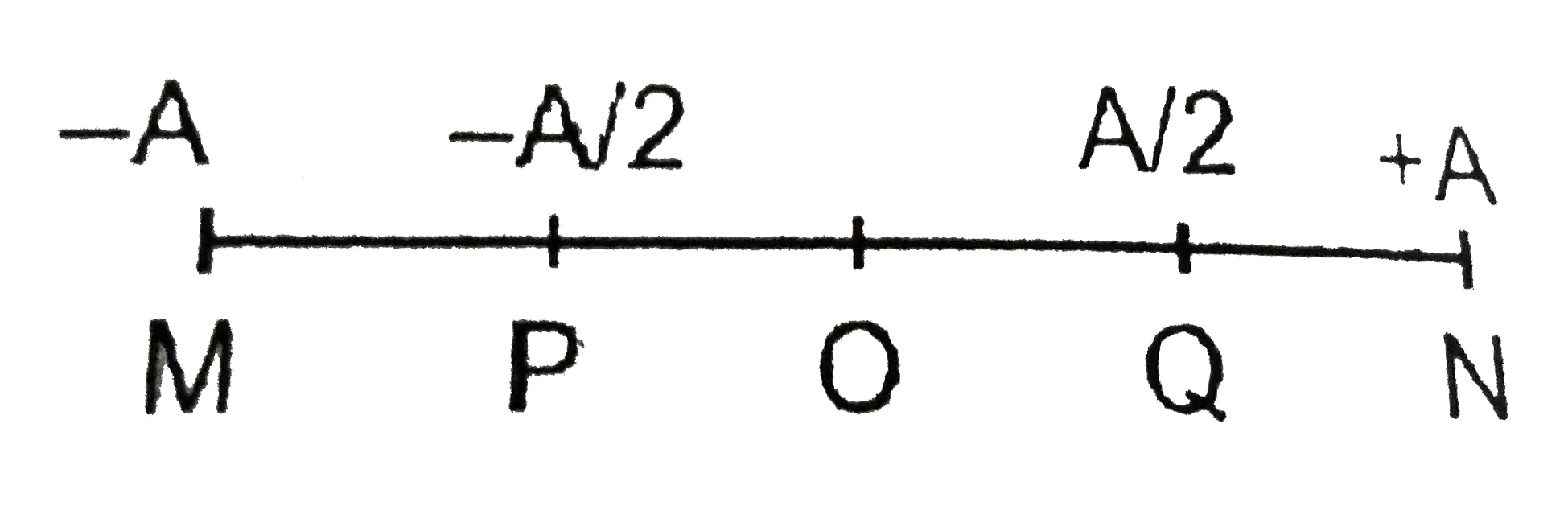
The displacement is `(A)?(2)` when particle goes from `O` to `Q`, from `O` to `N` to `Q`.
from `O` to `N` to `O` to `P`, then so on
`:. t=(T)/(12)`or `t=(T)/(4)+(T)/(6)=(5T)/(12)`
or `t=(T)/(2)+(T)/(12)=(7T)/(12)`
Hence possible time period `T` is
`T=12s ` or `T=(12xx1)/(5) 2.4 s ` or `T=(12xx1)/(7)s`
similarly displacements is `(A)/(2)` when particle goes from `M` to `P` or `M` to `N` to `P`
Hence the possible time period `T` is
`T=1xx6=6s` or `T=(6xx1)/(5)s=1.2s`
`Ans. T=1.2s,6s,2.4s,12s`