A stone is dropped from the top of a tower. When it crosses a point 5 m below the top, another stone is let fall from a point 25 m below the top. Both stones reach the bottom of the tower simultaneously. Find the height of the tower. Take $g = 10 m / s^{2} .$

A

$45 m$

B

$50 m$

C

$60 m$

D

$65 m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Vel. Of 1st stone when passing at $A \to$
$V^{2} = 0 + 2.10 .5$
$S_{1} - S_{2} = 20 m .$
$\Rightarrow (10 . t + \frac{1}{2} 10 . t^{2}) - (\frac{1}{2} .10 . t^{2}) = 20$

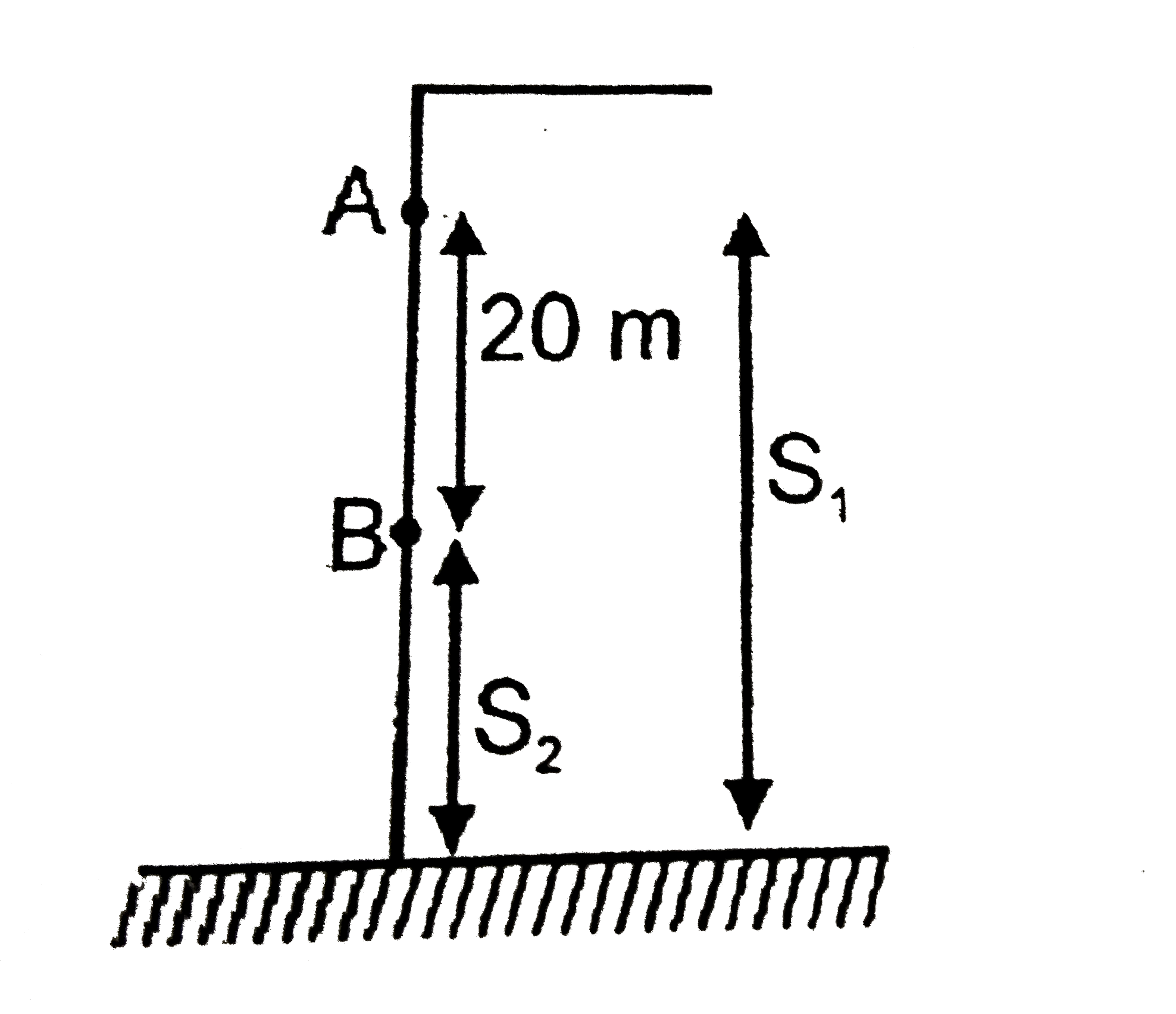
$t = 2 s$
$S_{2} = \frac{1}{2} .10 .4 = 20 m$
$H t = 25 + 20 = 45 m .$

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A stone is dropped from the top of tower. When it has fallen by $5 m$ from the top, another stone is dropped from a point $25 m$ below the top. If both stones reach the ground at the moment, then height of the tower from grounds is : (take $g = 10 m / s^{2}$ )
A $45 m$
B $50 m$
C $60 m$
D $65 m$
Question 2 - Select One
A stone is dropped from the top of a tower of height $h$ . Aftre $1 s$ another stone is droppped from the balcony $20 m$ below the top. Both reach the bottom simultaneously. What is the value of $h$ ? Take $g = 10 m s^{- 2}$ .
A $315 m$
B $312.5 m$
C $3125 m$
D $25, 31$
Question 3 - Select One
A stone dropped from the top of a tower reaches the ground in 3 s. The height of the tower is
A18.6 m
B39.2 m
C44.1 m
D98 m

RESONANCE-DAILY PRACTICE PROBLEMS-DPP NO. 14 PHYSICS

Home
Ask Doubt
Profile