Position vector →A is 2ˆi
Position vector −→B0 is 3ˆj
ˆi,ˆj,ˆk are along the shown x,y, and z is
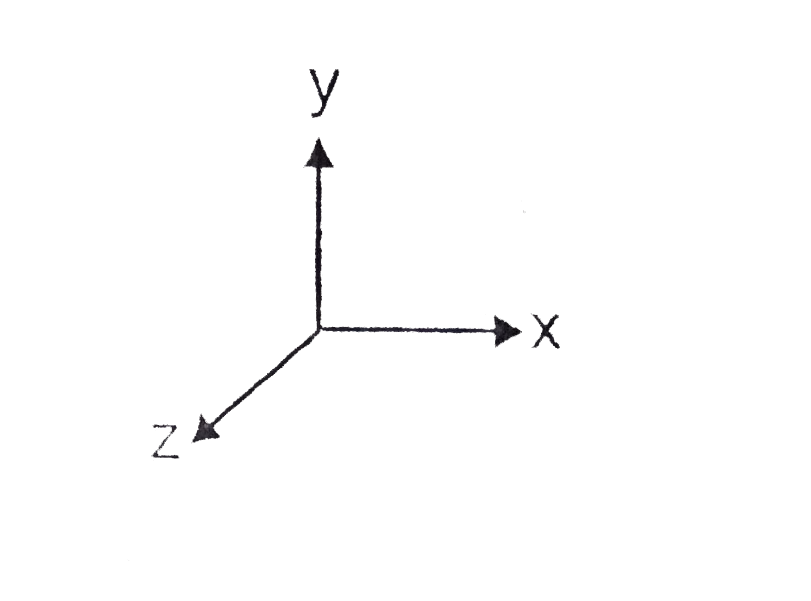
−4→A can be represented as
A
−−−→8units
B
←−−−8units
C
←−−A
D
−−→A
Video Solution
Text Solution
The correct Answer is:B
−4→A=−8i←−−−8units
|
Similar Questions
Knowledge Check
Position vector →A is 2ˆi
Position vector −→B0 is 3ˆj
ˆi,ˆj,ˆk are along the shown x,y, and z is

Geometrical representation of →A isA−−−→2unitsB↑2unitsC←−−−2unitsD¯¯¯¯¯¯¯¯¯¯¯2unitsPosition vector →A is 2ˆi
Position vector −→B0 is 3ˆj
ˆi,ˆj,ˆk are along the shown x,y, and z is

Geometrical representation of →B is :A3unitsB↑3unitsC←−−−3unitsD¯¯¯¯¯¯¯¯¯¯¯2unitsVector →A=ˆi+ˆj−2ˆk and →B=3ˆi+3ˆj−6ˆk are :
AParallelBAntiparallelCPerpendicularDat acute angle with each other
Linear momentum →P=2ˆi+4ˆj+5ˆk and position vector is →r=3ˆi−ˆj+2ˆk, the angular momentum is given by
View SolutionIf →A=2ˆi+ˆj+ˆk and →B=ˆi+ˆj+ˆk are two vectors, then the unit vector is
View SolutionA unit vector in the dirction of resultant vector of →A=−2ˆi+3ˆj+ˆk and →B=ˆi+2ˆj−4ˆk is
View SolutionDetermine a vector product of →A=ˆi+ˆj+ˆkand→B=−3ˆi+ˆj+ˆk
View SolutionGiven →A=3ˆi+2ˆjand→B=ˆi+ˆj. The component of vector →A along vector →B is
View Solution