106 positrons are flowing normally through an area in forward direction and same amount of electrons are flowing in backward direction in the interval of 10 ms.Find the current through the area.
To solve the problem, we will follow these steps:
Step 1: Understand the Charges Involved
We have:
- 106 positrons flowing in the forward direction.
- 106 electrons flowing in the backward direction.
Step 2: Calculate the Total Charge for Each Particle Type
The charge of one positron is equal to the charge of one electron, which is:
e=1.6×10−19 coulombs
- Total charge from positrons (Q1):
Q1=106×1.6×10−19=1.6×10−13 coulombs
- Total charge from electrons (Q2):
Q2=106×(−1.6×10−19)=−1.6×10−13 coulombs
Step 3: Calculate the Net Charge Flowing
Since the positrons are moving in one direction and the electrons in the opposite direction, the net charge (Q) flowing through the area is given by:
Q=Q1−Q2=1.6×10−13−(−1.6×10−13)=1.6×10−13+1.6×10−13=3.2×10−13 coulombs
Step 4: Calculate the Current
Current (I) is defined as the rate of flow of charge:
I=QT
Where T is the time interval during which the charge flows. Given that T=10 ms=10×10−3 seconds=10−2 seconds, we can substitute the values:
I=3.2×10−1310×10−3=3.2×10−1310−2=3.2×10−11 amperes
Final Answer
The current through the area is:
I=3.2×10−11 A
---
We have:
- 106 positrons flowing in the forward direction.
- 106 electrons flowing in the backward direction.
The charge of one positron is equal to the charge of one electron, which is:
e=1.6×10−19 coulombs
- Total charge from positrons (Q1):
Q1=106×1.6×10−19=1.6×10−13 coulombs
- Total charge from electrons (Q2):
Q2=106×(−1.6×10−19)=−1.6×10−13 coulombs
Since the positrons are moving in one direction and the electrons in the opposite direction, the net charge (Q) flowing through the area is given by:
Q=Q1−Q2=1.6×10−13−(−1.6×10−13)=1.6×10−13+1.6×10−13=3.2×10−13 coulombs
Current (I) is defined as the rate of flow of charge:
I=QT
Where T is the time interval during which the charge flows. Given that T=10 ms=10×10−3 seconds=10−2 seconds, we can substitute the values:
I=3.2×10−1310×10−3=3.2×10−1310−2=3.2×10−11 amperes
The current through the area is:
I=3.2×10−11 A
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTECHAPTER - CURRENT ELECTRICITYEXERCISE - Try Yourself
32 Videos
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTECHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT(SECTION-A(OBJECTIVE TYPE QUESTIONS))
59 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTECHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTECHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
Knowledge Check
The direction of the flow of current through electric circuit is
Afrom low potential to high potential.Bfrom high potential to low potential.Cdoes not depend upon potential valueDcurrent cannot flow through circuit.If the current flowing through a circuit is 0.6 A for 6 mins ,the amount of electric charge flowing through it is _____.
A360 CB216 CC60 CD36 C62.5×1018 electrons per second are flowing through a wire of area of cross-section 0.1m2, the value of current flowing wil be
A1AB0.1 AC10AD0.11 A
How much charge flows through a wire in 10 minutes if the current through it is 2.5 A?
View SolutionIn the circuit, the current flowing through 10Ω resistance
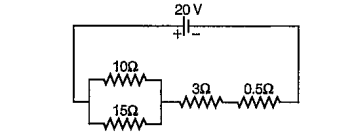
View SolutionIf the amount of electric charge passing through a conductor in 10 minutes is 300C, the current flowing is:
View Solution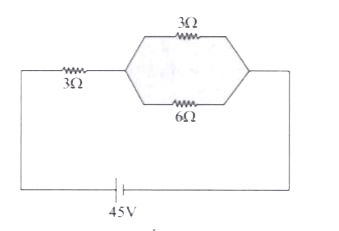
Find the amount of current that flows through the 6Ω resistor.
View Solution62.5×1018 electrons per second are flowing through a wire of area of cross-section 0.1m2, the value of current flowing wil be
View Solution
