STATEMENT-1 : At a point electrostatic field is zero, then potential at that point may be zero .
and
STATEMENT-2 : Negative of the potential gradient is equal to electrostatic field.
Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1
Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1
Statement-1 is True, Statement-2 is False
Statement-1 is False , Statement-2 is True
The correct Answer is:2
To analyze the statements given in the question, we will break down the concepts of electrostatic fields and electric potential step by step.
Step 1: Understanding Electrostatic Field and Electric Potential
The electrostatic field (E) at a point in space is a vector quantity that represents the force experienced by a unit positive charge placed at that point. The electric potential (V) at a point is a scalar quantity that represents the work done in bringing a unit positive charge from infinity to that point against the electric field.
Hint: Remember that electric field is related to the force on a charge, while electric potential relates to the work done on a charge.
Step 2: Analyzing Statement 1
Statement 1: "At a point where the electrostatic field is zero, the potential at that point may be zero."
If the electrostatic field is zero at a point, it implies that there are no forces acting on a unit charge at that point. However, the potential at that point can be any value. For example, at infinity (where the electric field is zero), the potential can be defined as zero, but at other points, the potential can be positive or negative. Thus, the statement is true.
Hint: Consider the concept of potential at infinity and how it can differ from potential at other points.
Step 3: Analyzing Statement 2
Statement 2: "The negative of the potential gradient is equal to the electrostatic field."
This statement is a fundamental relationship in electrostatics. The electrostatic field (E) is defined as the negative gradient of the electric potential (V). Mathematically, this is expressed as:
E=−dVdx
This means that the electric field points in the direction of decreasing potential. Therefore, this statement is also true.
Hint: Remember the mathematical relationship between electric field and potential gradient.
Step 4: Conclusion
Both statements are correct, but they do not imply each other. Statement 1 discusses a condition where the electric field is zero, while Statement 2 provides a relationship between electric field and potential gradient. Therefore, both statements are true independently.
Final Answer: Both statements are correct, but they do not explain each other.
The electrostatic field (E) at a point in space is a vector quantity that represents the force experienced by a unit positive charge placed at that point. The electric potential (V) at a point is a scalar quantity that represents the work done in bringing a unit positive charge from infinity to that point against the electric field.
Statement 1: "At a point where the electrostatic field is zero, the potential at that point may be zero."
Statement 2: "The negative of the potential gradient is equal to the electrostatic field."
E=−dVdx
This means that the electric field points in the direction of decreasing potential. Therefore, this statement is also true.
Both statements are correct, but they do not imply each other. Statement 1 discusses a condition where the electric field is zero, while Statement 2 provides a relationship between electric field and potential gradient. Therefore, both statements are true independently.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-F(MATRIX-MATCH TYPE QUESTIONS)
2 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-G(INTEGER ANSWER TYPE QUESTIONS)
8 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-D(COMPREHENSION-I)
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
- GRAVITATION BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
16 Videos
Similar Questions
Knowledge Check
The neagative gradient of potential at any point in electric field is
Aelectric intensityBelectric fluxCelectric dipole momentDnone of theseElectric potential at some point in space is zero then at that point
Aelectric intensity is necessarily zeroBelecrtic intensity is necessarily non zeroCelectric intensity of may or may not be zeroDelectric intensity is necessarily infinteConsider the three statements in context of electrostatic field lines.
STATEMENT-1 : Electrostatic field lines are continuous curves in space.
STATEMENTS-2 : Electrostatic field lines are always perpendicular to equipotential surface.
STATEMENT-3 : A charge when released in electrostatic field moves along electrostatic field lines.AT T FBT F TCF T FDT F F
If electric field →E is zero at a particular point then potential V at that point.....
View SolutionShow that the electric field intensity at a point can be given as negative of potential gradient.
View SolutionAssertion: If electric potential remains constant along a path in electric field, then the electric field must be zero.
Reason: The negative of an electric potential gradient in a direction is equal to the component of electric field in that direction.
View SolutionSTATEMENT -1 : Induced charge does not contribute to electric field or potential at a give point .
STATEMENT -2 : A point cahrge q0 is kept outside a solid metallic sphere , the electric field inside the sphere is zero.
View SolutionIn the given circuit the potential at point B is zero, the potential at points A and D will be
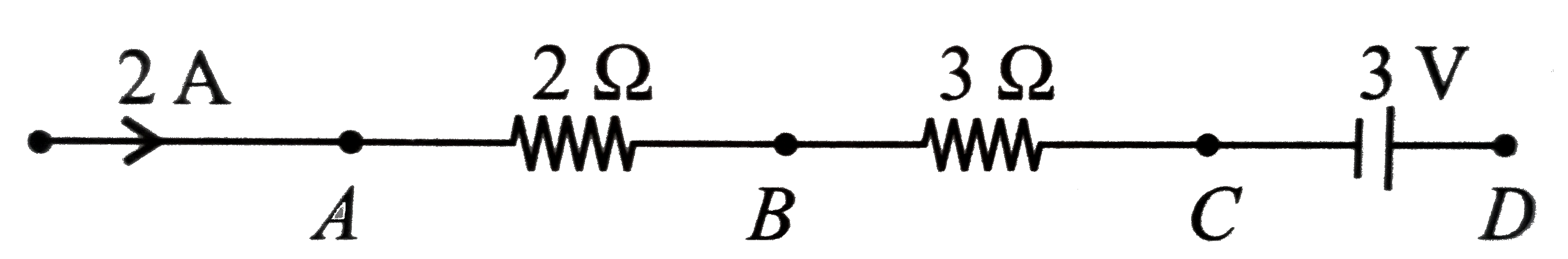
View Solution
