Two short dipoles, each of diple moment p , are placed at origin. The dipole moment of one dipole is along x-axis, while that of other is along y-axis . The electric field at a point (a,0) is given by
Zero
The correct Answer is:3
To find the electric field at the point (a, 0) due to two short dipoles placed at the origin, we can follow these steps:
Step 1: Understand the dipoles' configuration
We have two dipoles at the origin:
- Dipole 1 has a dipole moment →p1 along the x-axis.
- Dipole 2 has a dipole moment →p2 along the y-axis.
Step 2: Write the formula for the electric field due to a dipole
The electric field →E at a point along the axial line of a dipole is given by:
→E=14πϵ0⋅2→pr3
where →p is the dipole moment and r is the distance from the dipole to the point of interest.
Step 3: Calculate the electric field due to each dipole at point (a, 0)
1. Electric field due to dipole 1 (along x-axis):
- The distance from the dipole to point (a, 0) is r=a.
- The electric field →E1 due to dipole 1 is:
→E1=14πϵ0⋅2pa3^i
2. Electric field due to dipole 2 (along y-axis):
- The distance from the dipole to point (a, 0) is still r=a.
- The electric field →E2 due to dipole 2 is:
→E2=14πϵ0⋅pa3^j
Step 4: Combine the electric fields
Since the two dipoles are perpendicular to each other, we can find the resultant electric field →E by using the Pythagorean theorem:
→E=√E21+E22
Substituting the expressions for E1 and E2:
E=√(14πϵ0⋅2pa3)2+(14πϵ0⋅pa3)2
=√(2p4πϵ0a3)2+(p4πϵ0a3)2
=√4p2(4πϵ0)2a6+p2(4πϵ0)2a6
=√5p2(4πϵ0)2a6=√5p4πϵ0a3
Step 5: Final expression for the electric field
Thus, the electric field at the point (a, 0) due to the two dipoles is:
→E=√5p4πϵ0a3
We have two dipoles at the origin:
- Dipole 1 has a dipole moment →p1 along the x-axis.
- Dipole 2 has a dipole moment →p2 along the y-axis.
The electric field →E at a point along the axial line of a dipole is given by:
→E=14πϵ0⋅2→pr3
where →p is the dipole moment and r is the distance from the dipole to the point of interest.
1. Electric field due to dipole 1 (along x-axis):
- The distance from the dipole to point (a, 0) is r=a.
- The electric field →E1 due to dipole 1 is:
→E1=14πϵ0⋅2pa3^i
- The distance from the dipole to point (a, 0) is still r=a.
- The electric field →E2 due to dipole 2 is:
→E2=14πϵ0⋅pa3^j
Since the two dipoles are perpendicular to each other, we can find the resultant electric field →E by using the Pythagorean theorem:
→E=√E21+E22
Substituting the expressions for E1 and E2:
E=√(14πϵ0⋅2pa3)2+(14πϵ0⋅pa3)2
=√(2p4πϵ0a3)2+(p4πϵ0a3)2
=√4p2(4πϵ0)2a6+p2(4πϵ0)2a6
=√5p2(4πϵ0)2a6=√5p4πϵ0a3
Thus, the electric field at the point (a, 0) due to the two dipoles is:
→E=√5p4πϵ0a3
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTEChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:SECTION-C (OBJECTIVE TYPE QUESTIONS (More than one answer))
Explore 4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTEChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:SECTION-D(COMPREHENSION-I)
Explore 3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:AAKASH INSTITUTEChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:SECTION-B (OBJECTIVE TYPE QUESTIONS (ONLY ONE ANSWER) )
Explore 1 Video
- ELECTROMAGNETIC WAVESBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC WAVESExercise:ASSIGNMENT SECTION - D Assertion-Reason Type Questions
Explore 25 Videos
- GRAVITATION Book:AAKASH INSTITUTEChapter:GRAVITATION Exercise:ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
Explore 16 Videos
Similar Questions
A short magnetic dipole when placed at origin with its dipole moment oriented along x-axis , the magnetic field at the point (a,0) is →B . What will be the magnetic field at the above point , if another identical dipole is also placed at origin with its dipole moment oriented along y-axis ?
View SolutionA dipole of dipole moment p is placed at origin along x axis. Another dipole of dipole moment also p is kept at (0, 1, 0) along y axis. Find the resultant potential and electric field at (1, 0, 0).
View Solution
Knowledge Check
Two short dipoles , each of dipole moment P are placed at a large separation r. The force between them
Ais proportional to product of dipole momentaBis inversely proportional to r4Cthe force is attractive , if direction of dipole momenta are same , repulsive if oppositeDall options are correctFour short dipoles each of dipole moment 'p' are placed at the vertices of a square of side a. The direction of the dipole moments are shown in the figure.
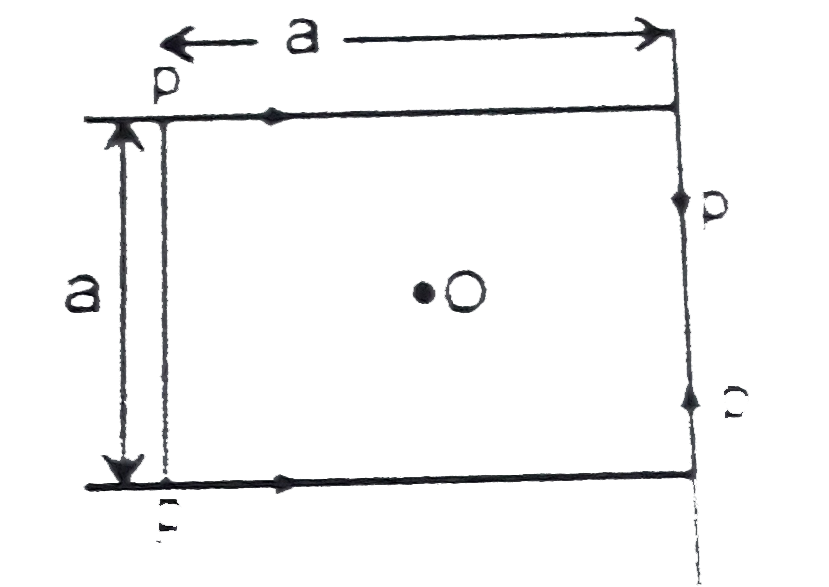 AElectric field at O is √2p2π∈0a3BElectric field at O is √2pπ∈0a3CElectrostatic potential at O is zeroDNet dipole moment is 2p
AElectric field at O is √2p2π∈0a3BElectric field at O is √2pπ∈0a3CElectrostatic potential at O is zeroDNet dipole moment is 2pA small electric dipole is placed at origin with its dipole moment directed along positive x-axis .The direction of electric field at point (2,2√2,0)
Aalong z-axisBalong y-axisCalong negative y-axisDalong negative z-axis
A short electric dipole has dipole moment p. Find the distance of farthest point from the dipole where (a) potential due to the dipole is V0 (b) Electric field due to the dipole is E0
View SolutionTen dipoles ,each of dipole moment 'p' , are placed inside a hollow sphere . The total electric flux coming out of the sphere will be
View SolutionA small electric dipole is placed at origin with its dipole moment directed along positive x−aξs .The direction of electric field at point (2,2sqrt(2),0)
View SolutionAn electric dipole is placed along x-axis with its centre at origin:
View SolutionAn electric dipole is placed at origin in the xy plane with its orientation along the positive x-axis. The direction is electric field .
View Solution