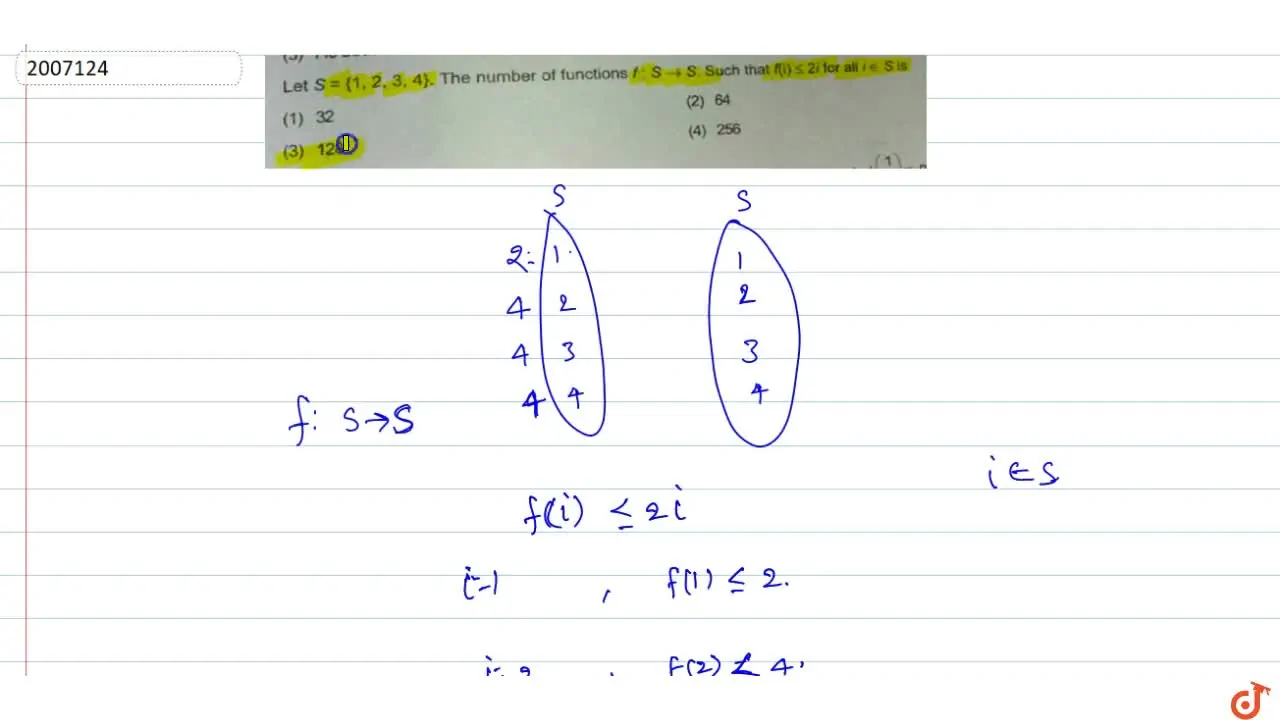
Let S={1,2,3,4). The number of functions f:S→S.Such that f(i)≤2i for all i∈S is
32
64
128
256
The correct Answer is:C
Step by step video, text & image solution for Let S = {1, 2, 3, 4). The number of functions f: S->S.Such that f(i) le 2i for all i in S is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - I) Subjective Type Questions15videos
RELATIONS AND FUNCTIONS
- BOOK - AAKASH INSTITUTECHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-J (aakash challengers questions)13videos
PROBABILITY
- BOOK - AAKASH INSTITUTECHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers12videos
SEQUENCES AND SERIES
Similar Questions
Let S={1,2,3,4). The number of functions f:S→S.Such that f(i)≤2i for all i∈S is
View SolutionLet S = {1, 2, 3, 4}. The number of functions f:S→S which satisfy the condition f(f(x))=x∀x∈S, is
View SolutionLetA={1,2,3,4}. The number of functionsf:A→A satisfying f(f)=1 for all 1≤i≤4 is (A) 1 (B) 6 (C) 9 (D) 10
View SolutionLet A={1,2,3,4,5} and B={-2,-1,0,1,2,3,4,5}.
Onto functions from A to A such that f(i)≠i for all i, isView SolutionLet S={1,2,3,45,6,7}. Then the number of possible functions f:S→S such that f(m.n)= f(m).f(n) for every m,n∈Sandm.n∈S is equal to ____
View SolutionLet A={1,2,3,4,5} and f:A→A be an into function such that f(i)≠,∀i∈A, then number of such functions f are
View SolutionLet: f:{x1,x2,x3}→{1,2,3,4,..,13}beafunction,Theνmberoffunctions'fsuchtˆf(x_i+1)-f(x_i)=2fori=1,2 is
View SolutionLet R = (a, b, c, d} and S = {1, 2, 3), then the number of function f, from R to S, which are onto is
View SolutionLet S={1,2,3}. Determine whether the functions f:S→S defined as below have inverses. Find f−1, if it exists
.(a) f={(1,1),(2,2),(3,3)}
(b) f={(1,2),(2,1),(3,1)}
(C) f={(1,3),(3,2),(2,1)}View SolutionThe number of functions f from the set A = {0, 1, 2} in to the set B = {0, 1, 2, 3, 4, 5, 6, 7} such that f(i)≤f(j) for i<j and i,j∈A is
View Solution