For given equation x2−ax+b=0 , match conditions in column I with possible values in column II
column -I Colum-II(A) If roots differ by unity then a2 is equal to(p) b(ab+2)(B) If roots differ by unity then 1+a2 is equal to (q) 1 + 4b(C) If one of the root be twice the other then 2a2 is equal to (r) 2(1+2b)(D) If the sum of roots of the equation equal to the sum of squares of their reciprocal then a2 is equal to (s) 9b
The correct Answer is:A(q), B( r) , C(s) , D(p)
Step by step video, text & image solution for For given equation x^(2) -ax+b=0 , match conditions in column I with possible values in column II {:("column -I " , "Colum-II"),("(A) If roots differ by unity then " a^(2)" is equal to" , "(p) b(ab+2)"),("(B) If roots differ by unity then " 1 + a^(2) " is equal to " , "(q) 1 + 4b"),("(C) If one of the root be twice the other then " 2a^(2) " is equal to " , "(r) 2(1+2b)"),("(D) If the sum of roots of the equation equal to the sum of squares of their reciprocal then " a^(2) " is equal to " , "(s) 9b"):} by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - section-G( Integer Answer type Questions)
8 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - section-H ( Multiple True-False Type Questions
5 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - Assertion -Reason Type Questions
19 Videos
- BINOMIAL THEOREMBOOK - AAKASH INSTITUTECHAPTER - BINOMIAL THEOREMEXERCISE - Assignment (section-J) Objective type question (Aakash Challengers Questions)
4 Videos
- CONIC SECTIONSBOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - SECTION - J ( Aakash Challengers Questions )
14 Videos
Similar Questions
For the quadratic equatio x2−(k−3)x+k=0 , match the condition in condition in column I with the correspending values of k in column II.
{:("Column-I " , " Column -II"),("(A) Both the roots are positive", "(p) " phi),("(B) Both the roots are negative " , "(q) " (-oo","1) cup (9","oo)),("(C) Both the roots are real " , "(r) " [10 "," oo)),(" (D) one root is less than -1 and other is greater than 1" "(s) " (0","1]),(" " , "(t) " [ 125"," 1250]):}
View SolutionIf the ratio of the roots of the equation ax2+bx+c=0 is equal to ratio of roots of the equation x2+x+1=0 then a,b,c are in
View Solution
Knowledge Check
if the difference of the roots of the equation x2+ax+b=0 is equal to the difference of the roots of the equation x2+bx+a=0 ,then
Aa + b =4Ba = b = - 4Ca -b = 4Da -b = - 4Match the Column I and Column II.,B
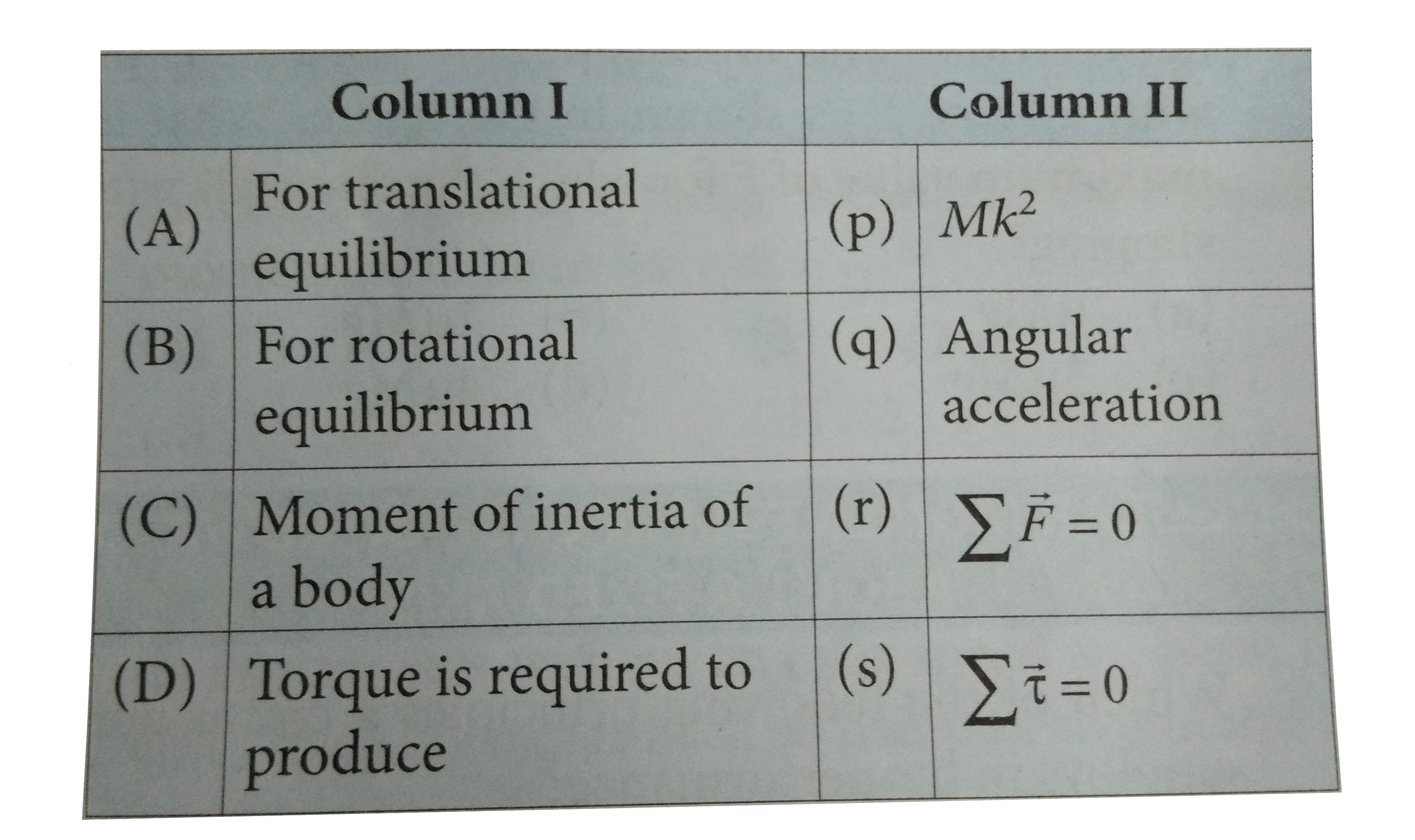 AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-q
AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-qif the difference of the roots of the equation x2−px+q=0 is unity.
Ap2+4q=1Bp2−4q=1Cp2−4q2=(1+2q)2D4p2+q2=(1+2p)2
If the roots of the equation x2+ax+b=0 are twice the roots of x2+cx+a=0 where a,b,c∈R−{0}, then b/c is equal to-
View Solution. If the difference of the roots is equal to the product of the roots of the equation 2x2−(a+1)x+(a−1)=0then the value of a is
View SolutionIf the roots of the equationax2+x+b=0 be real, and unequal then the roots of the equation x2−4√abx+1=0 will be
View SolutionMatch the following
Column-I Column -II(A) if the roots of the equationx2−2ax+a2+a−3=0 are real and less than 3, then a belongs to (p) a does not exist(B) If the roots of the equation x2−2ax+a2+a−3=0 are real and greater than , 3 then a belongs to (q) (32,3)(C) If exactly one root of the above equation lies in the interval (1,3) then a belongs to (r) (−1,2)∪(2,3)(D) IF the roots of the above equation are such that one root is greater than 3 and other smaller than 1, then a belongs to (s) (−∞,2)
View SolutionIf 4 - 5i is a root of the quadratic equation x2+ax+b=0 then ( a, b) is equal
View Solution
