The co-ordinates of the middle point of the chord cut off on 2x - 5y +18= 0 by the circle x2+y2−6x+2y−54=0 are (A) (1,1) (B) (2, 4) (C) (4,1) (D) (1, 4)
A
(1,4)
B
(2,4)
C
(4,1)
D
(1,1)
Video Solution
Text Solution
The correct Answer is:A
The co-ordinates …………
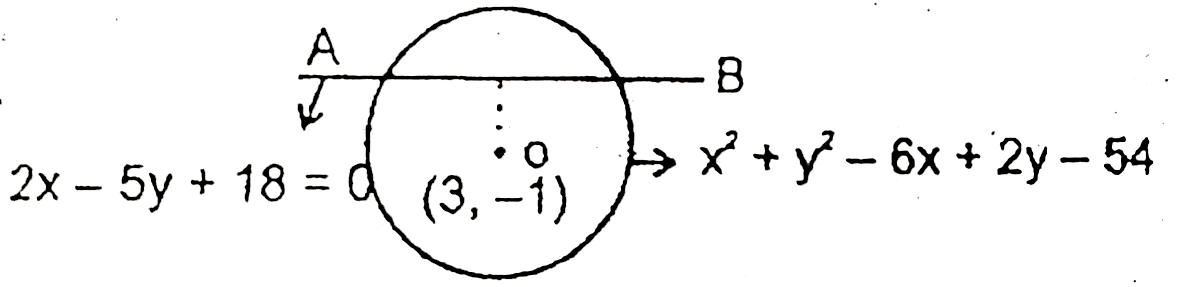
2x−5y+18=0 …….(i)
x2+y2−6x+2y−5=0 ……….(ii)
let mid point be (h,k)
mOM×mAB=−1
(K+1h−3)×(25)=−1 and (h,k) lies on 2x−5y+18=0
Hence 2h−2k+18=0 ......(ii)
by (i) and (ii) (h,k)=(1,4)
|
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PART : 1MATHEMATICS SEC - 1
Explore 1 Video
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PART : 1MATHEMATICS
Explore 9 Videos
- TEST PAPERBook:RESONANCEChapter:TEST PAPERExercise:CHEMISTRY
Explore 37 Videos
- TEST SERIESBook:RESONANCEChapter:TEST SERIESExercise:MATHEMATICS
Explore 131 Videos
Similar Questions
Knowledge Check
The co-ordinates of the limiting points of the co-axial system of circles x2+y2+3x+2y−4=0 and x2+y2−x−2y+4=0 are
A(3−√234,5−√234)B(3+√234,5+√234)C(3+√232,5+√232)DnoneThe length of tangent from (5,1) to the circle x2+y2+6x−4y−3=0 is
A7B49C63D21
Find the co-ordinates of the mid-point of the chord intersect by the lime x-y+2=0 and the circle x2+y2+4x−2y=0.
View SolutionFind the coordinates of the middle point of the chord which the circle x2+y2+4x−2y−3=0 cuts off on the line x−y+2=0
View SolutionFind the length of the chord cut-off by y=2x+1 from the circle x2+y2=2
View SolutionFind the co-ordinates of the middle point of the chord which the circle x2+y2−2x+2y−2=0 cuts off on the line y=x−1
View SolutionFind the coordinates of the middle point of the chord which the circle x2+y2+4x−2y−3=0 cuts-off the line x-y+2=0.
View SolutionLength of the chord on the line 4x−3y+10=0 cut-off by the circlex2+y2−2x+4y−20=0 is
View Solution
