A point sources $S$ emitting light of wavelength $600 n m$ is placed at a very small height $h$ above the flat reflecting surface $A B$ (see figure).The intensity of the reflected light is $36 %$ of the intensity.interference firnges are observed on a screen placed parallel to the reflecting surface a very large distance $D$ from it.
(A)What is the shape of the interference fringes on the screen?

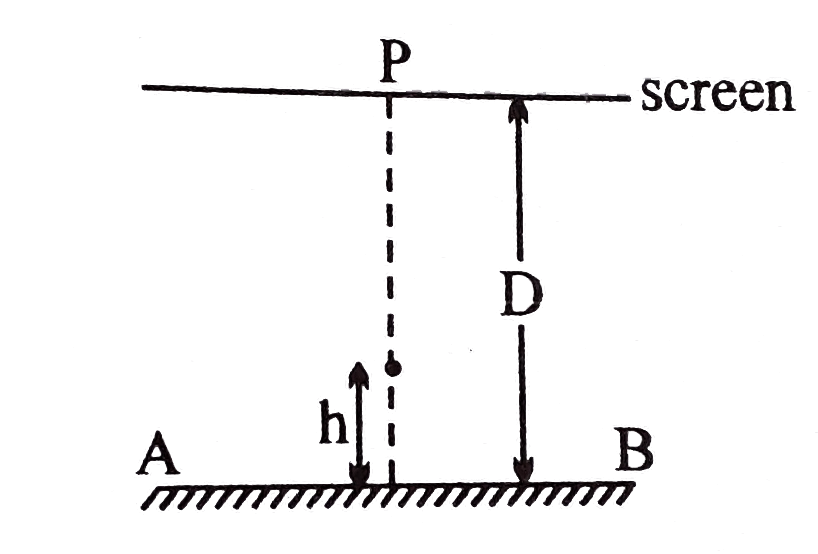
(B)Calculate the ratio of the minimum to the maximum to the maximum intensities in the interference fringes fromed near the point $P$ (shown in the figure) (c) if the intenstities at point $P$ corresponds to a maximum,calculate the minimum distance through which the reflecting surface $A B$ should be shifted so that the intensity at $P$ again becomes maximum.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(i). Circular
(ii). $\frac{1}{16}$
(iii). 300 nm.

(i). S is a point source, fringes formed will be circular
(ii). Ratio of minimum and maximum intensities intensity of light direct from source $I_{1} = I_{0}$ (say)
Intensity after reflection $I_{2} = 0.36 I_{0}$
$∴ \frac{I_{min}}{I_{max}} = {(\frac{\sqrt{I_{1}} \sqrt{I_{2}}}{\sqrt{1}} + \sqrt{I_{2}})}^{2} = {(\frac{0.4}{1.6})}^{2} = \frac{1}{16}$
(iii). Shift of AB for same intensity
if intensity at P correspionds to maximum it means that constructive interference occurs at P.
$∴$ Path difference between direct waves from S and reflected waves, from reflector AB, is $n λ$
Let AB is shifted by x (towards P or away from P)
$∴$ Additional path difference introduced =2x
For minimum value of $x_{1}, n = 1$
$∴$ Path difference $= 1 \times λ = 600 n m$
$2 x = 600 n m \Rightarrow x = 300 n m$

Updated on:21/07/2023

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

Knowledge Check

A point sources $S$ emitting light of wavelength $600 n m$ is placed at a very small height $h$ above the flat reflecting surface $A B$ (see figure).The intensity of the reflected light is $36 %$ of the intensity.interference firnges are observed on a screen placed parallel to the reflecting surface a very large distance $D$ from it.
(A)What is the shape of the interference fringes on the screen?

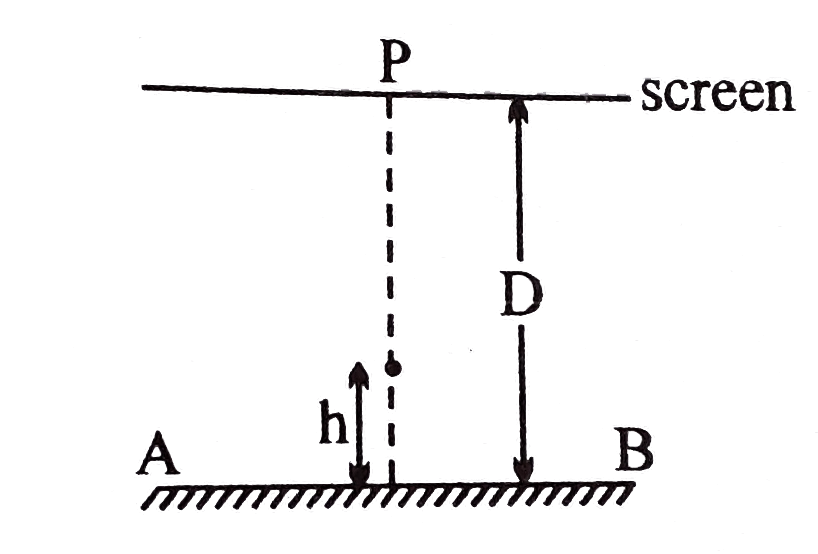
(B)Calculate the ratio of the minimum to the maximum to the maximum intensities in the interference fringes fromed near the point $P$ (shown in the figure) (c) if the intenstities at point $P$ corresponds to a maximum,calculate the minimum distance through which the reflecting surface $A B$ should be shifted so that the intensity at $P$ again becomes maximum.
Acircle
Bsquare
Cparbola
Dstraight line
A point is emitting light of wavelength $6000 Å$ is placed at a very small height $h$ above a flat reflecting surface $M N$ as shown in the figure.The intensity of the reflected light is $36 %$ of the incident intensity.Interference fringes are observed on a screen placed parallel to the reflecting surface at a very large distance $D$ from it.

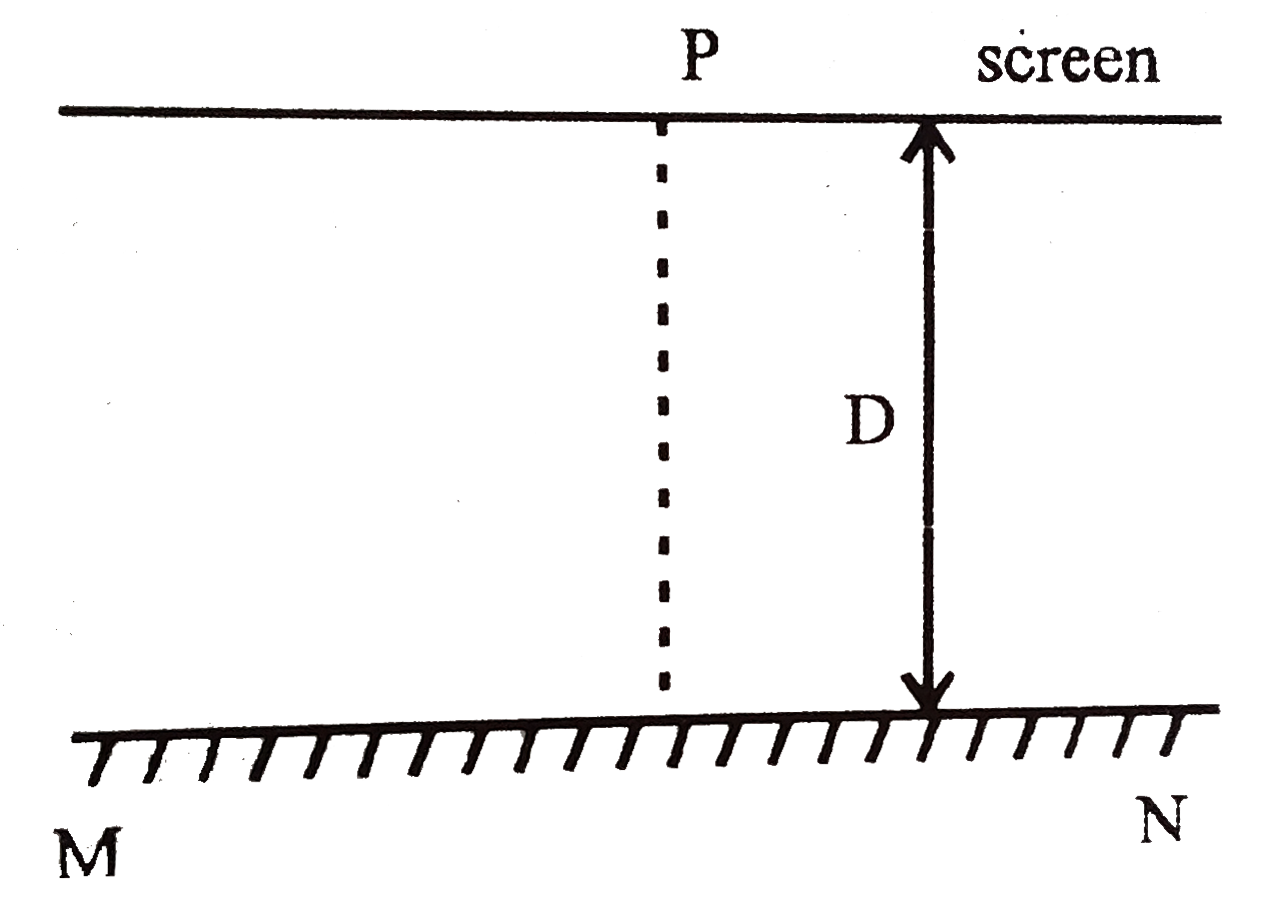
If the intensity at pbe maximum then the minimum distance through which the reflecting suface $M N$ should be displaced so that at $P$ again becomes a mixture.?
A $3 \times 10^{- 10} m$
B $6 \times 10^{- 10} m$
C $1.5 \times 10^{- 10} m$
D $12 \times 10^{- 10} m$
A point source S emits light of wavelength 600 nm. It is palced at a very small distance from a flat reflecting mirror AB. Interface. Frignes as observed on the screen placed parallel to reflecting surface at very large distance D from it. The ratio of minimum to maximum intensities in the interference frignes formed near the point P is 1/6.
Q. If the intensity of P correspoonds to a maximum, calculate the minimum distance through which the reflecting surface AB should so that intensity at P again becomes maximum.

A150 nm
B300 nm
C450 nm
D575 nm